Google Earth Voyager Story: The Geometry of Castles

During Medieval times, castles were fortresses, mini-cities where the royalty lived, and symbols of power. Most castles were tall, imposing structures. But why were they so tall? Was it just for symbolic reasons, to allow a king to show his center of power and influence? In this exploration, we will explore the geometry of castles in order to better understand why they were built the way they were. To see the Google Earth version of this lesson go to this link (best viewed in Chrome).

To see the complete collection of Google Earth Voyager Stories from Media4Math, go to this link.

1. Introduction: Angles and Line of Sight

To start this lesson, watch this video about Himeji Castle and role of line of sight.

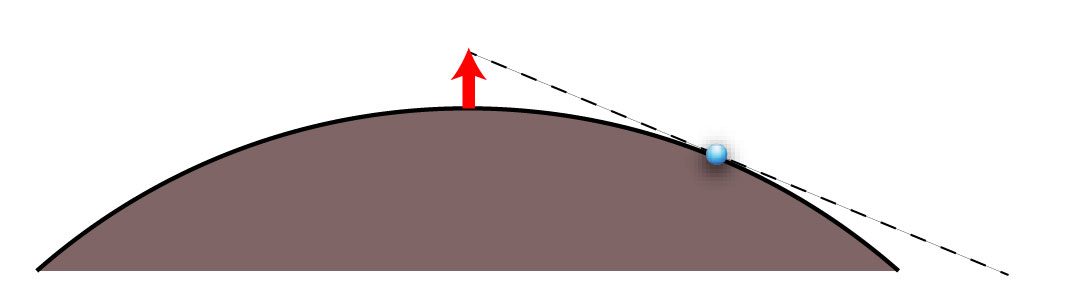
Many castles had a circular watch tower that gave a wide view of the surrounding countryside. These towers provided a clear line of sight to enemy armies approaching. And the taller the tower the better.

In the diagram below the red line with an arrow tip represents a watch tower. The dashed line represents the line of sight. Since the Earth is circular, the line of sight extends to the point where the line of sight intersects the circular shape. This point is where the line is tangent to the circle. The distance from the watch tower height to the point of tangency is the maximum line of sight possible.

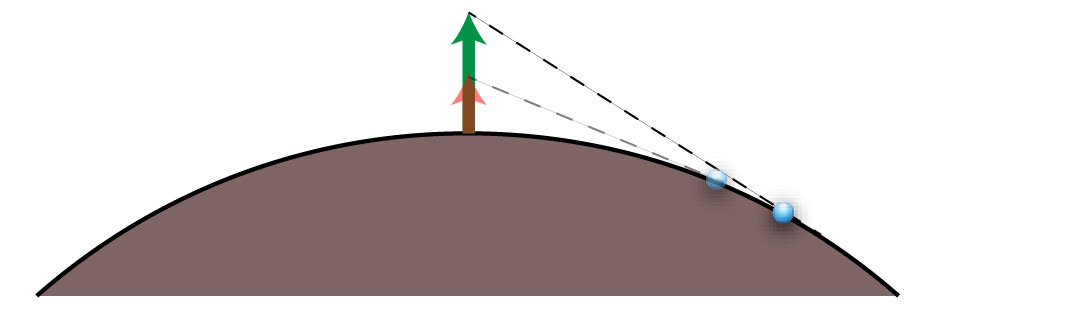
But watch what happens when the height of the tower is increased. In this diagram, the green line is a taller tower. Note how much farther away the point of tangency is than in the previous example. A taller tower means a much greater line of sight.

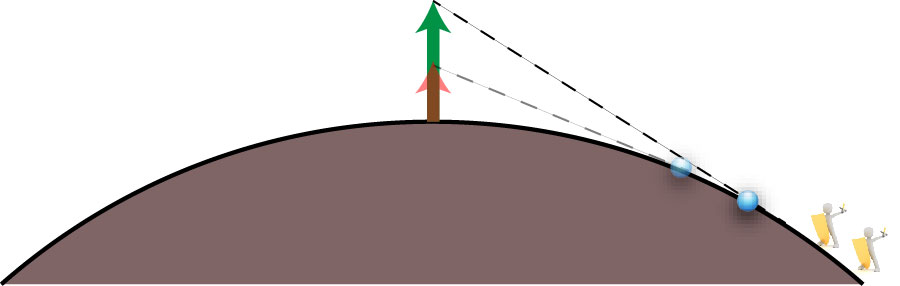
What is the advantage of a greater line of sight? Soldiers on a watch tower can see an approaching enemy army as soon as it's in the line of sight. The taller the watch tower, the farther away the army can be spotted. The farther away an enemy army is, the more time the castle military has to prepare its defenses for an impending attack.

Now that you know how important the line of sight is, how can you calculate the actual distance of the line of sight? There are several strategies available, from using the Pythagorean Theorem to calculating trig ratios.

Now that you've seen the relationship between line of sight and tangents to circles, think of how a satellite takes advantage of these geometric features to send and receive signals to radar towers on Earth.

2. Calculating Line of Sight Distance

Watch the video to learn how to calculate the line of sight distance. This video uses the TI-Nspire graphing calculator, which you can use to follow along.

Notice that this video relies on the Pythagorean theorem to calculate the line of sight distance. Can you think of other ways this distance could be calculated?