 Lesson Plan: Subtracting Fractions with Unlike Denominators
Lesson Plan: Subtracting Fractions with Unlike Denominators
Lesson Summary
This 50-minute lesson focuses on subtracting fractions with unlike denominators and solving related word problems. Students will learn the process of finding common denominators and subtracting fractions step-by-step. The lesson incorporates various multimedia resources from Media4Math.com, including visual aids and interactive activities. A 10-question quiz with an answer key is provided at the end to assess student understanding of the concepts covered.
Lesson Objectives
- Subtract fractions with unlike denominators
- Solve word problems involving subtraction of fractions with unlike denominators
Common Core Standards
- CCSS.Math.Content.5.NF.A.1: Add and subtract fractions with unlike denominators (including mixed numbers) by replacing given fractions with equivalent fractions in such a way as to produce an equivalent sum or difference of fractions with like denominators.
Prerequisite Skills
- Adding fractions with unlike denominators
- Subtracting fractions with like denominators
Key Vocabulary
- Unlike denominators: Fractions with different bottom numbers
- Difference: The result of subtracting one number or fraction from another
- Common denominator: A common multiple of the denominators of two or more fractions
- Least common denominator (LCD): The smallest common denominator for a set of fractions
Multimedia Resources
- A collection of fraction definitions: https://www.media4math.com/Definitions--Fractions
- A collection of video definitions on the topic of fractions: https://www.media4math.com/MathVideoCollection--FractionsVocabulary
- A slide show of definitions on the topic of fractions: https://www.media4math.com/library/slideshow/fraction-definitions
Warm Up Activities
Choose from one or more of these activities:
Calculator Activity
Use any fraction calculator for this activity. Desmos.com has a freely available calculator.
- Students use calculators to convert fractions to decimals and estimate differences of fractions with unlike denominators.
- For example, estimate 34 - 13 by converting to decimals (0.75 - 0.33 ≈ 0.42).
Fraction Bars Activity
- Display images of fraction subtraction with unlike denominators using fraction bars.
- Ask students to describe what they observe and how the visual representations help them understand the concept.
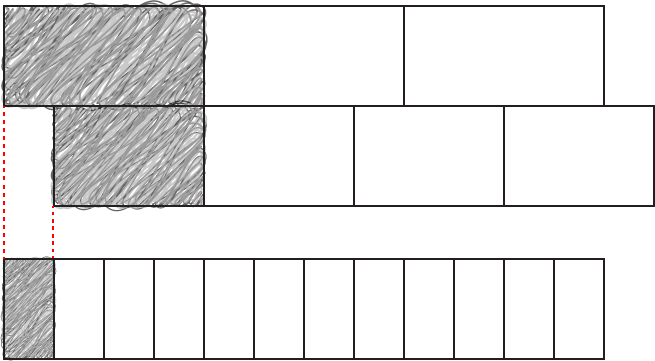
13 - 14 = 112
Number Line Activity
- Provide students with blank number lines from 0 to 1.
- Have them mark fractions like 14, 13, 12, 23, and 34 on the number line.
- Then, ask them to visualize and estimate differences between pairs of fractions, such as 34 - 13 or 23 - 14, using the number line.
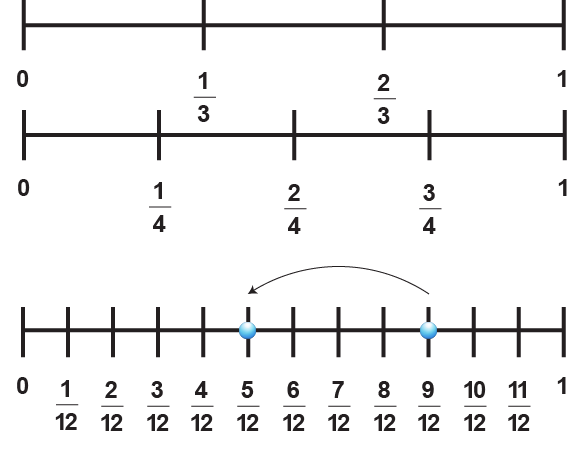
34 - 13 = 512
Teach
Subtracting fractions with unlike denominators requires finding a common denominator before performing the subtraction. This process ensures that we are working with equivalent fractions that represent the same parts of a whole.
Steps for subtracting fractions with unlike denominators:
- Find the least common denominator (LCD) of the fractions.
- Convert each fraction to an equivalent fraction with the LCD.
- Subtract the numerators while keeping the common denominator.
- Simplify the result if necessary.
Example 1: Subtracting fractions where one denominator is a multiple of the other
Subtract: 38 - 14
- Step 1: The LCD of 8 and 4 is 8.
- Step 2: Convert 14 to an equivalent fraction with denominator 8: 14=28.
- Step 3: Subtract the numerators: 38−28=18.
Therefore, 38−14=18. Notice that in this example one denominator is a multiple of the other.
Example 2: Subtracting simple fractions with unlike denominators using fraction bars
Subtract: 34 - 13 using fraction bars.
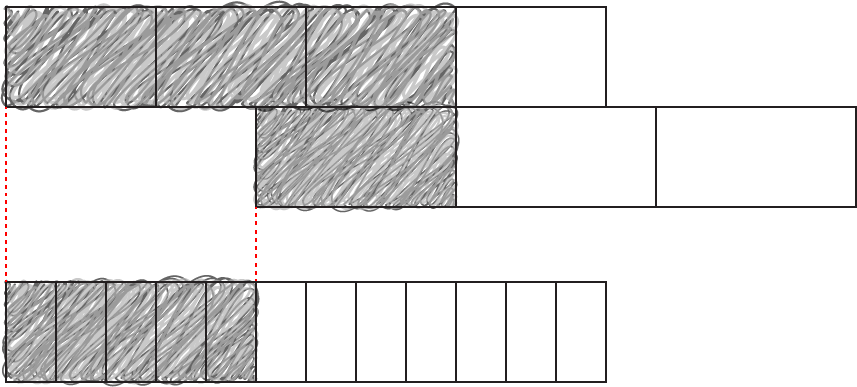
34 - 13 = 512
You can also solve by finding the LCD:
- Step 1: Find the LCD of 4 and 3, which is 12.
- Step 2: Convert each fraction to an equivalent fraction with denominator 12: 34=912, 13=412.
- Step 3: Subtract the numerators: 912−412=512.
- Step 4: The fraction 512 is already in its simplest form.
Therefore, 34−13=512.
Example 3: Subtracting an improper fraction by a proper fraction
Subtract: 74 - 58
- Step 1: Find the LCD of 4 and 8, which is 8.
- Step 2: Convert 74 to an equivalent fraction with denominator 8: 74=148.
- Step 3: Subtract the numerators: 148−58=98.
- Step 4: Convert 98 to a mixed number: 118.
Therefore, 74−58=118.
Example 4: Real-world application
A quilter has 58 yard of fabric and uses 310 yard for a project. How much fabric is left?
- Step 1: Find the LCD of 8 and 10, which is 40.
- Step 2: Convert each fraction to an equivalent fraction with denominator 40: 58=2540, 310=1240.
- Step 3: Subtract the numerators: 2540−1240=1340.
Therefore, the quilter has 1340 yard of fabric left.
Example 5: Real-world application
A baker has 910 cup of sugar and uses 25 cup for a recipe. How much sugar is left?
- Step 1: Find the LCD of 10 and 5, which is 10.
- Step 2: Convert 25 to an equivalent fraction with denominator 10: 25=410.
- Step 3: Subtract the numerators: 910−410=510.
- Step 4: Simplify 510 to 12.
Therefore, the baker has 12 cup of sugar left.
Multimedia Resources
Here are some tutorial videos that provide additional examples:
- Adding Fractions by Using Equivalent Fractions I: https://www.media4math.com/library/39462/asset-preview
- Adding Fractions by Using Equivalent Fractions II: https://www.media4math.com/library/39461/asset-preview
- Adding Mixed Numbers by Using Equivalent Fractions: https://www.media4math.com/library/39460/asset-preview
- Subtracting Fractions Using Equivalent Fractions: https://www.media4math.com/library/39458/asset-preview
- Subtracting Mixed Using Equivalent Fractions: https://www.media4math.com/library/39456/asset-preview
Review
In this lesson, we focused on subtracting fractions with unlike denominators. Key takeaways include:
- To subtract fractions with unlike denominators, always find the least common denominator first.
- Convert fractions to equivalent fractions with the LCD.
- Perform the subtraction and simplify the result if necessary.
Review of Vocabulary:
- Unlike denominators: Fractions with different denominators, requiring adjustment to subtract.
- Least common denominator (LCD): The smallest common multiple of the denominators.
- Equivalent fractions: Fractions that represent the same value, used to align denominators.
Review Example 1: Subtracting fractions with unlike denominators
Subtract: 712 - 16
- Step 1: Find the LCD of 12 and 6, which is 12.
- Step 2: Convert 16 to an equivalent fraction: 16=212.
- Step 3: Subtract the numerators: 712−212=512.
Therefore, 712−16=512.
Review Example 2: Subtracting mixed numbers
Subtract: 214−123
- Step 1: Convert to improper fractions: 214=94, 123=53.
- Step 2: Find the LCD of 4 and 3, which is 12.
- Step 3: Convert to equivalent fractions: 94=2712, 53=2012.
- Step 4: Subtract the numerators: 2712−2012=712.
Therefore, 214−123=712.
Review Example 3: Real-world application
Maria has 56 of a cup of flour. She uses 14 of a cup for a recipe. How much flour does she have left?
- Step 1: Find the LCD of 6 and 4, which is 12.
- Step 2: Convert to equivalent fractions: 56=1012, 14=312.
- Step 3: Subtract the numerators: 1012−312=712.
Therefore, Maria has 712 of a cup of flour left.
Multimedia Resources
- A collection of fraction definitions: https://www.media4math.com/Definitions--Fractions
- A collection of video definitions on the topic of fractions: https://www.media4math.com/MathVideoCollection--FractionsVocabulary
- A slide show of definitions on the topic of fractions: https://www.media4math.com/library/slideshow/fraction-definitions
Quiz
Directions: Solve the following problems involving subtraction of fractions with unlike denominators. Show your work and simplify your answers when possible.
- 56−13
- 34−25
- 78−12
- 23−14
- 45−310
- 1112−23
- 58−316
- 76−512
- 35−215
- 1320−710
Answer Key
- 12
- 720
- 38
- 512
- 12
- 14
- 716
- 14
- 715
- 320