Loading [Contrib]/a11y/accessibility-menu.js

 Lesson Plan: Introduction to Ratios and Unit Rates

 

Lesson Summary

This lesson introduces seventh-grade students to the foundational concepts of ratios and unit rates, emphasizing their applications in real-world scenarios. Students will learn to:

  • Understand Ratios: Comprehend ratios as comparisons between two quantities, expressed in various forms such as a:b, a to b, or a/b.
  • Compute Unit Rates: Calculate unit rates to determine the quantity per one unit of another measure, facilitating easier comparisons and decision-making.
  • Apply Ratios and Unit Rates: Utilize ratios and unit rates to solve practical problems, including those involving speed, pricing, and other rates.

Through engaging activities and examples, students will develop the ability to interpret and solve problems involving ratios and unit rates, enhancing their proportional reasoning skills.

Lesson Objectives

  • Compute unit rates associated with ratios of fractions
  • Apply unit rates to solve real-world problems involving different quantities
  • Use ratio reasoning to convert measurement units
  • Graph linear functions representing unit rates

This lesson plan is designed for one 50-minute class period but could be extended to two periods for more in-depth practice and discussion.

Common Core Standards

  • 7.RP.A.1 Compute unit rates associated with ratios of fractions, including ratios of lengths, areas and other quantities measured in like or different units.

Prerequisite Skills

  • Basic fraction knowledge
  • Understanding of division
  • Graphing linear functions

Key Vocabulary

  • Ratio: A comparison between two quantities, often expressed as a:b, a to b, or a/b. For example, a ratio of 3:2 indicates that for every 3 units of one quantity, there are 2 units of another.
  • Rate: A specific type of ratio that compares two quantities with different units, such as miles per hour or cost per item.
  • Unit Rate: A rate in which the second quantity is one unit. It indicates how many units of the first quantity correspond to one unit of the second quantity. For example, if a car travels 60 miles in 2 hours, the unit rate is 30 miles per hour.
  • Equivalent Ratios: Ratios that express the same relationship between quantities. For instance, the ratios 2:3 and 4:6 are equivalent because they represent the same proportional relationship.
  • Conversion Factor: A ratio used to convert one unit of measurement to another. For example, to convert inches to centimeters, the conversion factor is 2.54 cm per inch.
  • Linear Function: A function that produces a straight line when graphed, often represented in the form y = mx + b, where m is the slope and b is the y-intercept.
  • Slope: The measure of the steepness or incline of a line, calculated as the change in the y-coordinate divided by the change in the x-coordinate (rise over run). It represents the rate of change between two variables.

Multimedia Resources

 

 

Warm Up Activities

Choose one or more activities.

Activity 1: Brief Review of Ratios

Objective: Reinforce students' understanding of ratios and how they compare two quantities.

Instructions:

  1. Write several ratio scenarios on the board, such as:
    • In a class of 30 students, 12 are wearing glasses. What is the ratio of students with glasses to those without?
    • A fruit basket contains 5 apples and 8 oranges. What is the ratio of apples to oranges?
  2. Have students express each ratio in three different ways: fraction form (5/8), colon form (5:8), and word form ("5 to 8").
  3. Ask students: "Are these ratios part-to-part or part-to-whole?" and discuss their reasoning.

Activity 2: Brief Review of Equivalent Ratios

Objective: Help students recognize and generate equivalent ratios.

Instructions:

  1. Write a base ratio on the board, such as 2:3.
  2. Ask students: "How can we create an equivalent ratio?" Guide them to multiply or divide both numbers by the same factor.
  3. Demonstrate:
    • \( 2:3 = 4:6 \) (multiply both by 2)
    • \( 2:3 = 6:9 \) (multiply both by 3)
  4. Provide students with a list of incomplete equivalent ratios and have them fill in the missing values.

Activity 3: Brief Review of Rates

Objective: Introduce students to rates and how they differ from ratios.

Instructions:

  1. Write a scenario on the board: "A car travels 120 miles in 2 hours."
  2. Ask: "How can we express this as a rate?" Guide students to write it as \( 120 \text{ miles} \div 2 \text{ hours} = 60 \text{ miles per hour} \).
  3. Present additional rate scenarios:
    • A grocery store sells 6 apples for $3. What is the cost per apple?
    • A runner completes 4 laps in 10 minutes. What is the lap rate per minute?
  4. Have students calculate the unit rate for each scenario and discuss why unit rates are useful.

Activity 4: Speed As a Rate

Present students with the following scenario:

A car travels 180 miles in 3 hours.

Ask students to:

  • Calculate the speed of the car in miles per hour
  • Determine how far the car would travel in 5 hours at this rate
  • Convert the speed to kilometers per hour (use 1 mile ≈ 1.60934 km)

Discuss how these calculations relate to unit rates and conversions between different units.

 

 

Teach

Definitions

  • Ratio: A comparison between two quantities, often expressed as a:b, a to b, or a/b.
  • Unit rate: A ratio where the second term is 1, used to compare quantities per unit.
  • Equivalent ratios: Ratios that represent the same relationship between quantities.
  • Conversion factor: A ratio used to convert one unit of measurement to another.
  • Linear function: A function that produces a straight line when graphed, often in the form y = mx + b.
  • Slope: The steepness of a line, calculated as the change in y divided by the change in x.

Use this slide show to review these and related definitions that are used in this lesson:

https://www.media4math.com/library/slideshow/ratio-and-rate-definitions

Instruction

Use this slide show to introduce rates and unit rates:

https://www.media4math.com/library/slideshow/introduction-rates

As time allows show the following examples of ratios and rates.

Example 1: Calculating a Ratio

Problem: A class has 12 boys and 18 girls. What is the ratio of boys to girls? What is the ratio of boys to total students?

Solution:

  1. Write the ratio of boys to girls: \[ \frac{12}{18} = 12:18 = 12 \text{ to } 18 \]
  2. Simplify the ratio by dividing both numbers by their greatest common factor (GCF = 6): \[ \frac{12 \div 6}{18 \div 6} = \frac{2}{3} = 2:3 \]
  3. Find the ratio of boys to total students: \[ \frac{12}{12 + 18} = \frac{12}{30} \]
  4. Simplify: \[ \frac{12 \div 6}{30 \div 6} = \frac{2}{5} = 2:5 \]
  5. Final Answer: The ratio of boys to girls is 2:3, and the ratio of boys to total students is 2:5.

Example 2: Using Equivalent Ratios

Problem: A recipe calls for 4 cups of flour and 6 cups of sugar. If a baker wants to make a larger batch using 10 cups of flour, how much sugar should they use?

Solution:

  1. Write the given ratio: \[ \frac{4}{6} \]
  2. Find the scale factor: \[ 10 \div 4 = 2.5 \]
  3. Multiply the sugar by the same factor: \[ 6 \times 2.5 = 15 \]
  4. Final Answer: The baker should use 15 cups of sugar.

Example 3: Solving a Proportion Using Equivalent Ratios

Problem: A car travels 150 miles in 3 hours. At the same speed, how far will it travel in 5 hours?

Solution:

  1. Write the given ratio: \[ \frac{150}{3} \]
  2. Find the equivalent ratio for 5 hours: \[ \frac{x}{5} \]
  3. Find the scale factor: \[ 5 \div 3 = \frac{5}{3} \]
  4. Multiply the miles by the same factor: \[ 150 \times \frac{5}{3} = 250 \]
  5. Final Answer: The car will travel 250 miles in 5 hours.

Example 4: Physics - Speed Calculation (Finding the Unit Rate)

A cyclist travels 36 miles in 2.4 hours.

Question: What is the cyclist's speed in miles per hour?

Solution:

Unit rate (speed) = Distance ÷ Time
                      = 36 miles ÷ 2.4 hours
                      = 15 miles per hour

The cyclist's speed is 15 miles per hour.

Example 5: Business - Production Planning (Calculating and Using Unit Rate)

A factory produces 540 widgets in a 12-hour shift.

Questions:

  1. What is the production rate in widgets per hour?
  2. How many widgets can the factory produce in an 8-hour shift at this rate?

Solution:

a) Unit rate = Total widgets ÷ Total hours
                 = 540 widgets ÷ 12 hours
                 = 45 widgets per hour
b) Production in 8 hours = 45 widgets/hour × 8 hours = 360 widgets

The factory produces 45 widgets per hour and can produce 360 widgets in an 8-hour shift.

Example 6: Engineering - Fuel Efficiency (Graphing and Calculating Unit Rate)

A car's fuel consumption can be represented by the following data points. For each gallon of gas used, the car travels a certain number of miles.

GallonsMiles
260
5150
8240

Questions:

  1. Graph these points on a coordinate plane with gallons on the x-axis and miles on the y-axis.
  2. Draw a line through these points.
  3. Calculate the slope of this line.
  4. What does the slope represent, and what are its units?

Solution:

 

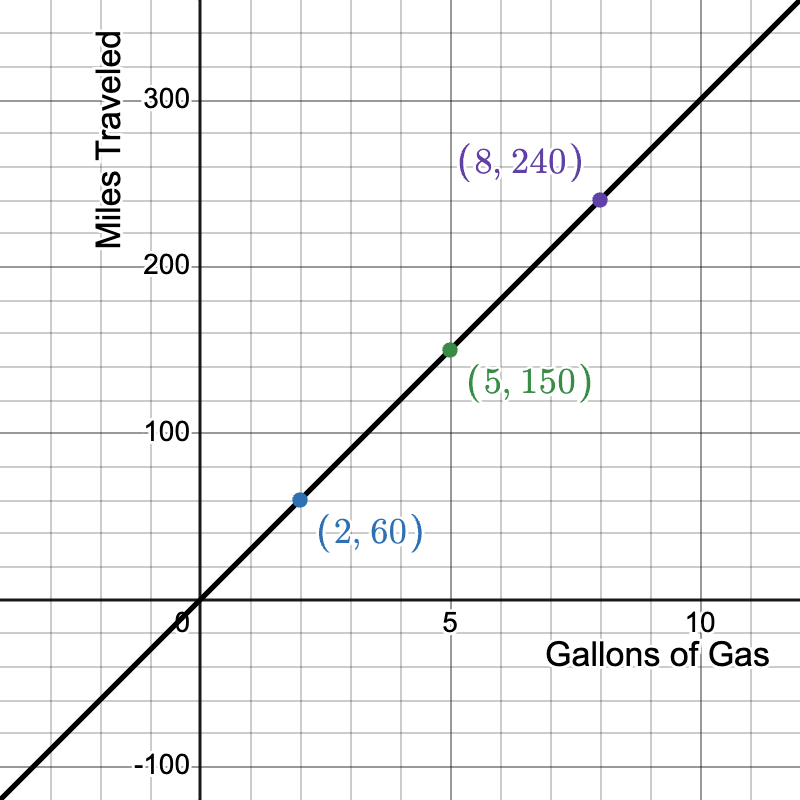
Coordinate Graph

 

 

Slope = Change in y ÷ Change in x
             = (240 - 60) ÷ (8 - 2)
             = 180 ÷ 6
             = 30
The slope represents the fuel efficiency of the car. Its units are miles per gallon (mpg).

The car's fuel efficiency is 30 miles per gallon.

 

 

Review

Lesson Summary

In this lesson, students explored the foundational concepts of ratios and unit rates. They learned how to:

  • Define and express ratios: Represent ratios in three different ways: fraction form, colon form, and word form.
  • Generate equivalent ratios: Use scaling to create equivalent ratios that maintain the same relationship.
  • Understand unit rates: Calculate unit rates to compare different quantities and simplify decision-making.
  • Solve proportions using equivalent ratios: Apply proportional reasoning to find missing values in real-world situations.

By practicing these skills, students developed problem-solving strategies that apply to everyday situations, such as comparing prices, calculating speed, and adjusting recipe measurements.

Key Vocabulary

  • Ratio: A comparison of two quantities, expressed as a fraction, with a colon, or in words (e.g., 3:4, 3/4, "3 to 4").
  • Rate: A ratio that compares two quantities with different units (e.g., miles per hour, cost per pound).
  • Unit Rate: A rate in which the second quantity is 1, allowing easy comparison between different values (e.g., "miles per gallon").
  • Proportion: An equation that shows two ratios are equal, often used to solve for unknown values.
  • Scaling: Multiplying or dividing both terms of a ratio by the same factor to create an equivalent ratio.

Multimedia Resources

Use this video to review unit rates:

https://www.media4math.com/library/1797/asset-preview

 If time allows, work through another detailed example with the class:

A coffee shop sells 180 cups of coffee in 3 hours.

Questions:

  1. What is the unit rate of coffee sales per hour?
  2. How many cups of coffee can they expect to sell in an 8-hour day at this rate?
  3. Graph the relationship between hours and cups of coffee sold from 0 to 10 hours.
  4. Using the graph, estimate how long it would take to sell 300 cups of coffee.

Guide students through each step, encouraging participation and discussion. If necessary, refer to these grade 6 lessons on ratios for additional review:

Additional Worked-Out Examples: Real-World Unit Rate Problems

Example 1: Grocery Store Pricing

Problem: A grocery store sells a 12-pack of soda for $9. How much does each can cost?

Solution:

  1. Set up the rate: \[ \frac{9 \text{ dollars}}{12 \text{ cans}} \]
  2. Find the unit rate by dividing: \[ \frac{9}{12} = 0.75 \]
  3. Final Answer: Each can costs $0.75.

Example 2: Fuel Efficiency

Problem: A car travels 240 miles on 8 gallons of gas. What is the fuel efficiency in miles per gallon?

Solution:

  1. Set up the rate: \[ \frac{240 \text{ miles}}{8 \text{ gallons}} \]
  2. Find the unit rate by dividing: \[ \frac{240}{8} = 30 \]
  3. Final Answer: The car gets 30 miles per gallon.

Example 3: Hourly Wage

Problem: A part-time worker earns $72 after working 6 hours. What is the worker’s hourly wage?

Solution:

  1. Set up the rate: \[ \frac{72 \text{ dollars}}{6 \text{ hours}} \]
  2. Find the unit rate by dividing: \[ \frac{72}{6} = 12 \]
  3. Final Answer: The worker earns $12 per hour.

Example 4: Running Speed

Problem: A runner completes a 5-kilometer race in 25 minutes. What is the runner’s speed in kilometers per minute?

Solution:

  1. Set up the rate: \[ \frac{5 \text{ km}}{25 \text{ min}} \]
  2. Find the unit rate by dividing: \[ \frac{5}{25} = 0.2 \]
  3. Final Answer: The runner’s speed is 0.2 kilometers per minute.

 

 

Quiz

Answer the following questions.

 

  1. If a car travels 240 miles in 4 hours, what is its speed in miles per hour?

     
  2. Convert 65 miles per hour to kilometers per hour. (1 mile ≈ 1.60934 km)

     
  3. A recipe calls for 2/3 cup of flour for every 1/4 cup of sugar. How much flour is needed for 1 cup of sugar?

     
  4. If 18 widgets are produced in 1.5 hours, what is the production rate in widgets per hour?

     
  5. A delivery truck uses 12 gallons of gas to travel 180 miles. What is its fuel efficiency in miles per gallon?

     
  6. Convert 30 miles per gallon to kilometers per liter. (1 gallon ≈ 3.78541 liters, 1 mile ≈ 1.60934 km)

     
  7. If 5/8 kg of rice costs \$2.40, what is the price per kg?

     
  8. A printer prints 80 pages in 2 minutes. How many pages can it print in 7 minutes?

     
  9. If 3/4 cup of flour weighs 90 grams, how many grams does 1 cup of flour weigh?

     
  10. A train travels 450 km in 3 hours. What is its speed in meters per second?

Answer Key

  1. 60 mph
  2. 104.6 km/h
  3. 2 2/3 cups of flour
  4. 12 widgets per hour
  5. 15 mpg
  6. 12.75 km/L
  7. \$3.84 per kg
  8. 280 pages
  9. 120 grams
  10. 41.67 m/s