Illustrative Math Alignment: Grade 8 Unit 2

Overview

Overview

The Transformations collection on Media4Math offers a comprehensive set of 56 math examples, providing educators with a rich resource for teaching geometric transformations. This collection covers a wide range of skills and concepts related to transformations, including translations, reflections, rotations, and dilations.

Topic

Transformations

Topic

Transformations

Description

Explores translations and rotations in motion, using roller coasters as an example. Key concepts include displacement vectors, magnitude, and direction, modeled geometrically and computationally for practical applications in physics and engineering.

In this video, you will explore the fascinating applications of transformations in real-world scenarios. These mathematical concepts are central to understanding geometric principles and their practical applications in various fields.

Topic

Transformations

Description

This segment applies 3D translations to logistics, focusing on container arrangement in shipping. Using coordinate systems, it demonstrates efficiency in loading/unloading cargo, leveraging geometric organization and data sorting methods for real-world impact.

In this video, you will explore the fascinating applications of transformations in real-world scenarios. These mathematical concepts are central to understanding geometric principles and their practical applications in various fields.

Topic

Transformations

Description

Analyzes observatory designs and their use of rotational and reflective symmetry for astronomical viewing. The segment models celestial movements and applies rotational geometry concepts to optimize the structure’s functionality.

In this video, you will explore the fascinating applications of transformations in real-world scenarios. These mathematical concepts are central to understanding geometric principles and their practical applications in various fields.

This hands-on activity is a companion to the video segment from the Geometry Applications: Angles and Planes video. In particular, it supports the segment on the architecture of Himeji Castle.

Library of Instructional Resources

To see the complete library of Instructional Resources , click on this link.

This hands-on activity is a companion to the video segment from the Geometry Applications: Angles and Planes video. In particular, it supports the segment on the architecture of Himeji Castle.

Library of Instructional Resources

To see the complete library of Instructional Resources , click on this link.

This hands-on activity is a companion to the video segment from the Geometry Applications: Angles and Planes video. In particular, it supports the segment on the architecture of Himeji Castle.

Library of Instructional Resources

To see the complete library of Instructional Resources , click on this link.

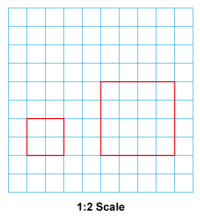
Topic

Geometry Concepts

Description

This image depicts a scale drawing of a square that has been scaled up by a factor of 1:2. This means that each side of the square in the drawing is twice the length of the corresponding side in the actual object. Scale drawings are essential for accurately representing objects in a manageable size while maintaining proportionality.

Teacher's Script: "Observe this scale drawing of a square. How does the 1:2 scale factor help us understand the relationship between the drawing and the actual square? What are some real-world applications of scaling up objects, such as in architecture or design?"

Topic

Geometry Concepts

Description

This image illustrates a scale drawing of a square that has been scaled up by a factor of 1:3. Each side of the square in the drawing is three times the length of the corresponding side in the actual object. This scaling helps in visualizing larger versions of objects while maintaining accurate proportions.

Teacher's Script: "Look at this scale drawing of a square. How does the 1:3 scale factor affect the dimensions of the drawing compared to the actual square? What are some mathematical concepts you can explore using scale drawings, such as ratios or proportions?"

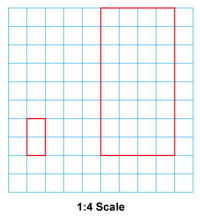
Topic

Geometry Concepts

Description

This image shows a scale drawing of a rectangle that has been scaled up by a factor of 1:4. Each side of the rectangle in the drawing is four times the length of the corresponding side in the actual object. This scaling is useful for enlarging objects while maintaining their proportional dimensions.

Teacher's Script: "Examine this scale drawing of a rectangle. How does the 1:4 scale factor influence the size of the drawing compared to the actual rectangle? What are some real-world applications of scaling up objects, such as in engineering or construction?"

Topic

Geometry Concepts

Description

This image illustrates a scale drawing of a rectangle that has been scaled up by a factor of 1:5. Each side of the rectangle in the drawing is five times the length of the corresponding side in the actual object. Scale drawings like this are crucial for visualizing larger versions of objects while maintaining accurate proportions.

Teacher's Script: "Observe this scale drawing of a rectangle. How does the 1:5 scale factor affect the dimensions of the drawing compared to the actual rectangle? What are some mathematical concepts you can explore using scale drawings, such as ratios or proportions?"

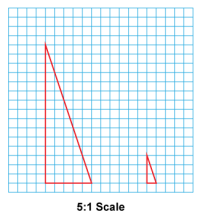
Topic

Geometry Concepts

Description

This image depicts a scale drawing of a right triangle that has been scaled down by a factor of 5:1. This means each side of the triangle in the drawing is one-fifth the length of the corresponding side in the actual object. Scaling down is useful for creating manageable representations of larger objects.

Teacher's Script: "Examine this scale drawing of a right triangle. How does the 5:1 scale factor help us understand the relationship between the drawing and the actual triangle? What are some real-world applications of scaling down objects, such as in model making or map creation?"

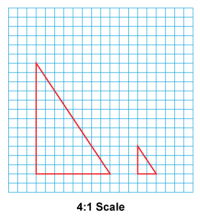
Topic

Geometry Concepts

Description

This image illustrates a scale drawing of a right triangle that has been scaled down by a factor of 4:1. Each side of the triangle in the drawing is one-fourth the length of the corresponding side in the actual object. This scaling helps in visualizing smaller versions of objects while maintaining accurate proportions.

Teacher's Script: "Observe this scale drawing of a right triangle. How does the 4:1 scale factor affect the dimensions of the drawing compared to the actual triangle? What are some mathematical concepts you can explore using scale drawings, such as ratios or proportions?"

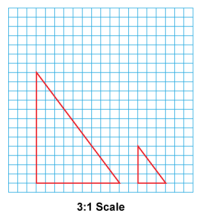
Topic

Geometry Concepts

Description

This image depicts a scale drawing of a right triangle that has been scaled down by a factor of 3:1. Each side of the triangle in the drawing is one-third the length of the corresponding side in the actual object. Scaling down is useful for creating manageable representations of larger objects.

Teacher's Script: "Examine this scale drawing of a right triangle. How does the 3:1 scale factor help us understand the relationship between the drawing and the actual triangle? What are some real-world applications of scaling down objects, such as in model making or map creation?"

Topic

Geometry Concepts

Description

This image illustrates a scale drawing of a right triangle that has been scaled down by a factor of 2:1. Each side of the triangle in the drawing is one-half the length of the corresponding side in the actual object. This scaling helps in visualizing smaller versions of objects while maintaining accurate proportions.

Teacher's Script: "Observe this scale drawing of a right triangle. How does the 2:1 scale factor affect the dimensions of the drawing compared to the actual triangle? What are some mathematical concepts you can explore using scale drawings, such as ratios or proportions?"

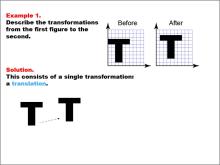
Topic

Transformations

Description

This example demonstrates a simple translation in geometric transformations. Two "T" shapes are shown on a grid, labeled "Before" and "After". The "Before" shape on the left is translated to become the "After" shape on the right, illustrating how an object can move position without changing its size or orientation.

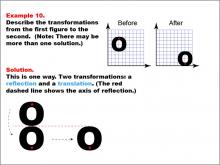
Topic

Transformations

Description

This example showcases a combination of reflection and translation in geometric transformations. Two circular "O" shapes are displayed on a grid, one labeled "Before" and the other "After". A red dashed line indicates the axis of reflection. The transformation involves first reflecting the shape across this axis and then translating it to a new position. This demonstrates how multiple transformations can be applied in sequence to create more complex movements.

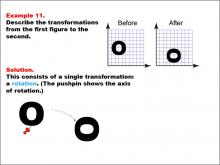
Topic

Transformations

Description

This example demonstrates a single rotation in geometric transformations. Two circular "O" shapes are shown on a grid, one labeled "Before" and the other "After". The shape undergoes a rotation around a point indicated by a pushpin, which serves as the axis of rotation. This illustrates how an object can change its orientation without altering its size or position relative to the rotation point.

Topic

Transformations

Description

This example illustrates a combination of rotation and reflection in geometric transformations. Two circular "O" shapes are displayed on a grid, one labeled "Before" and the other "After". The transformation involves two steps: first, a rotation around a point indicated by a pushpin, which serves as the axis of rotation, followed by a reflection across a red dashed line representing the axis of reflection. This demonstrates how multiple transformations can be applied sequentially to create more complex changes in orientation and position.

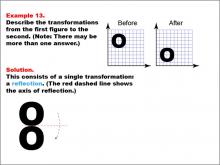
Topic

Transformations

Description

This example demonstrates a single reflection in geometric transformations. Two grids are shown with the letter "O" before and after a transformation. The "Before" grid has the "O" on the left side, while the "After" grid has it on the right side. A red dashed line indicates the axis of reflection in the middle of the two "O"s. Below, there is a diagram showing the reflection transformation along this axis, illustrating how the shape is flipped across the line while maintaining its size and shape.

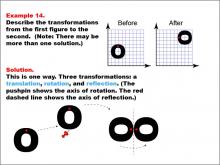
Topic

Transformations

Description

This example illustrates a complex combination of transformations: translation, rotation, and reflection. Two grids are shown with an "O" shape before and after transformations. The "Before" grid has an "O" with a red dot at its center, while the "After" grid shows the same "O" but rotated and reflected. Below, a step-by-step diagram illustrates three sequential transformations: first, a translation moves the shape, then a rotation turns it around a pushpin (indicating the axis of rotation), and finally, a reflection flips it across a red dashed line.

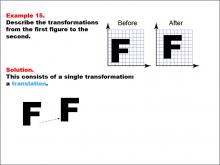
Topic

Transformations

Description

This example demonstrates a simple translation in geometric transformations. Two grids are displayed with the letter "F" before and after a transformation. The "Before" grid shows an "F" on the left side, and the "After" grid shows it on the right side. Below, there is an arrow indicating that only a translation occurred between the two positions of the letter, showcasing how an object can move position without changing its size or orientation.

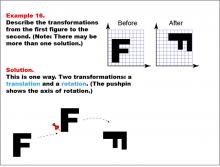
Topic

Transformations

Description

This example demonstrates a combination of translation and rotation in geometric transformations. Two grids are shown with the letter "F" before and after transformations. The "Before" grid has an upright "F", while the "After" grid shows it rotated 90 degrees clockwise. Below, there is a diagram illustrating two steps: first, a translation moves the shape, then a rotation turns it around a pushpin axis.

Topic

Transformations

Description

This example illustrates a combination of reflection and translation in geometric transformations. The image shows a large letter "F" on a grid transforming into a letter "L". The transformation involves two steps: first, a reflection across an axis represented by a red dashed line, and then a translation to a new position.

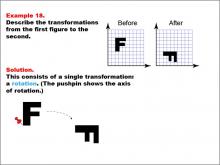
Topic

Transformations

Description

This example demonstrates a single rotation in geometric transformations. The image shows the letter "F" on a grid being rotated to form the same letter in a different orientation. A pushpin indicates the axis of rotation, and an arrow shows the direction of rotation, illustrating how an object can change its orientation without altering its size or shape.

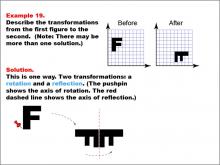
Topic

Transformations

Description

This example illustrates a combination of rotation and reflection in geometric transformations. The image shows the letter "F" being transformed into an upside-down version of itself. This transformation involves two steps: first, a rotation around an axis indicated by a pushpin, followed by a reflection across a line represented by a red dashed line.

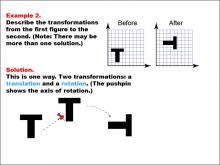
Topic

Transformations

Description

This example illustrates a combination of transformations: translation and rotation. Two "T" shapes are shown on a grid, labeled "Before" and "After". The shape undergoes a translation (movement) and a rotation, with a pushpin indicating the axis of rotation. This demonstrates how multiple transformations can be applied sequentially to a shape.

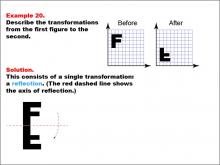
Topic

Transformations

Description

This example demonstrates a single reflection in geometric transformations. The image shows the letter "F" being reflected to form the letter "L". A red dashed line indicates the axis of reflection, illustrating how the letter changes its orientation through this single transformation. This showcases how an object can be flipped over a line while changing its shape and orientation.

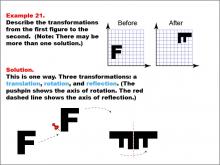
Topic

Transformations

Description

This example demonstrates a complex combination of transformations: translation, rotation, and reflection. The image shows an uppercase letter "F" on a grid labeled "Before" and a transformed version of the letter "F" upside down on another grid labeled "After." Below, there are illustrations showing the three steps of the transformation process.

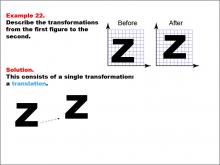
Topic

Transformations

Description

This example demonstrates a simple translation in geometric transformations. The image shows an uppercase letter "Z" on a grid labeled "Before" and a horizontally shifted version of the same letter on another grid labeled "After." Below, there is an illustration showing just a translation, indicating that the letter "Z" is moved horizontally without any rotation or reflection.

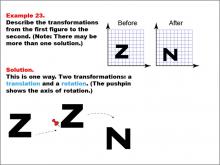
Topic

Transformations

Description

This example illustrates a combination of translation and rotation in geometric transformations. The image shows an uppercase letter "Z" on a grid labeled "Before" and an uppercase letter "N" on another grid labeled "After." Below, there are illustrations showing the two steps of the transformation: first a translation (moving the figure) followed by a rotation (turning the figure). A pushpin indicates the axis of rotation.

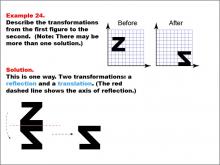
Topic

Transformations

Description

This example demonstrates a combination of reflection and translation in geometric transformations. The image shows an uppercase letter "Z" on a grid labeled "Before" and a rotated and translated version of the same letter in another position on another grid labeled "After." Below, there are illustrations showing the two steps of the transformation: first, a reflection (flipping the figure across an axis shown by the red dashed line) and then a translation (moving the figure).

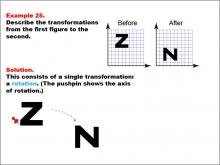
Topic

Transformations

Description

This example demonstrates a single rotation in geometric transformations. The image shows a transformation of the letter "Z" from an upright position to a rotated position resembling "N". The grid background helps visualize the transformation, and a pushpin icon indicates the axis of rotation, illustrating how an object can change its orientation without altering its size or shape.

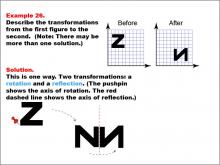
Topic

Transformations

Description

This example illustrates a combination of rotation and reflection in geometric transformations. The image shows a transformation of the letter "Z" into an upside-down "N". A pushpin indicates the axis of rotation, and a red dashed line represents the axis of reflection. This demonstrates how multiple transformations can be applied sequentially to create more complex changes in orientation and position.

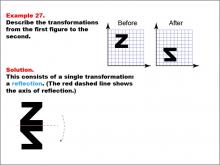
Topic

Transformations

Description

This example demonstrates a single reflection in geometric transformations. The image shows a reflection of the letter "Z" across a horizontal red dashed line, resulting in a backward "Z". The grid background helps visualize the reflection, illustrating how an object can be flipped over a line while maintaining its size and shape but changing its orientation.

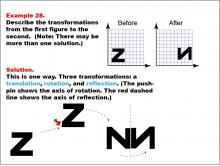
Topic

Transformations

Description

This example demonstrates a complex combination of transformations: translation, rotation, and reflection. The image shows a transformation of the letter "Z" into an upside-down "N". This transformation involves three steps: first, a translation moves the shape, then a rotation turns it (indicated by a pushpin showing the axis of rotation), and finally, a reflection flips it across a red dashed line representing the axis of reflection.

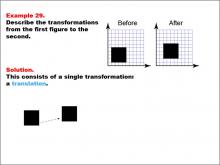
Topic

Transformations

Description

This example demonstrates a simple translation in geometric transformations. The image shows a "Before" and "After" transformation of a black square on a grid. The square moves horizontally to the right, and a dotted arrow indicates the direction of movement. This showcases how an object can move position without changing its size or orientation.

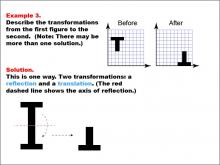
Topic

Transformations

Description

This example showcases a combination of reflection and translation in geometric transformations. Two "T" shapes are displayed on a grid, labeled "Before" and "After". The shape is first reflected across a red dashed line (the axis of reflection) and then translated to a new position. This demonstrates how multiple transformations can be applied in sequence to create more complex movements.

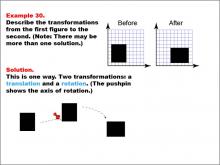
Topic

Transformations

Description

This example illustrates a combination of translation and rotation in geometric transformations. The image depicts a "Before" and "After" transformation of a black square on a grid. The square moves down and rotates 90 degrees clockwise. A pushpin marks the axis of rotation, and dotted arrows indicate the movement and rotation. This demonstrates how multiple transformations can be applied sequentially to create more complex changes in position and orientation.

Topic

Transformations

Description

This example demonstrates a combination of reflection and translation in geometric transformations. The image shows a "Before" and "After" transformation of a black rectangle on a grid. The rectangle first reflects across a horizontal axis, marked by a red dashed line, and then translates horizontally to the right. This illustrates how multiple transformations can be applied sequentially to create more complex changes in position and orientation.

Topic

Transformations

Description

This example demonstrates a single rotation in geometric transformations. The image shows a "Before" and "After" transformation of a black rectangle on a grid. The rectangle rotates 90 degrees clockwise around an axis marked by a pushpin. This illustrates how an object can change its orientation without altering its size or shape.

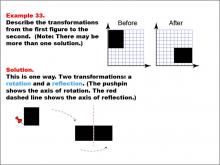
Topic

Transformations

Description

This example illustrates a combination of rotation and reflection in geometric transformations. The image shows a transformation of a black L-shaped figure on a grid. The "Before" figure is at the top left, and the "After" figure is at the top right. Below, there is a sequence of two transformations: first, a rotation (indicated by a pushpin showing the axis of rotation), followed by a reflection (shown by a red dashed line representing the axis of reflection).

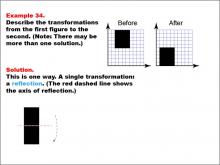
Topic

Transformations

Description

This example demonstrates a single reflection in geometric transformations. The image shows a black L-shaped figure on a grid. The "Before" figure is at the top left, and the "After" figure is at the top right. Below, there is a single transformation: a reflection across a vertical axis shown with a red dashed line. This showcases how an object can be flipped over a line while maintaining its size and shape but changing its orientation.

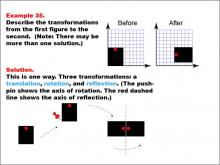
Topic

Transformations

Description

This example demonstrates a complex combination of transformations: translation, rotation, and reflection. The image shows a black L-shaped figure with a red dot on a grid. The "Before" figure is at the top left, and the "After" figure is at the top right. Below, there are three transformations: first, a translation moves the shape, then a rotation turns it around an axis shown by a pushpin, and finally, a reflection flips it across an axis shown by a red dashed line.

Topic

Transformations

Description

This example demonstrates a simple translation in geometric transformations. The image shows two hexagons on grids. The "Before" figure is at the top left, and the "After" figure is at the top right. Below, there is a single transformation: translation, as indicated by an arrow showing movement from one position to another. This showcases how an object can move position without changing its size or orientation.

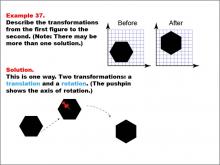
Topic

Transformations

Description

This example illustrates a combination of translation and rotation in geometric transformations. The image shows a hexagon on a grid, with a "Before" and "After" transformation. The pushpin indicates the axis of rotation, and the hexagon is translated and rotated. This demonstrates how multiple transformations can be applied sequentially to create more complex changes in position and orientation.

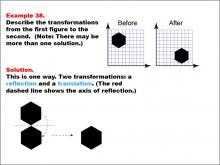
Topic

Transformations

Description

This example demonstrates a combination of reflection and translation in geometric transformations. The image shows a hexagon on a grid, with a "Before" and "After" transformation. A red dashed line shows the axis of reflection, followed by a translation. This illustrates how multiple transformations can be applied sequentially to create more complex changes in position and orientation.

Topic

Transformations

Description

This example demonstrates a single rotation in geometric transformations. The image shows a hexagon on a grid, with a "Before" and "After" transformation. A pushpin indicates the axis of rotation, and only one transformation occurs. This illustrates how an object can change its orientation without altering its size or shape.