Display Title
Definition | 3D Geometry Concepts | Horizontal Cross-Sections of a Triangular Prism
Display Title
Horizontal Cross Sections of a Triangular Prism
Topic
3D Geometry
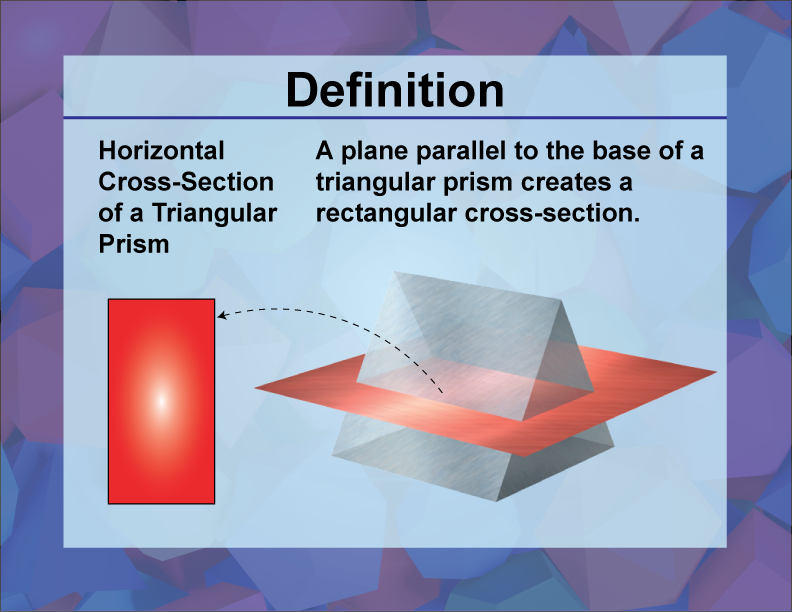
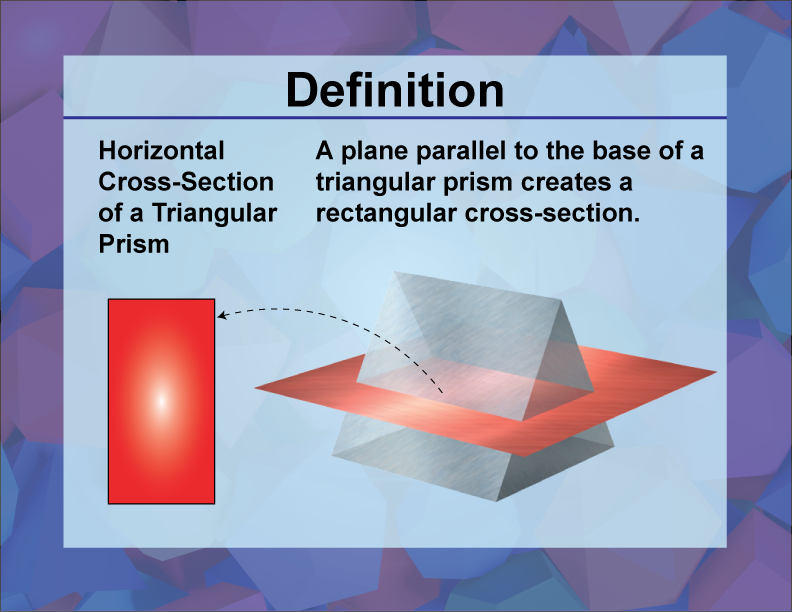
Definition
A horizontal cross-section of a triangular prism is the shape obtained by cutting the prism with a plane parallel to its base.
Description
In three-dimensional geometry, a cross-section is the intersection of a plane with a solid figure. When this plane is parallel to the base of the solid, the resulting shape is called a horizontal cross-section. For a triangular prism with a rectangular base, this cross-section will always be a rectangle, similar in shape to the base of the prism.
Understanding cross-sections is crucial for visualizing and analyzing the internal structures of three-dimensional objects. In the case of a triangular prism, which consists of two parallel triangular bases connected by three rectangular lateral faces, the horizontal cross-sections help in comprehending the uniformity and consistency of the shape along its length.
Horizontal cross-sections are particularly useful in various fields such as engineering, architecture, and computer graphics, where precise modeling of objects is essential. By studying these cross-sections, one can gain insights into the properties and dimensions of the prism, aiding in tasks such as calculating volume, surface area, and material requirements.
For a complete collection of terms related to 3D geometry click on this link: 3D Collection.
| Common Core Standards | CCSS.MATH.CONTENT.5.MD.C.3, CCSS.MATH.CONTENT.7.G.A.3 |
|---|---|
| Grade Range | 4 - 6 |
| Curriculum Nodes |
Geometry • 3D Geometry • Triangular Prisms |
| Copyright Year | 2021 |
| Keywords | three-dimensional geometry, 3d Geometry, defnitions, glossary term |