Display Title
Definition | 3D Geometry Concepts | Vertical Cross-Sections of a Cone
Display Title
Vertical Cross Sections of a Cone
Topic
3D Geometry
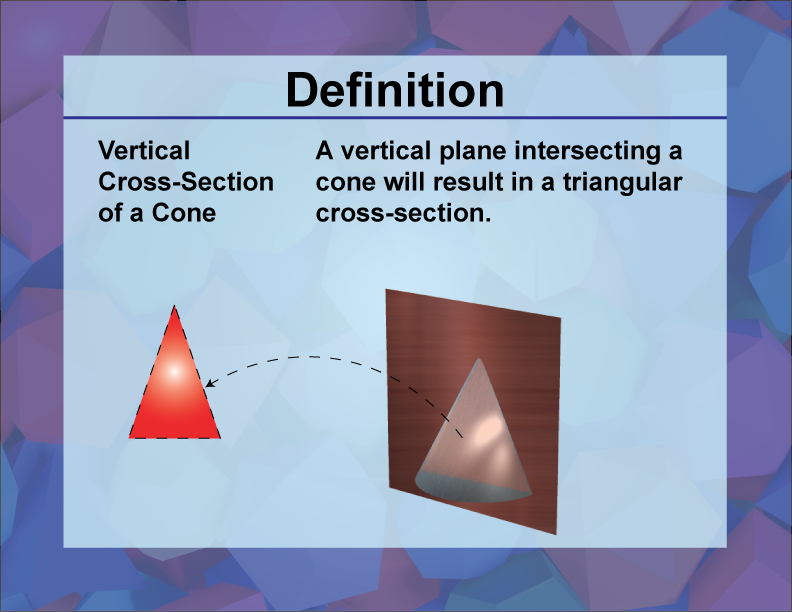
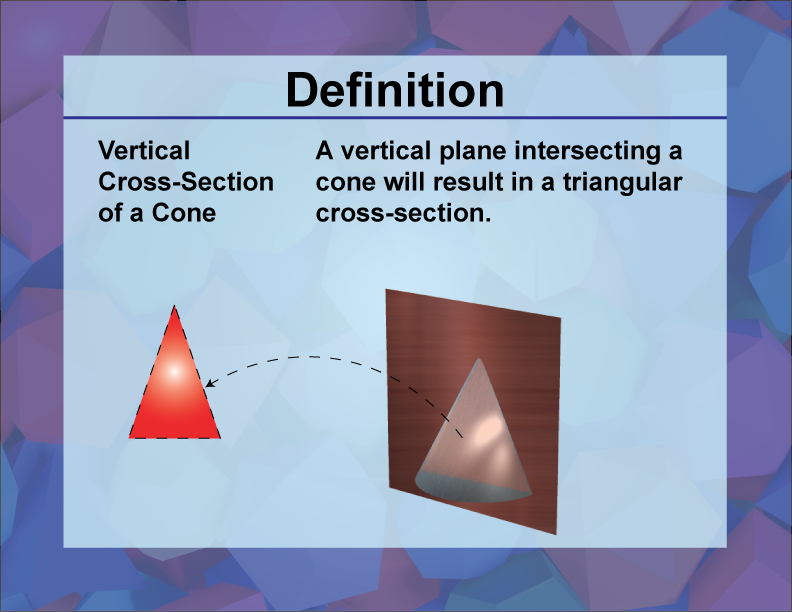
Definition
A vertical cross section of a cone is the intersection of the cone with a plane that passes through its vertex and base, resulting in a two-dimensional shape.
Description
In the study of three-dimensional geometry, understanding the concept of vertical cross sections is crucial for visualizing and analyzing the properties of 3D figures. A cone, which is a solid figure with a circular base and a single vertex, can be sliced in various ways to reveal different cross sections. When a plane intersects a cone vertically, passing through its apex and base, the resulting cross section is typically a triangle. This specific type of cross section helps in understanding the internal structure of the cone and is fundamental in applications such as volume calculation, surface area determination, and in various fields like engineering and architecture.
For a complete collection of terms related to 3D geometry click on this link: 3D Collection.
| Common Core Standards | CCSS.MATH.CONTENT.5.MD.C.3, CCSS.MATH.CONTENT.7.G.A.3 |
|---|---|
| Grade Range | 4 - 6 |
| Curriculum Nodes |
Geometry • 3D Geometry • Cones |
| Copyright Year | 2021 |
| Keywords | three-dimensional geometry, 3d Geometry, defnitions, glossary term |