Display Title
Definition--Calculus Topics--Acceleration
Display Title
Definition--Calculus Topics--Acceleration
Topic
Calculus
Definition
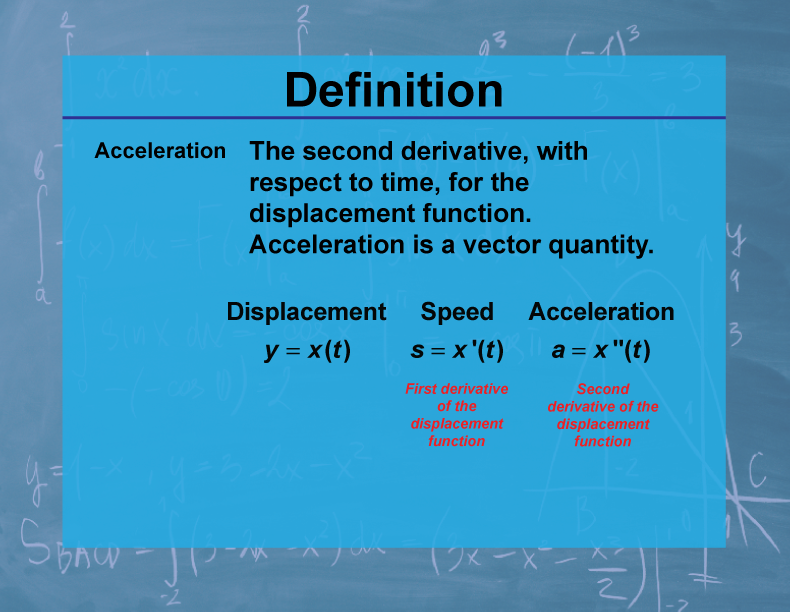
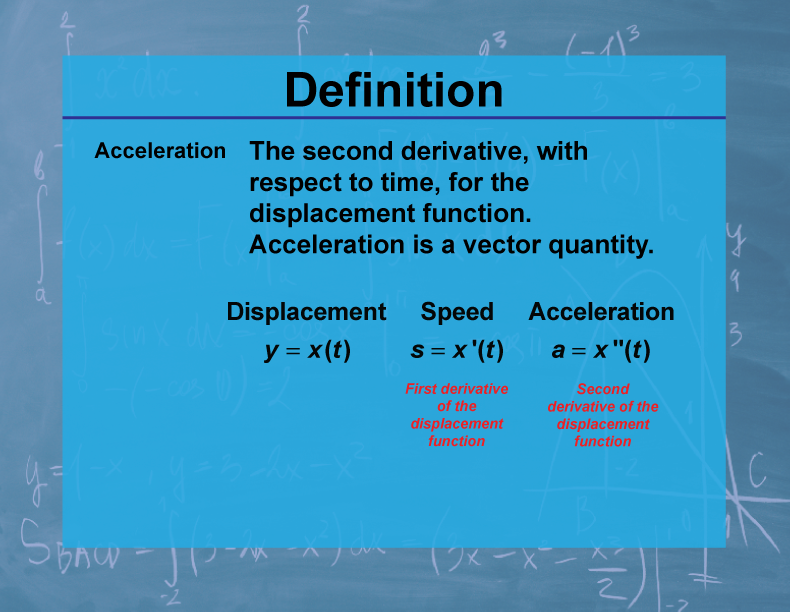
Acceleration is the rate of change of velocity with respect to time. It is the second derivative of position with respect to time.
Description
Acceleration is a fundamental concept in calculus, particularly in the study of motion and kinematics. It's crucial for understanding how objects move and change speed over time. In real-world applications, acceleration is used extensively in physics and engineering, from designing roller coasters to launching spacecraft. For example, in automotive engineering, understanding acceleration helps in designing safer vehicles and more efficient engines.
In mathematics education, acceleration serves as an excellent example of second-order derivatives and their physical interpretations. It helps students grasp the relationship between position, velocity, and acceleration, illustrating how higher-order derivatives provide increasingly detailed information about a function's behavior. This concept is essential for developing intuition about rates of change and how they apply to real-world scenarios.
Teacher's Script: "Let's consider a car starting from rest and accelerating uniformly. If its position function is s(t) = 2t2, where s is in meters and t is in seconds, we can find its velocity by taking the first derivative: v(t) = 4t m/s. The acceleration is the second derivative: a(t) = 4 m/s2. This constant acceleration of 4 m/s2 means the car's speed increases by 4 meters per second every second. Can you visualize how the car's speed changes over time?"

For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |