Display Title
Definition--Calculus Topics--Antiderivative
Display Title
Definition--Calculus Topics--Antiderivative
Topic
Calculus
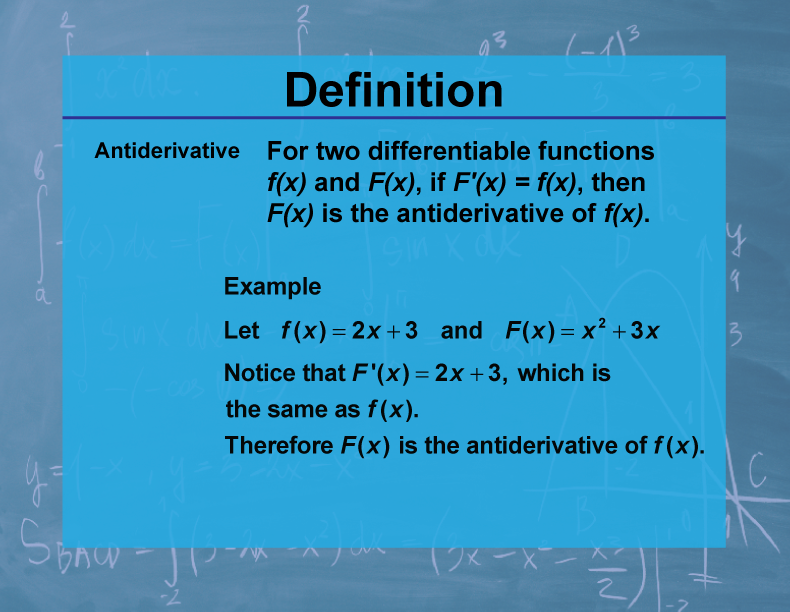
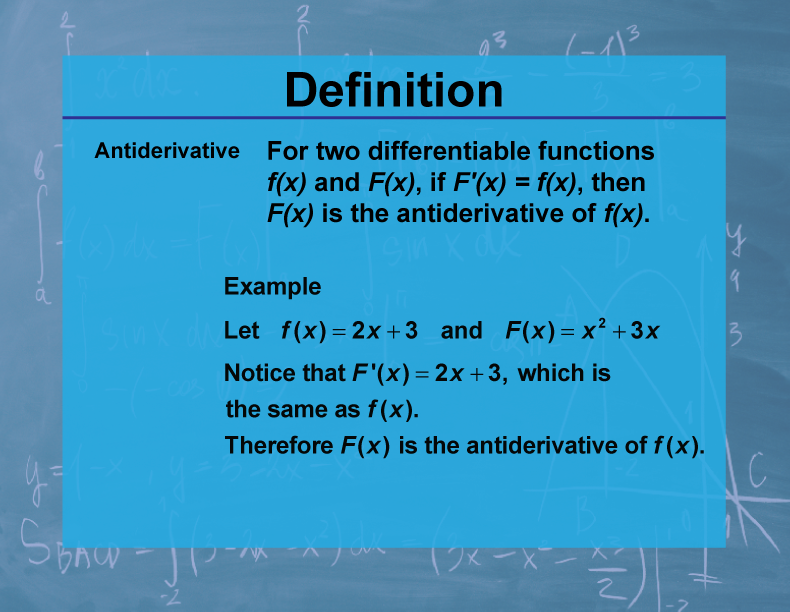
Definition
An antiderivative of a function f(x) is a function F(x) whose derivative is f(x). It is also known as the indefinite integral of f(x).
Description
The concept of antiderivatives is crucial in calculus as it forms the foundation of integral calculus. It's the reverse process of differentiation and is essential for solving many real-world problems involving accumulation and total change. In applications, antiderivatives are used to calculate areas under curves, volumes of solids, work done by a variable force, and in solving differential equations.
In mathematics education, understanding antiderivatives helps students grasp the fundamental relationship between differentiation and integration, as expressed by the Fundamental Theorem of Calculus. It introduces the idea that integration can be viewed as the inverse operation of differentiation, much like multiplication is the inverse of division. This concept is vital for developing problem-solving skills in calculus and for understanding how to model and analyze complex systems in physics, engineering, and economics.
Teacher's Script: "Imagine you're analyzing the speed of a car over time, given by the function v(t) = 3t2 + 2t. To find the distance traveled, we need to find an antiderivative of this function. One antiderivative would be s(t) = t3 + t2 + C, where C is a constant. Notice how when we differentiate s(t), we get back to v(t). The constant C represents the initial position of the car. This process allows us to calculate the car's position at any time, given its speed function."

For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |