
Display Title
Definition--Calculus Topics--Area Beneath a Curve
Display Title
Definition--Calculus Topics--Area Beneath a Curve
Topic
Calculus
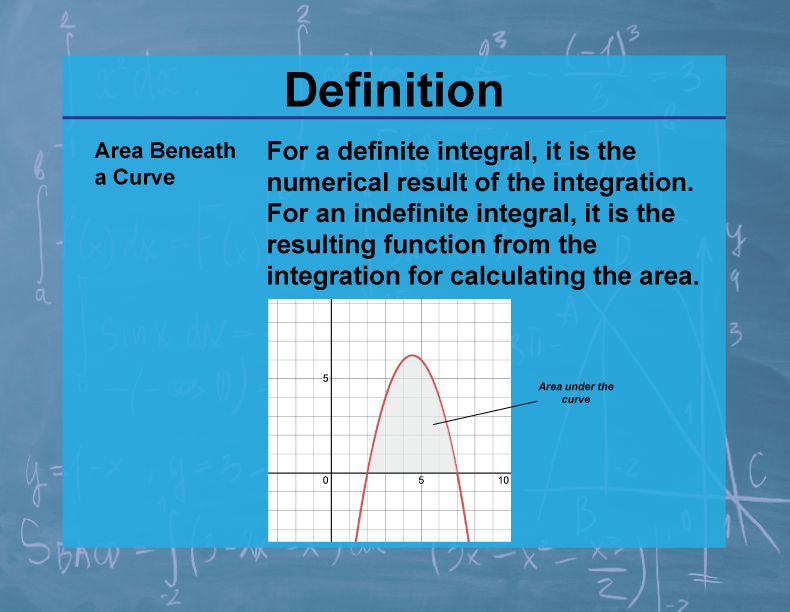
Definition
The area beneath a curve refers to the space enclosed between the graph of a function and the x-axis over a specified interval. It is calculated using definite integrals.
Description
Understanding the area beneath a curve is a fundamental concept in calculus, bridging the gap between geometry and analysis. This concept is crucial for solving a wide range of real-world problems, from calculating the total distance traveled by an object given its velocity function to determining the total amount of a substance produced over time given its production rate. In fields like physics, engineering, and economics, the area beneath a curve often represents cumulative effects or total quantities.
In mathematics education, the area beneath a curve serves as an intuitive introduction to definite integrals. It helps students visualize the connection between the graphical representation of a function and its integral, making abstract concepts more concrete. This topic is essential for developing students' understanding of the Fundamental Theorem of Calculus, which links differentiation and integration.
Teacher's Script: "Let's consider a practical example. Imagine you're monitoring the rate at which water flows into a reservoir, represented by the function r(t) = 2t + 1, where r is in cubic meters per hour and t is time in hours. To find the total amount of water that has flowed into the reservoir over 5 hours, we need to calculate the area beneath this curve from t = 0 to t = 5. This is where we use a definite integral: ∫₀⁵(2t + 1)dt. Can you see how this area represents the accumulation of water over time?"

For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |