Display Title
Definition--Calculus Topics--Concave Function
Display Title
Definition--Calculus Topics--Concave Function
Topic
Calculus
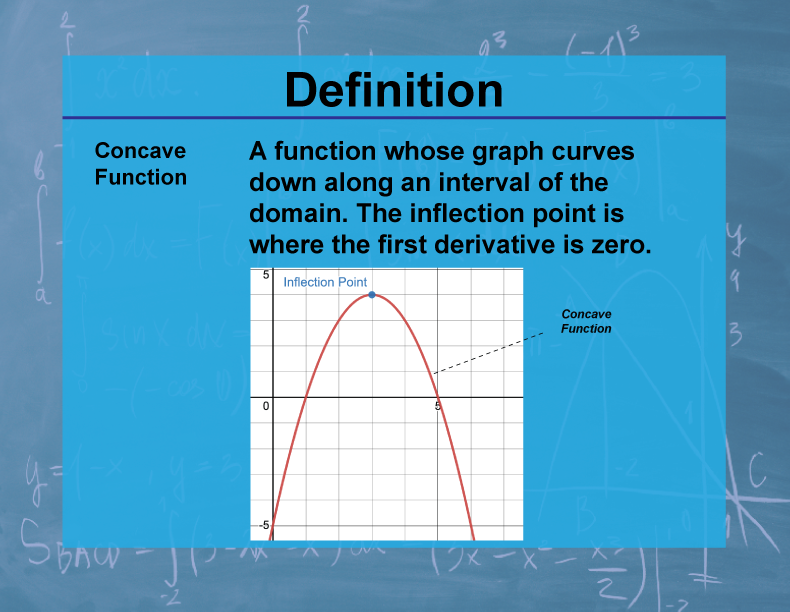
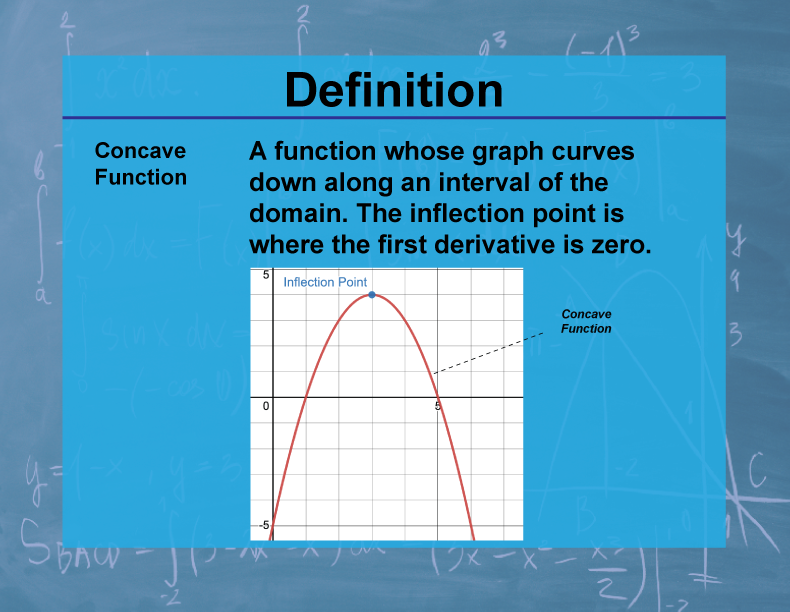
Definition
A function is concave on an interval if the line segment between any two points on the graph of the function lies below or on the graph.
Description
Concave functions play a crucial role in calculus and its applications, particularly in optimization problems. In economics, concave utility functions model diminishing marginal returns. In physics, concave functions describe various phenomena, such as the trajectory of projectiles under gravity. Understanding concavity is essential for analyzing function behavior and finding maxima and minima.
In mathematics education, the concept of concave functions helps students develop a deeper understanding of function behavior and its graphical representation. It's crucial for teaching optimization techniques and the second derivative test. This concept bridges the gap between visual intuition and rigorous mathematical analysis, enhancing students' problem-solving skills in calculus and its applications.
Teacher's Script: "Imagine you're throwing a ball upwards. The height of the ball over time forms a concave function. At first, it rises quickly, then slows down, reaches its peak, and starts falling. This is a perfect example of a concave function. The rate of change (velocity) is constantly decreasing. Can you think of other real-world examples where we might see this kind of behavior? How about the relationship between study time and test scores, or the effect of advertising on sales?"

For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |