Display Title
Definition--Calculus Topics--Definite Integral
Display Title
Definition--Calculus Topics--Definite Integral
Topic
Calculus
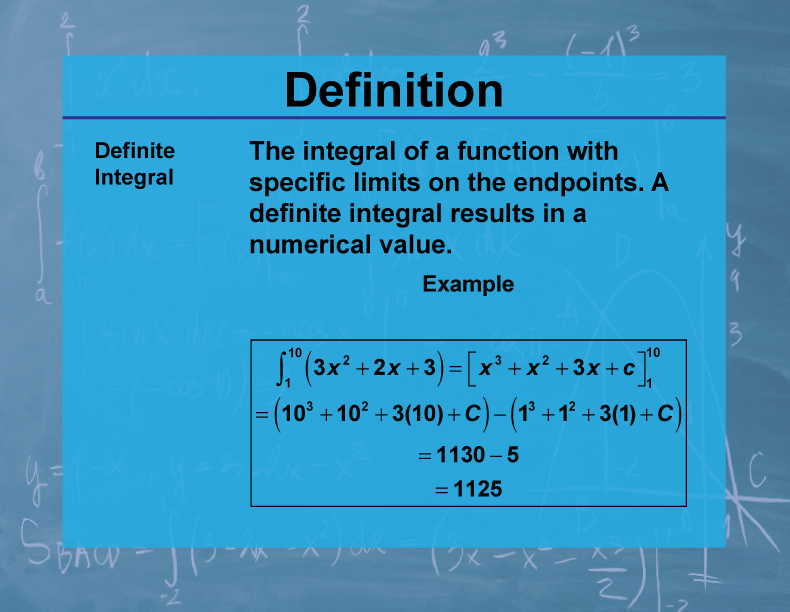
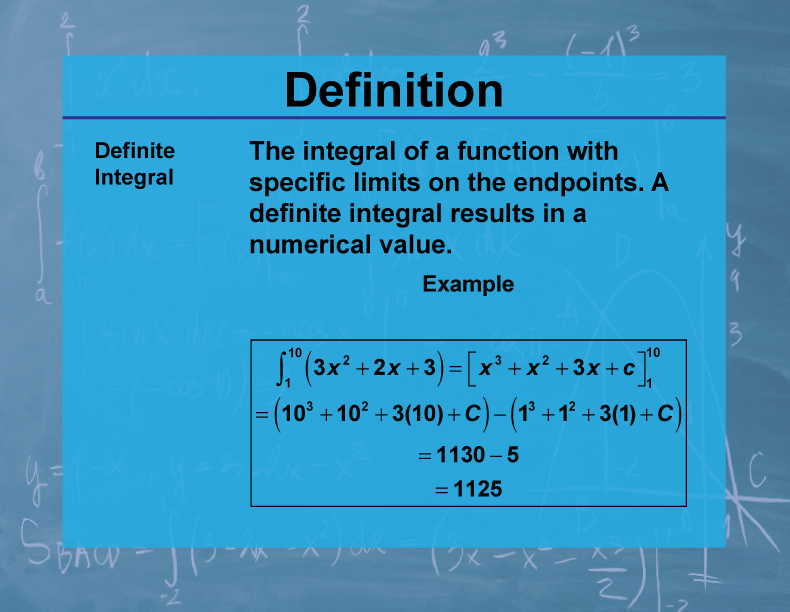
Definition
A definite integral represents the signed area between a function and the x-axis over a specified interval. It is denoted as ∫[a to b] f(x) dx, where a and b are the lower and upper limits of integration.
Description
The definite integral is a fundamental concept in calculus with wide-ranging applications in physics, engineering, and economics. It's used to calculate areas, volumes, work done by a force, and total accumulation over time. For instance, in physics, definite integrals are used to compute the work done by a variable force or the center of mass of an object with non-uniform density.
In mathematics education, understanding definite integrals helps students grasp the connection between differentiation and integration, as expressed by the Fundamental Theorem of Calculus. It introduces the idea of accumulation and provides a powerful tool for solving real-world problems involving rates of change and total quantities. This concept is crucial for developing students' ability to model and analyze complex systems in various scientific and engineering fields.
Teacher's Script: "Let's consider a practical example. Imagine you're analyzing the power consumption of a city over a day. If we have a function P(t) that represents the power usage (in megawatts) at time t (in hours), the definite integral of P(t) from t=0 to t=24 would give us the total energy consumed in a day. If P(t) = 500 + 200sin(πt/12), how would we set up the integral to find the total energy consumption? What does this calculation tell us about the city's power usage patterns?"

For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |