Display Title
Definition--Calculus Topics--Derivative of a Logarithmic Function
Display Title
Definition--Calculus Topics--Derivative of a Logarithmic Function
Topic
Calculus
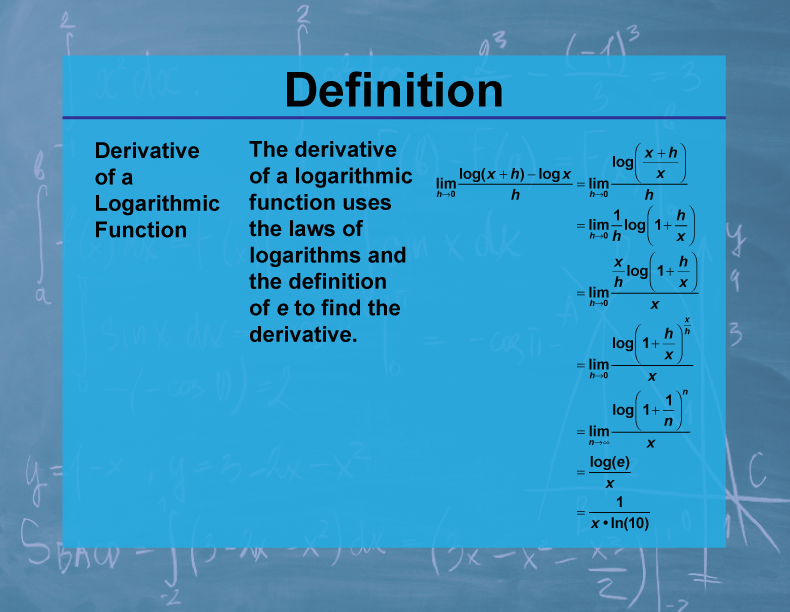
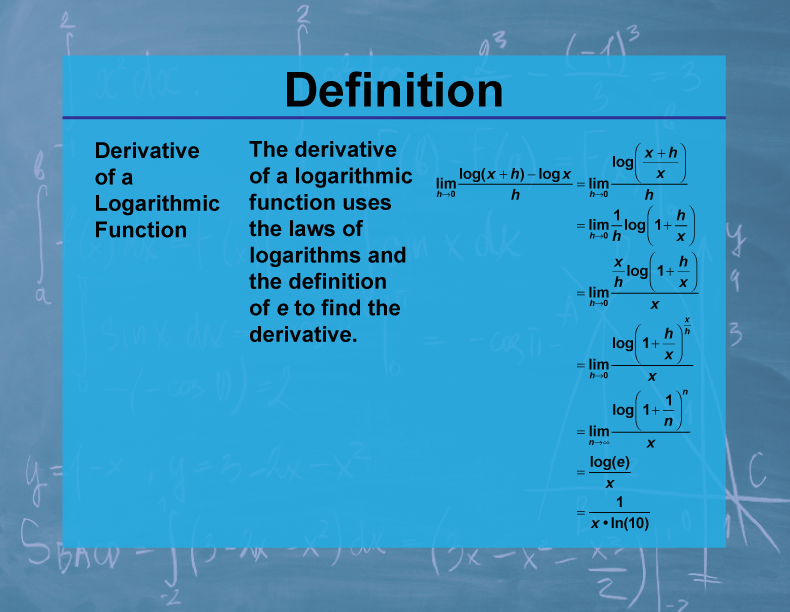
Definition
The derivative of a logarithmic function f(x) = loga(x) is given by f'(x) = 1 / (x ln(a)), where a is the base of the logarithm and a > 0, a ≠ 1.
Description
The derivative of logarithmic functions is a fundamental concept in calculus with wide-ranging applications in various fields of science and engineering. It's particularly useful in solving problems involving exponential growth or decay, such as in population dynamics, radioactive decay, or compound interest calculations.
In mathematics education, understanding the derivative of logarithmic functions helps students grasp the inverse relationship between exponential and logarithmic functions. It's crucial for solving differential equations and optimization problems involving logarithmic scales. This concept also introduces students to the natural logarithm (base e) and its special property in differentiation.
Teacher's Script: "Imagine you're analyzing the pH scale, which is logarithmic. If we have a function pH = -log[H+], where [H+] is the hydrogen ion concentration, how would we find the rate of change of pH with respect to [H+]? Using the derivative of logarithmic functions, we get dpH/d[H+] = -1 / ([H+] ln(10)). This tells us how sensitive the pH is to changes in hydrogen ion concentration. Can you think of other real-world scenarios where we might encounter logarithmic relationships and need to find their rates of change?"

For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |