
Display Title
Definition--Calculus Topics--Derivative of a Polynomial Function
Display Title
Definition--Calculus Topics--Derivative of a Polynomial Function
Topic
Calculus
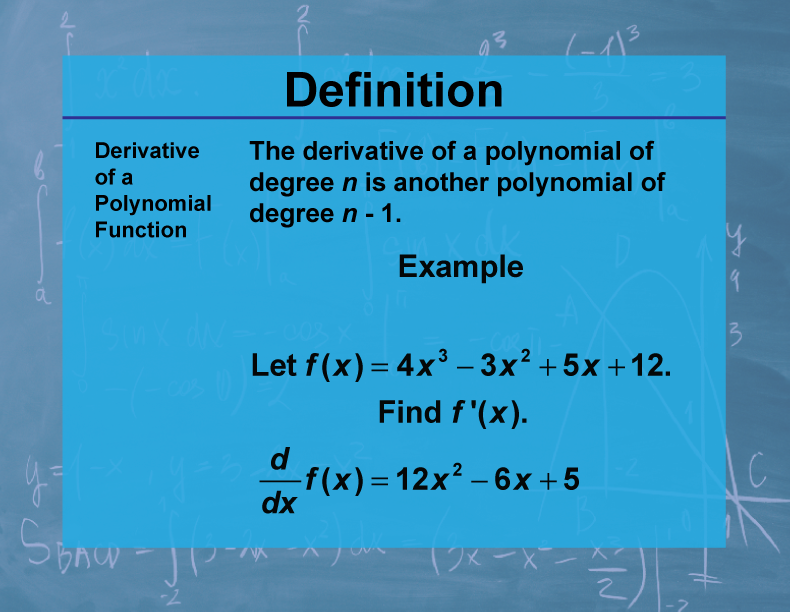
Definition
The derivative of a polynomial function f(x) = an xn + an-1 xn-1 + ... + a1 x + a0 is f'(x) = n an xn-1 + (n-1) an-1 xn-2 + ... + a1, where n is a non-negative integer.
Description
The derivative of polynomial functions is a fundamental concept in calculus, serving as a building block for understanding more complex derivatives. It has numerous applications in physics, engineering, and economics, where it's used to analyze rates of change, optimize functions, and solve differential equations.
In mathematics education, learning to differentiate polynomial functions helps students develop a strong foundation in calculus. It introduces the power rule and reinforces the concept of function transformation. This skill is essential for tackling more advanced topics in calculus and its applications in various fields.
Teacher's Script: "Let's consider a real-world example. Suppose we have a polynomial function modeling the height of a projectile over time: h(t) = -4.9t2 + 20t + 1.5, where h is in meters and t is in seconds. To find the velocity at any given time, we need to find h'(t). Using the power rule, we get h'(t) = -9.8t + 20 m/s. What does this tell us about the projectile's motion? At what time is the projectile at its highest point? How can we use this derivative to analyze the projectile's behavior throughout its flight?"

For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |