Display Title
Definition--Calculus Topics--Derivative of a Quadratic Function
Display Title
Definition--Calculus Topics--Derivative of a Quadratic Function
Topic
Calculus
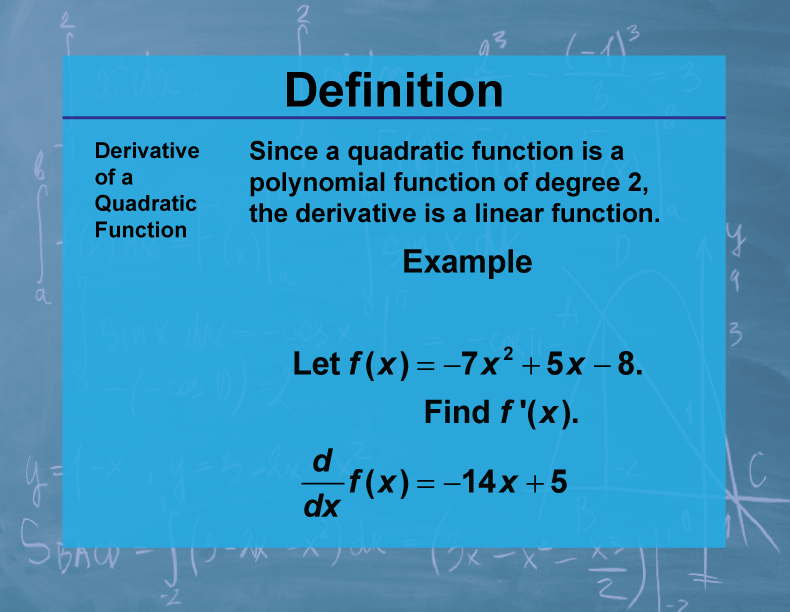
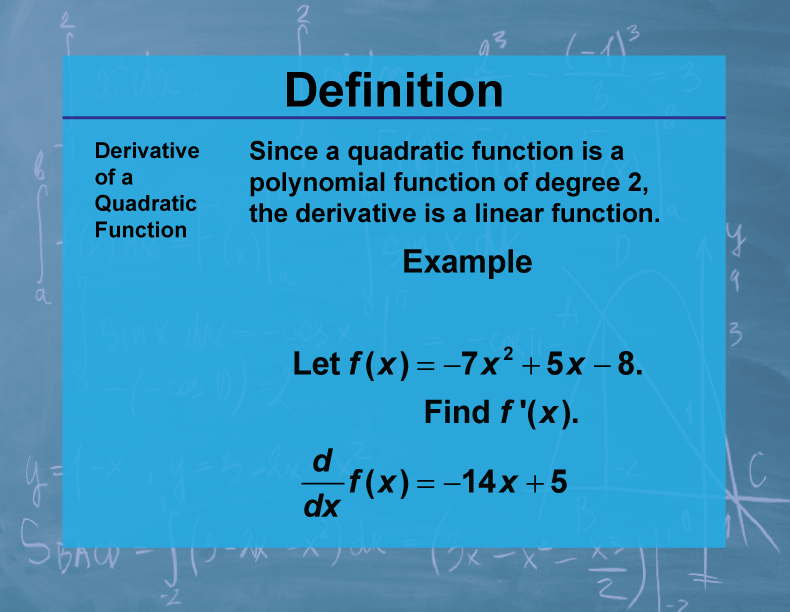
Definition
The derivative of a quadratic function f(x) = ax2 + bx + c is f'(x) = 2ax + b, where a, b, and c are constants and a ≠ 0.
Description
The derivative of quadratic functions is a crucial concept in calculus, bridging the gap between basic algebraic functions and more complex polynomial derivatives. It has wide-ranging applications in physics, economics, and engineering, particularly in problems involving motion, optimization, and rate of change analysis.
In mathematics education, understanding the derivative of quadratic functions helps students visualize the relationship between a parabola and its tangent lines. It's an excellent introduction to the concept of instantaneous rate of change and provides a foundation for understanding higher-degree polynomial derivatives. This concept is essential for solving real-world problems involving acceleration, profit maximization, and other quadratic models.
Teacher's Script: "Imagine you're designing a parabolic satellite dish. The cross-section of the dish can be modeled by the quadratic function y = -0.2x2 + 2, where x and y are in meters. To find the slope of the dish at any point, we need its derivative: y' = -0.4x. How can we use this to determine the angle of the dish at different points? At what point is the dish horizontal? How might this information be useful in positioning the satellite receiver?"

For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |