Display Title
Definition--Calculus Topics--Derivative of a Rational Function
Display Title
Definition--Calculus Topics--Derivative of a Rational Function
Topic
Calculus
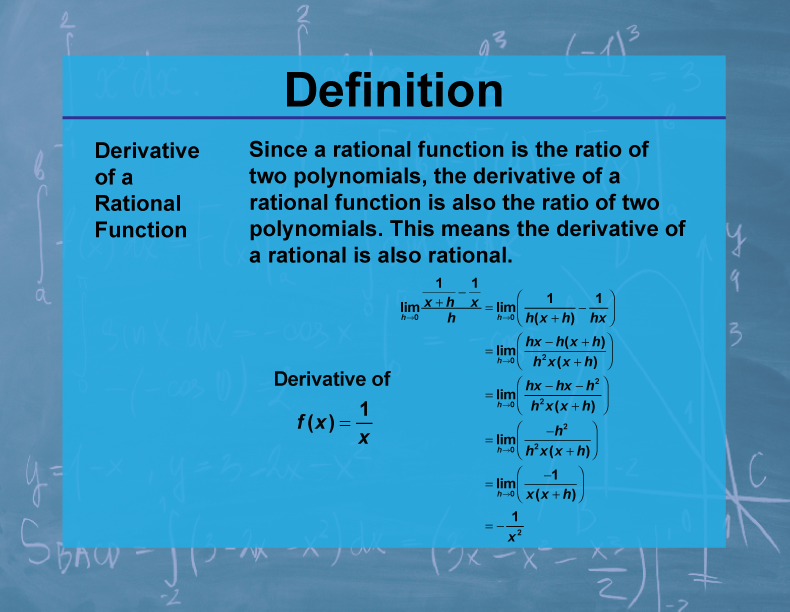
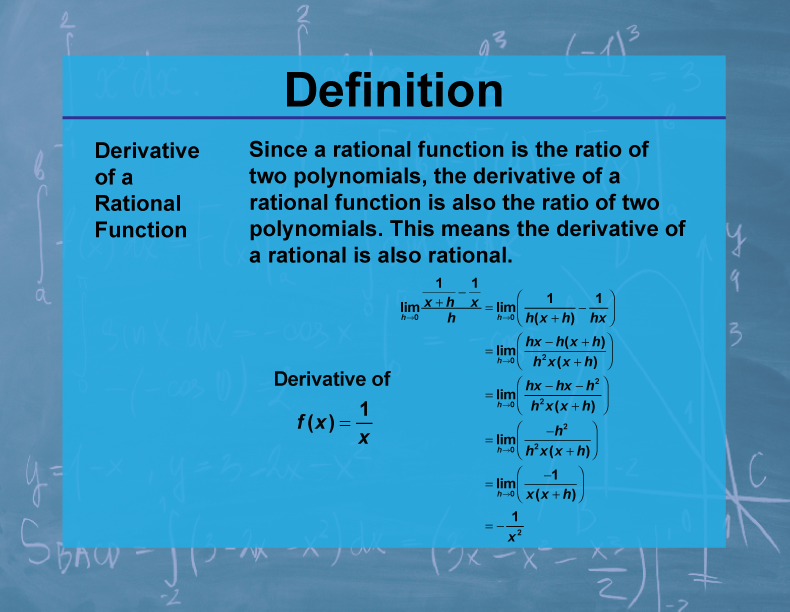
Definition
The derivative of a rational function f(x) = P(x)/Q(x), where P(x) and Q(x) are polynomials and Q(x) ≠ 0, is given by the quotient rule: f'(x) = (Q(x)P'(x) - P(x)Q'(x)) / [Q(x)]2.
Description
The derivative of rational functions is a fundamental concept in calculus with significant applications in various fields of science and engineering. It's particularly useful in analyzing rates of change in complex systems, solving differential equations, and optimizing processes in fields like economics, physics, and engineering.
In mathematics education, understanding the derivative of rational functions helps students grasp more advanced calculus concepts. It reinforces the application of the quotient rule and provides insight into function behavior near vertical asymptotes and holes. This concept is crucial for developing problem-solving skills in calculus and its applications in real-world scenarios.
Teacher's Script: "Let's consider a practical example from chemistry. The rate of a certain chemical reaction is modeled by the function r(t) = (2t2 + 3) / (t + 1), where r is the reaction rate and t is time in minutes. To find how the rate is changing at any given time, we need to find r'(t). How can we interpret this derivative? At what time is the reaction rate changing most rapidly? How might this information be useful in controlling the reaction?"
For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |