Display Title
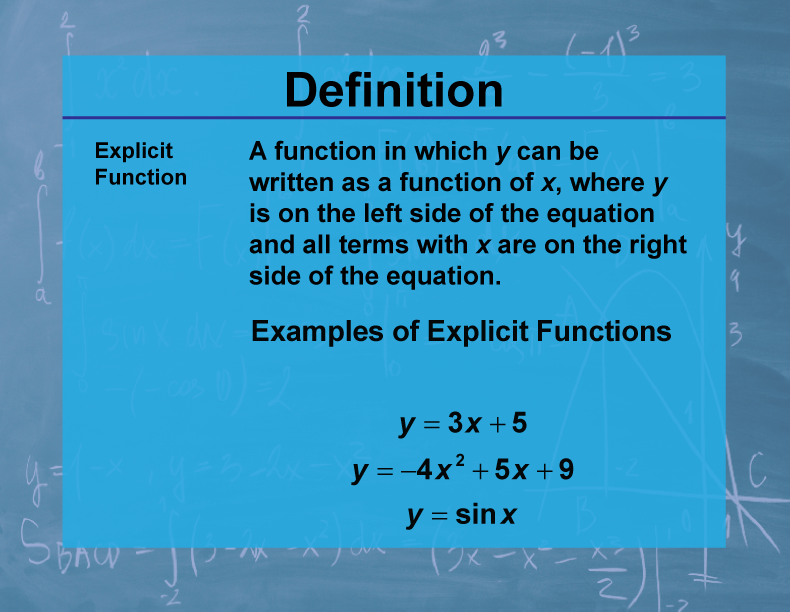
Definition--Calculus Topics--Explicit Function
Display Title
Definition--Calculus Topics--Explicit Function

Topic
Calculus
Definition
An explicit function is a function where the dependent variable is expressed directly in terms of the independent variable. It typically takes the form y = f(x).
Description
Explicit functions are fundamental in calculus and mathematical modeling. They provide a clear and direct relationship between variables, making them easier to analyze, differentiate, and integrate. In real-world applications, explicit functions are used to model various phenomena in physics, economics, and engineering where one quantity directly depends on another.
In mathematics education, understanding explicit functions is crucial for developing students' ability to interpret and manipulate mathematical expressions. It forms the basis for more advanced concepts in calculus, such as differentiation rules and integration techniques. The contrast between explicit and implicit functions helps students appreciate the different ways relationships between variables can be expressed and the implications for problem-solving strategies.
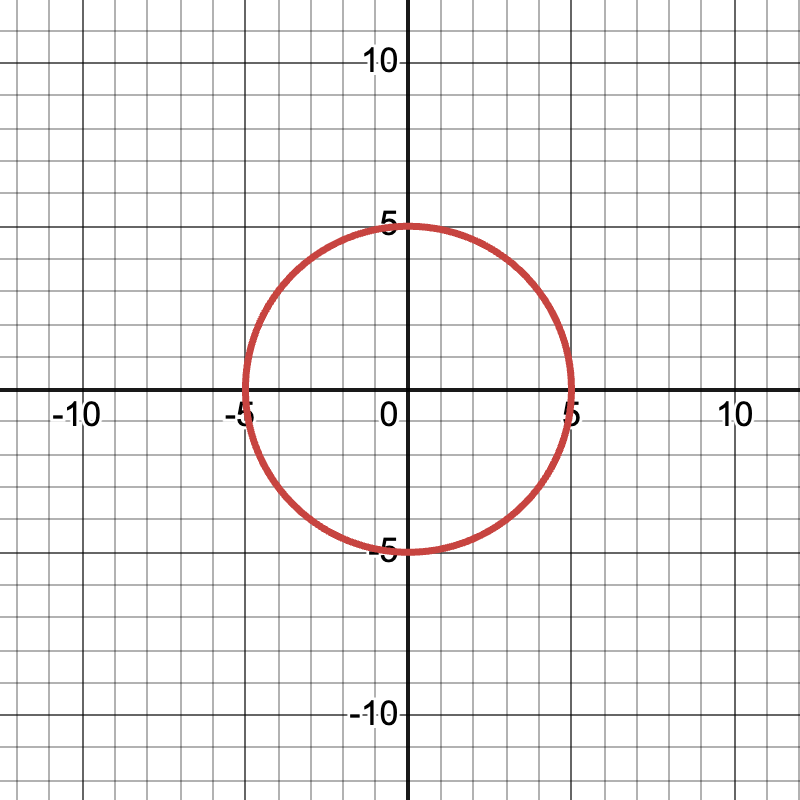
Teacher's Script: "Let's consider the function y = 2x + 3. This is an explicit function because we can directly calculate y for any given x. Now, compare this to the equation x2 + y2 = 25. Can we easily solve for y in terms of x? This second equation is an implicit function. In calculus, we often need to convert implicit functions to explicit forms. For example, how would we find dy/dx for our circle equation? This is where implicit differentiation comes in handy. Let's explore how we can use these concepts to solve real-world problems, like modeling the trajectory of a projectile or analyzing the growth of an investment over time."
For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |