Display Title
Definition--Calculus Topics--Fundamental Theorem of Calculus
Display Title
Definition--Calculus Topics--Fundamental Theorem of Calculus
Topic
Calculus
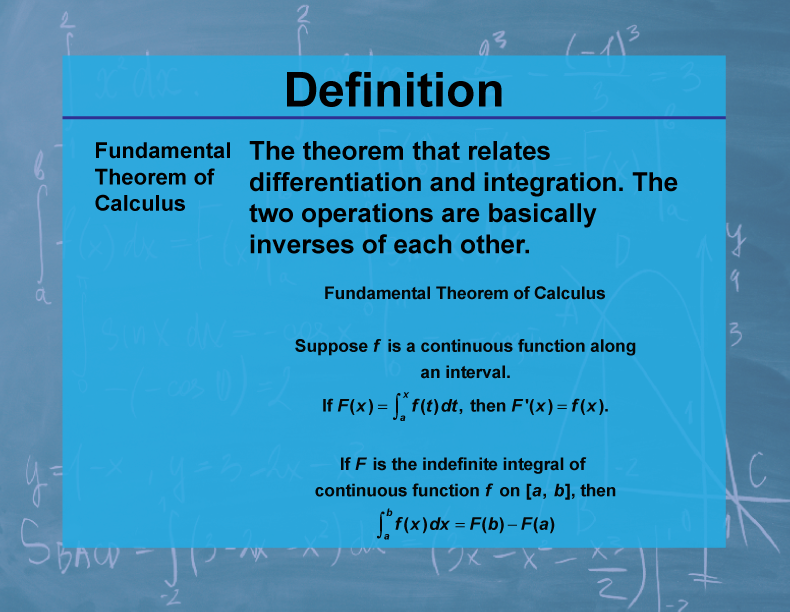
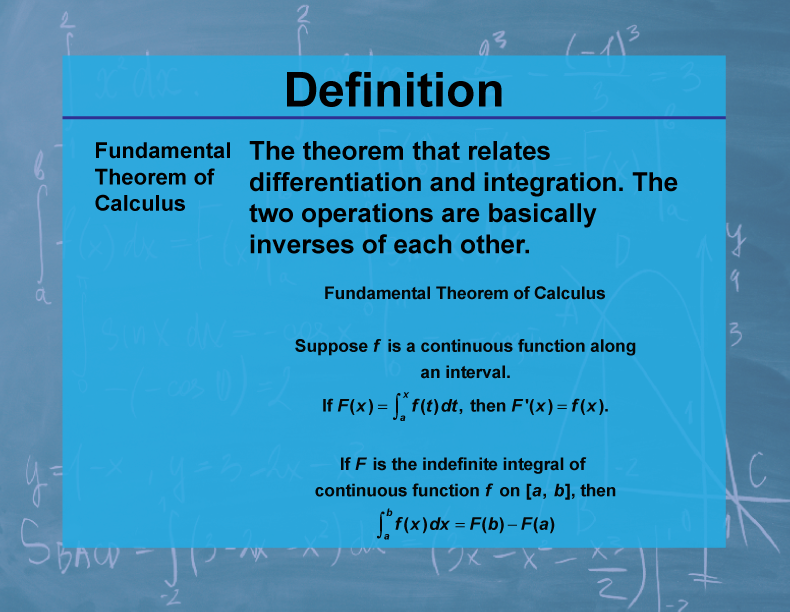
Definition
The Fundamental Theorem of Calculus establishes the relationship between differentiation and integration. It consists of two parts: Part 1 states that the derivative of an integral function is the original function, and Part 2 provides a method for evaluating definite integrals using antiderivatives.
Description
The Fundamental Theorem of Calculus is a cornerstone of calculus, unifying the concepts of differentiation and integration. It has profound implications in mathematics and its applications across various fields. In physics, it's used to derive equations of motion and analyze energy in systems. In engineering, it's crucial for understanding and designing systems involving rates of change and accumulation. The theorem also plays a vital role in probability theory and statistics.
In mathematics education, the Fundamental Theorem of Calculus represents a pivotal moment in students' understanding of calculus. It demonstrates how seemingly disparate concepts - derivatives and integrals - are intrinsically linked. This realization often leads to a deeper appreciation of the elegance and power of calculus. The theorem also provides practical tools for solving a wide range of problems, from finding areas and volumes to analyzing complex systems in physics and engineering.
Teacher's Script: "Let's consider a car's motion. If we know the car's velocity function v(t), the Fundamental Theorem tells us two important things. First, the distance traveled from time a to b is the area under the velocity curve, which we can calculate using a definite integral. Second, if we integrate the velocity function, we get the position function, and differentiating this position function gets us back to velocity. Isn't that remarkable? This theorem connects the dots between position, velocity, and acceleration. Now, let's explore how we can apply this concept to other scenarios, like analyzing population growth rates or calculating work done by a variable force."
For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |