
Display Title
Definition--Calculus Topics--Implicit Differentiation
Display Title
Definition--Calculus Topics--Implicit Differentiation
Topic
Calculus
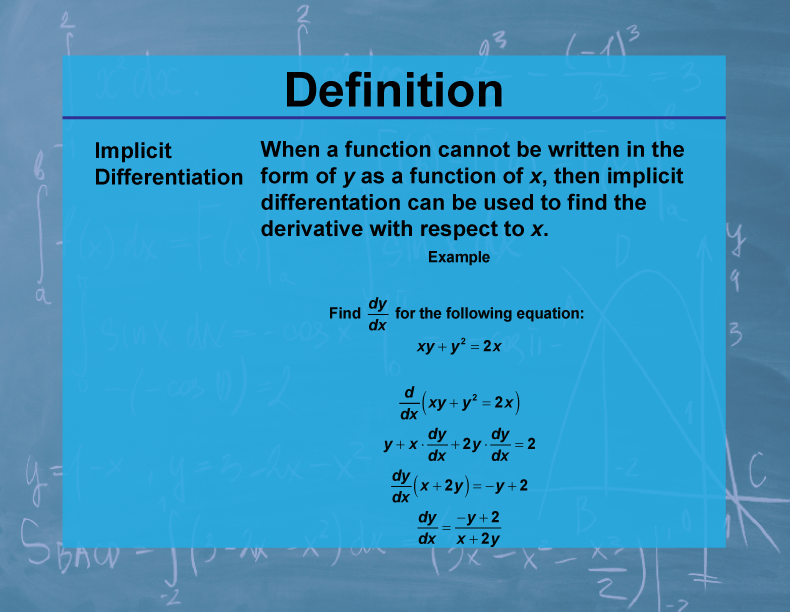
Definition
Implicit differentiation is a method used to find the derivative of an implicitly defined function. It involves differentiating both sides of an equation with respect to x, treating y as a function of x, and then solving for dy/dx.
Description
Implicit differentiation is a powerful technique in calculus that allows us to find derivatives of functions that are not explicitly defined in terms of one variable. It's particularly useful for dealing with equations that can't be easily solved for y in terms of x, such as circles, ellipses, and other conic sections. This method extends the applicability of differentiation to a wider range of functions and relationships.
In mathematics education, implicit differentiation helps students develop a deeper understanding of the chain rule and the nature of functional relationships. It challenges students to think about variables in a more abstract way and prepares them for more advanced topics in multivariable calculus. This technique is also crucial in many applications, including physics, engineering, and economics, where relationships between variables are often expressed implicitly.
Teacher's Script: "Let's consider the equation of a circle: x2 + y2 = 25. How can we find the slope of the tangent line at any point on this circle? We can't solve this for y explicitly, so we need to use implicit differentiation. We'll differentiate both sides with respect to x, remembering that y is a function of x. This gives us 2x + 2y(dy/dx) = 0. From here, we can solve for dy/dx to get the slope at any point. How might this technique be useful in analyzing more complex shapes or relationships?"
For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |