Display Title
Definition--Calculus Topics--Indefinite Integral
Display Title
Definition--Calculus Topics--Indefinite Integral
Topic
Calculus
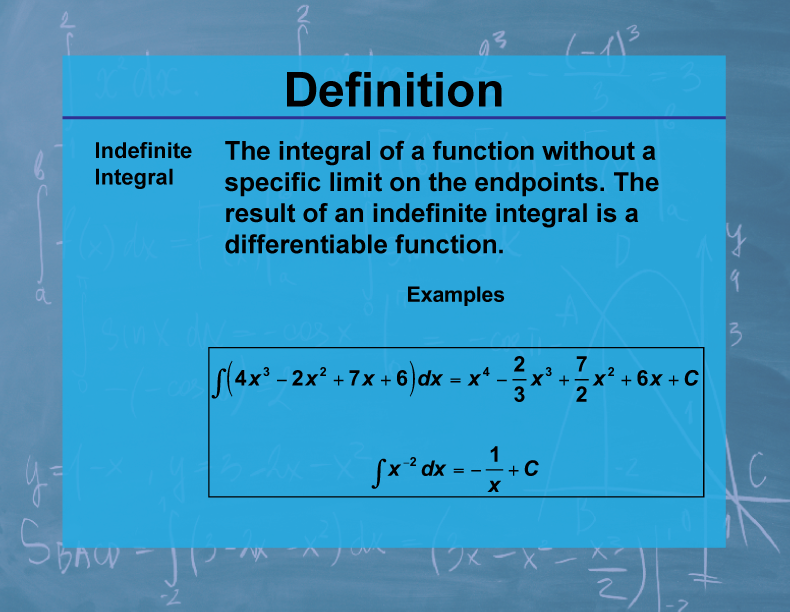
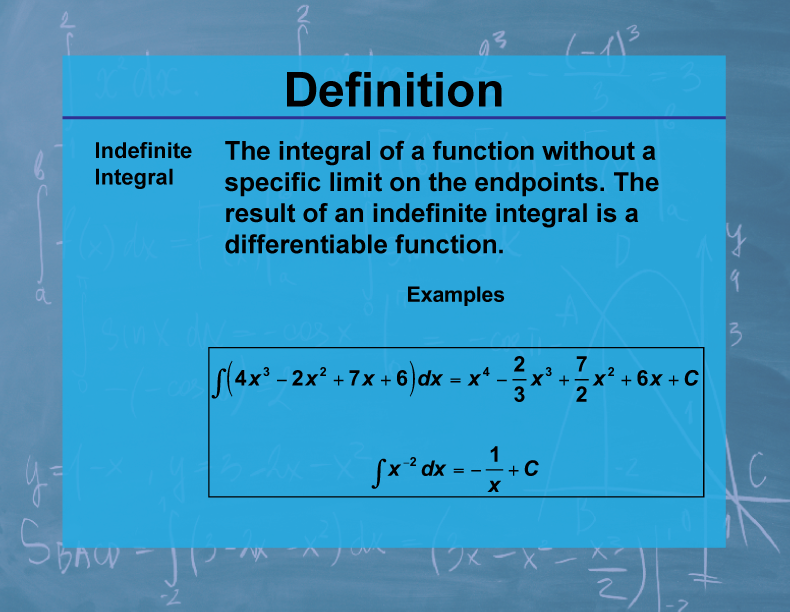
Definition
An indefinite integral of a function f(x) is a function F(x) whose derivative is f(x). It is represented as ∫f(x)dx = F(x) + C, where C is an arbitrary constant of integration.
Description
Indefinite integrals are fundamental to calculus, representing the reverse process of differentiation. They are crucial in solving differential equations, modeling physical systems, and understanding accumulation processes. In physics, indefinite integrals are used to find position from velocity, or velocity from acceleration. In economics, they can be used to calculate total cost from marginal cost functions.
In mathematics education, indefinite integrals help students understand the relationship between differentiation and integration, as expressed in the Fundamental Theorem of Calculus. They provide practice in pattern recognition and algebraic manipulation, and introduce the concept of a family of functions differing only by a constant. This prepares students for more advanced topics in calculus and differential equations.
Teacher's Script: "Imagine you're analyzing the velocity of a car, given by v(t) = 3t2 + 2t. To find the position function, we need to find the indefinite integral of v(t). This gives us s(t) = t3 + t2 + C. The constant C represents the initial position. How does this relate to the car's motion? Why do we need the constant C? How might we use this in real-world scenarios like traffic flow analysis or projectile motion?"
For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |