
Display Title
Definition--Calculus Topics--Integration by Substitution
Display Title
Definition--Calculus Topics--Integration by Substitution
Topic
Calculus
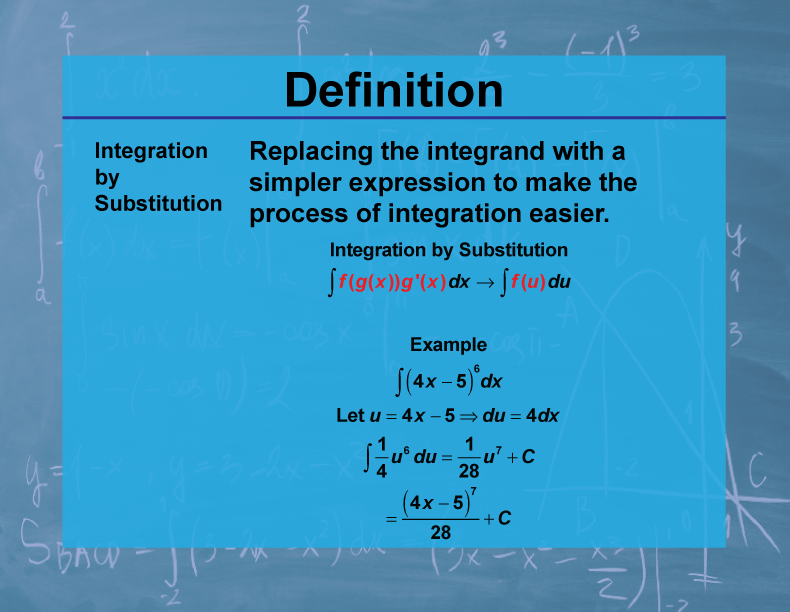
Definition
Integration by substitution is a method used to evaluate integrals by substituting a new variable for a part of the integrand, often simplifying the integral.
Description
Integration by substitution is a fundamental technique in calculus, often considered the counterpart to the chain rule for derivatives. It's particularly useful for integrating composite functions and functions involving trigonometric, exponential, or logarithmic terms. This method is widely applied in physics and engineering to solve problems involving changing variables or coordinate systems.
In mathematics education, learning integration by substitution helps students develop problem-solving skills and recognize patterns in complex integrals. It encourages students to think creatively about transforming integrals into more manageable forms. This technique is essential for tackling a wide range of integration problems and prepares students for more advanced calculus concepts.
Teacher's Script: "Let's consider the integral ∫ x cos(x2) dx. At first glance, this might seem challenging, but with substitution, we can simplify it. If we let u = x2, what happens to our integral? How does dx change in terms of du? After substitution, our integral becomes much simpler: (1/2) ∫ cos(u) du. Can you think of real-world scenarios where changing variables like this might be useful? Perhaps in physics when converting between different coordinate systems?"
For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |