Display Title
Definition--Calculus Topics--Inverse Function
Display Title
Definition--Calculus Topics--Inverse Function
Topic
Calculus
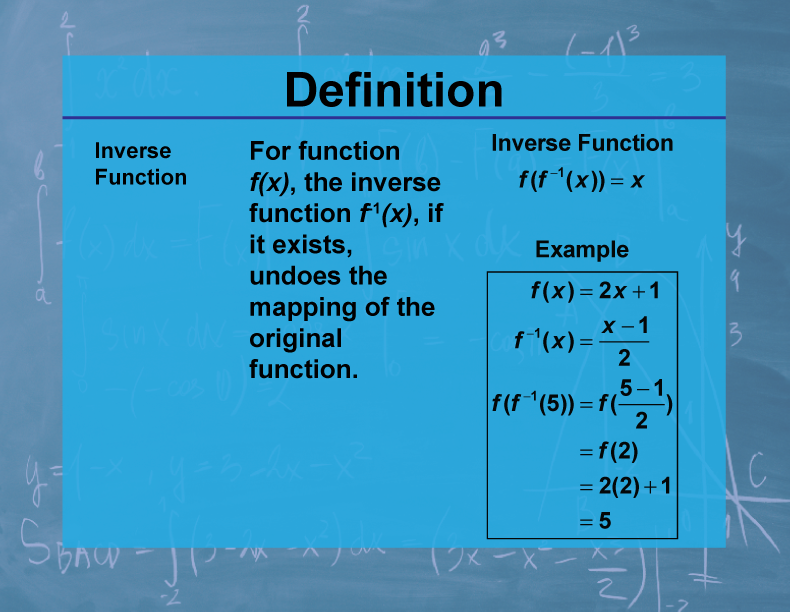
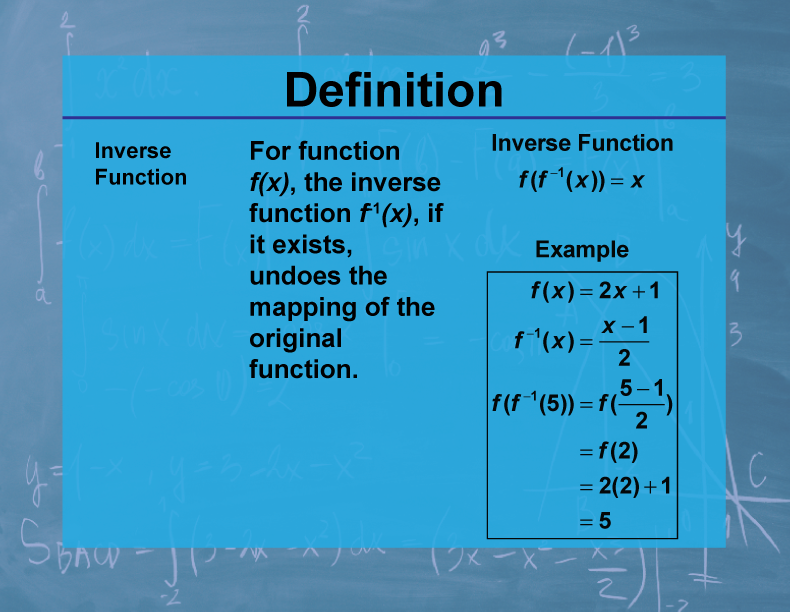
Definition
An inverse function f-1 is a function that "undoes" what f does. For every x in the domain of f, f-1(f(x)) = x, and for every y in the range of f, f(f-1(y)) = y.
Description
Inverse functions play a crucial role in calculus and have wide-ranging applications in mathematics and science. They are particularly important in solving equations, modeling physical phenomena, and in fields like cryptography. In calculus, understanding inverse functions is essential for working with inverse trigonometric functions, logarithms, and for solving certain types of differential equations.
In mathematics education, the concept of inverse functions helps students deepen their understanding of function behavior and relationships. It encourages critical thinking about function composition and reversibility. Learning about inverse functions also prepares students for more advanced topics in calculus, such as implicit differentiation and inverse function theorem.
Teacher's Script: "Let's think about a real-world example of inverse functions. Imagine you have a function that converts Celsius to Fahrenheit: F(C) = 9C/5 + 32. What would the inverse function do? That's right, it would convert Fahrenheit back to Celsius. How could we find this inverse function algebraically? Now, let's consider the graphs of these functions. What do you notice about their relationship? They're reflections of each other over the line y = x. Can you think of other pairs of functions and their inverses that we use in everyday life?"
For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |