
Display Title
Definition--Calculus Topics--Left-Hand Limit
Display Title
Definition--Calculus Topics--Left-Hand Limit
Topic
Calculus
Definition
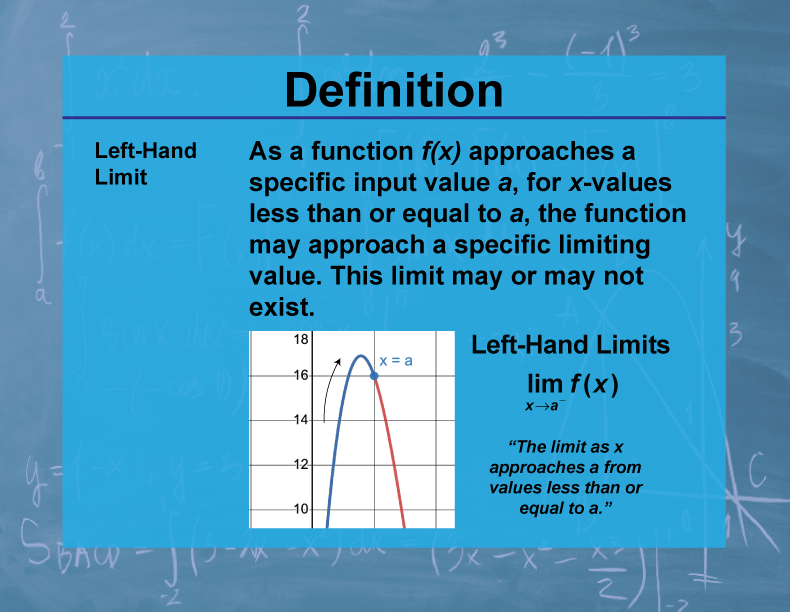
The left-hand limit of a function f(x) as x approaches a, denoted as lim[x→a-] f(x), is the value that f(x) approaches as x gets arbitrarily close to a from values less than a.
Description
Left-hand limits are crucial in understanding function behavior and continuity. They help us analyze functions at points where they might not be continuous or where their behavior might change. In real-world applications, left-hand limits can be used to model situations where a system's behavior just before a critical point is important.
In mathematics education, understanding left-hand limits helps students develop a more nuanced view of function behavior. It's particularly important for grasping the concept of continuity and preparing for more advanced topics in calculus. Left-hand limits, along with right-hand limits, form the foundation for understanding limits in general.
Teacher's Script: "Imagine you're approaching a speed bump in a car. The left-hand limit would be like considering your speed as you get very close to the bump, but just before you reach it. Let's look at a function f(x) = (x2 - 1) / (x - 1) as x approaches 1 from the left. What happens to the function values? Can we determine a left-hand limit? How does this compare to the function's actual value at x = 1? This example helps us understand why we need to consider limits from both sides of a point."
For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |