Display Title
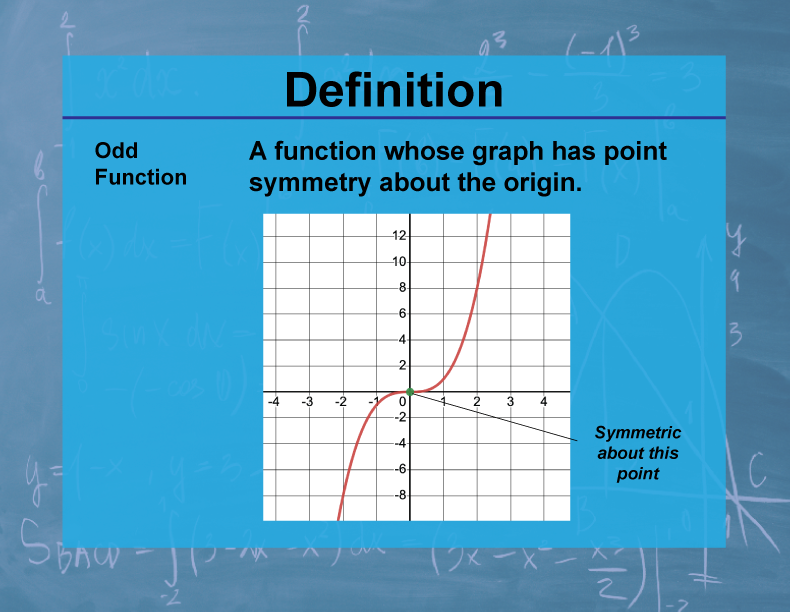
Definition--Calculus Topics--Odd Function
Display Title
Definition--Calculus Topics--Odd Function

Topic
Calculus
Definition
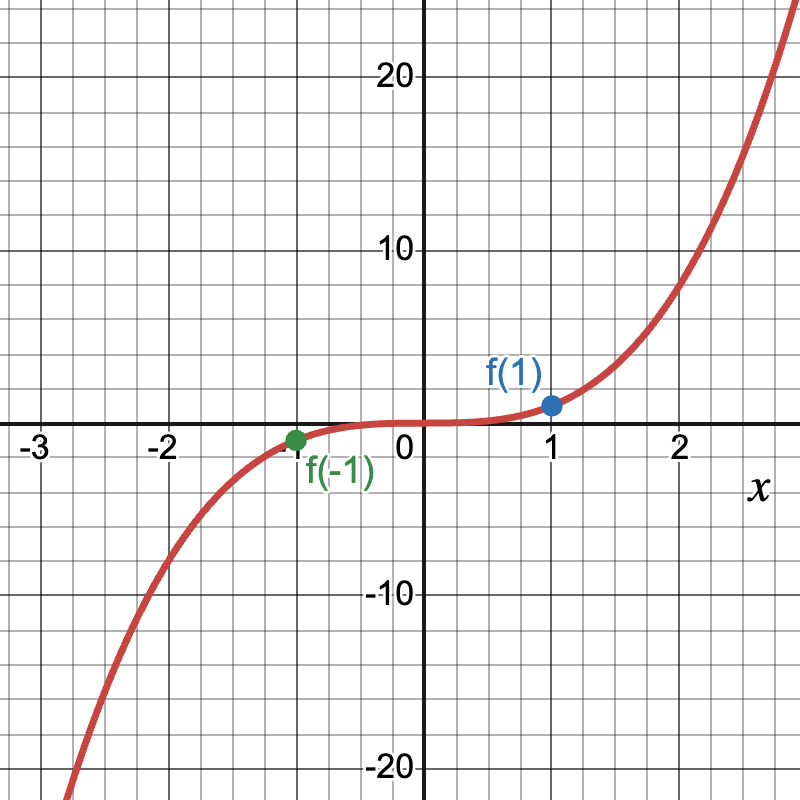
An odd function is a function f(x) that satisfies the condition f(-x) = -f(x) for all x in the domain of f. Graphically, odd functions are symmetric about the origin.
Description
Odd functions play a significant role in calculus and many areas of mathematics and physics. Their symmetry property makes them particularly useful in modeling physical phenomena that exhibit antisymmetric behavior, such as the sine function in trigonometry or the position of a simple harmonic oscillator. In calculus, odd functions have special properties with respect to integration and differentiation, which can simplify calculations and lead to important insights.
In mathematics education, the concept of odd functions helps students develop a deeper understanding of function symmetry and its implications. It's an important tool for analyzing and graphing functions, and it provides a foundation for more advanced topics such as Fourier series in higher mathematics. Understanding odd functions also enhances students' ability to recognize patterns and symmetries in mathematical expressions and real-world phenomena.
Teacher's Script: "Let's consider the function f(x) = x3. If we input any number and its negative, like 2 and -2, we get outputs that are negatives of each other: f(2) = 8 and f(-2) = -8. This antisymmetry is what makes it an odd function. Now, let's graph it. Notice how the graph is symmetric if we rotate it 180° around the origin. Can you think of other functions that might have this property? How about sin(x) or x5? How does this symmetry affect the area under the curve when we integrate an odd function from -a to a?"
For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |