Display Title
Definition--Calculus Topics--Quotient Rule
Display Title
Definition--Calculus Topics--Quotient Rule
Topic
Calculus
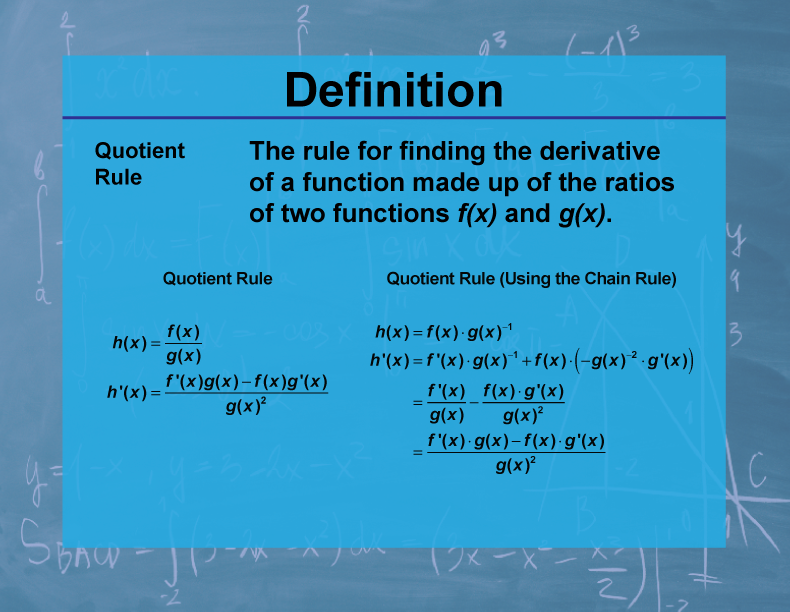
Definition
The Quotient Rule states that for two differentiable functions f(x) and g(x), the derivative of their quotient is given by: d/dx[f(x)/g(x)] = [g(x)f'(x) - f(x)g'(x)] / [g(x)]2, where g(x) ≠ 0.
Description
The Quotient Rule is a fundamental differentiation technique in calculus, used when we need to find the derivative of one function divided by another. It's particularly useful in situations where simplification before differentiation is not possible or practical. The Quotient Rule, along with the Product Rule and Chain Rule, forms the core set of rules for differentiating complex functions.
In mathematics education, learning the Quotient Rule helps students understand how to handle more complex functions and reinforces the idea that differentiation is a systematic process. It also provides opportunities to practice algebraic manipulation and simplification. Understanding and applying the Quotient Rule is crucial for solving problems in various fields, including physics, engineering, and economics.
Teacher's Script: "Let's apply the Quotient Rule to f(x) = (x2 + 1) / (x - 2). Remember, we put the denominator times the derivative of the numerator, minus the numerator times the derivative of the denominator, all over the denominator squared. Can you work through this step-by-step? How would you simplify the result? This process helps us understand how the rate of change of a quotient depends on the rates of change of its parts."
For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |