Display Title
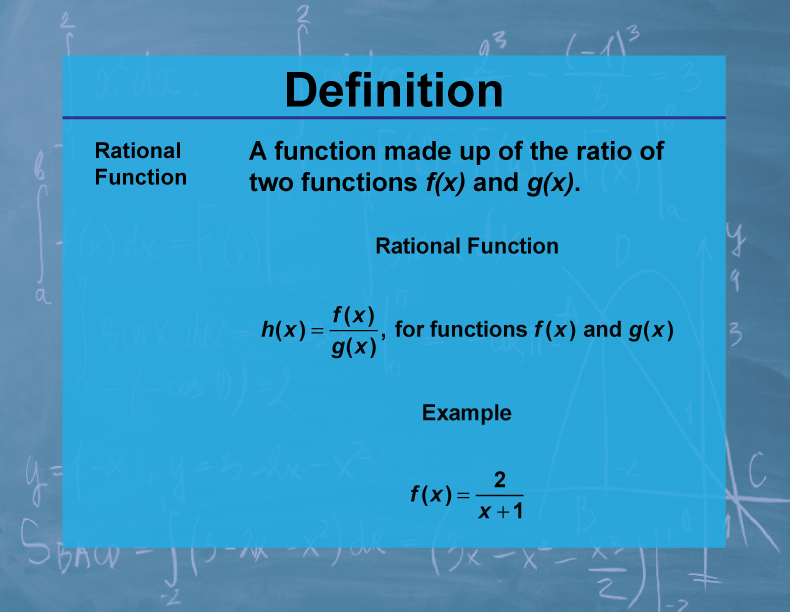
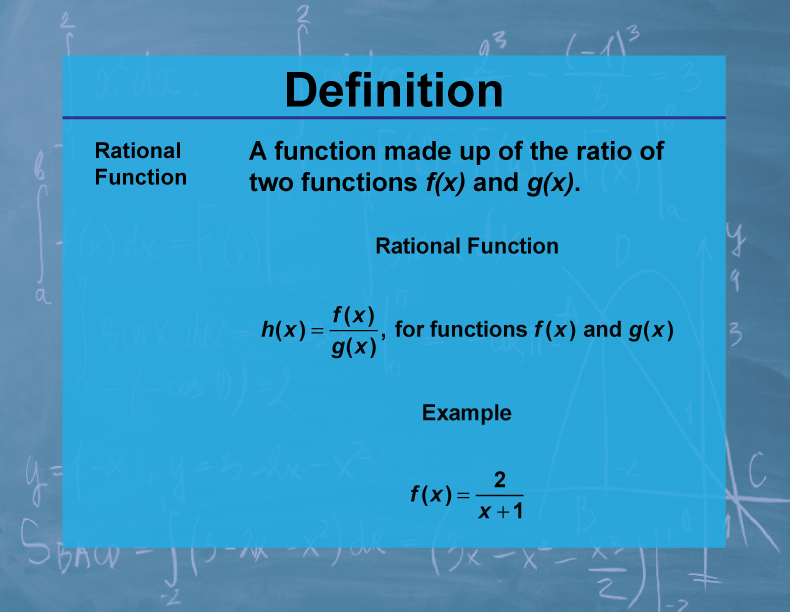
Definition--Calculus Topics--Rational Function
Display Title
Definition--Calculus Topics--Rational Function
Topic
Calculus
Definition
A rational function is a function that can be expressed as the ratio of two polynomials, P(x)/Q(x), where Q(x) ≠ 0.
Description
Rational functions are a crucial class of functions in calculus and algebra, with wide-ranging applications in science, engineering, and economics. They exhibit interesting behaviors such as asymptotes, holes, and discontinuities, making them valuable for modeling complex real-world phenomena. The study of rational functions involves analyzing their domain, range, intercepts, and end behavior.
In mathematics education, rational functions provide an excellent opportunity to integrate concepts from algebra and calculus. They challenge students to think about function behavior, limits, and continuity in more sophisticated ways. Understanding rational functions is essential for more advanced topics in calculus, such as partial fractions decomposition and improper integrals.
Teacher's Script: "Let's consider the rational function f(x) = (x2 - 1) / (x - 1). What happens as x approaches 1? As x approaches infinity? Can you identify any vertical or horizontal asymptotes? By analyzing these aspects, we can understand how rational functions behave and why they're so useful in modeling situations like chemical reaction rates or electrical circuits. How might simplifying this function change our analysis?"
For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |