Display Title
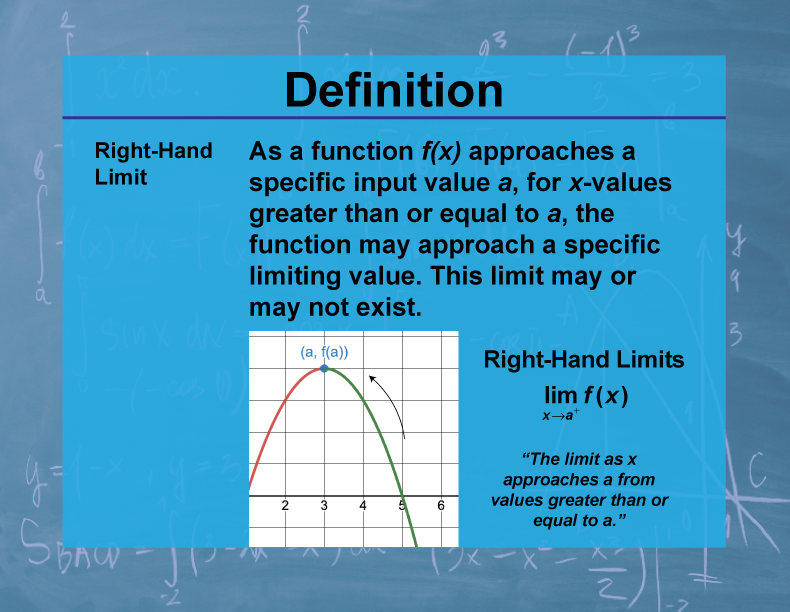
Definition--Calculus Topics--Right-Hand Limit
Display Title
Definition--Calculus Topics--Right-Hand Limit
Topic
Calculus
Definition
The right-hand limit of a function f(x) as x approaches a, denoted as lim[x→a+] f(x), is the value that f(x) approaches as x gets arbitrarily close to a from values greater than a.
Description
Right-hand limits are crucial in understanding function behavior, especially at points of discontinuity or where the function is defined differently on either side of a point. They are fundamental in analyzing piecewise functions, step functions, and in understanding continuity. In real-world applications, right-hand limits can model situations where a system's behavior just after a critical point is important.
In mathematics education, understanding right-hand limits helps students develop a more nuanced view of function behavior. It's particularly important for grasping the concept of continuity and preparing for more advanced topics in calculus. Right-hand limits, along with left-hand limits, introduce students to the idea that limits can exist even when a function is not defined at a point, broadening their understanding of mathematical analysis.
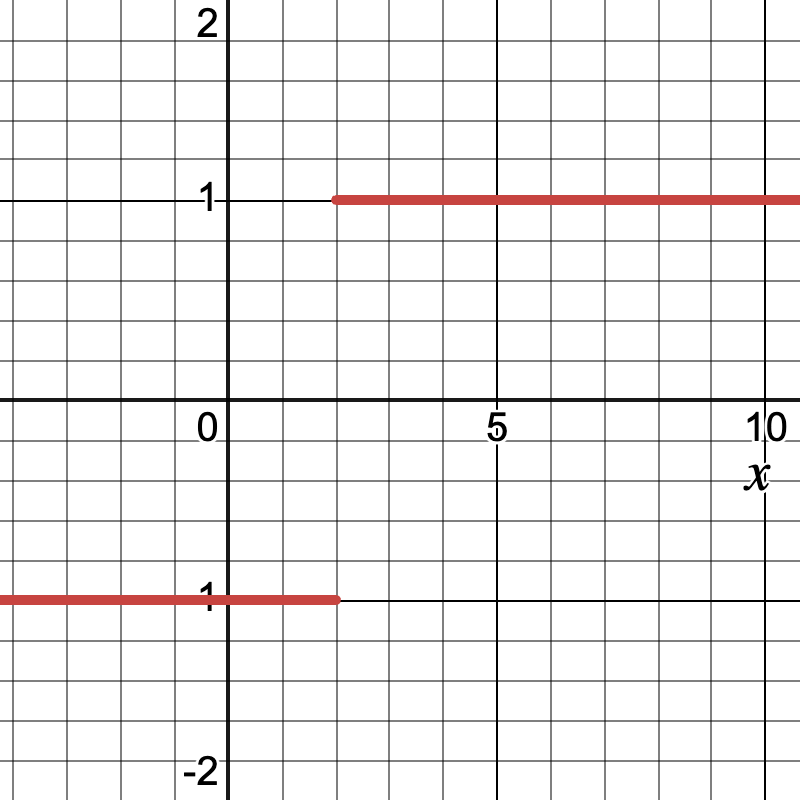
Teacher's Script: "Let's consider the function f(x) = (x - 2) / |x - 2|. What happens as x approaches 2 from the right? We can see that as x gets closer and closer to 2 (but stays greater than 2), f(x) approaches 1. This is the right-hand limit. How does this compare to the left-hand limit? What does this tell us about the continuity of the function at x = 2? Can you think of a real-world scenario where understanding what happens 'just after' a critical point might be important?"
For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |