Display Title
Definition--Calculus Topics--Tangent to a Curve
Display Title
Definition--Calculus Topics--Tangent to a Curve
Topic
Calculus
Definition
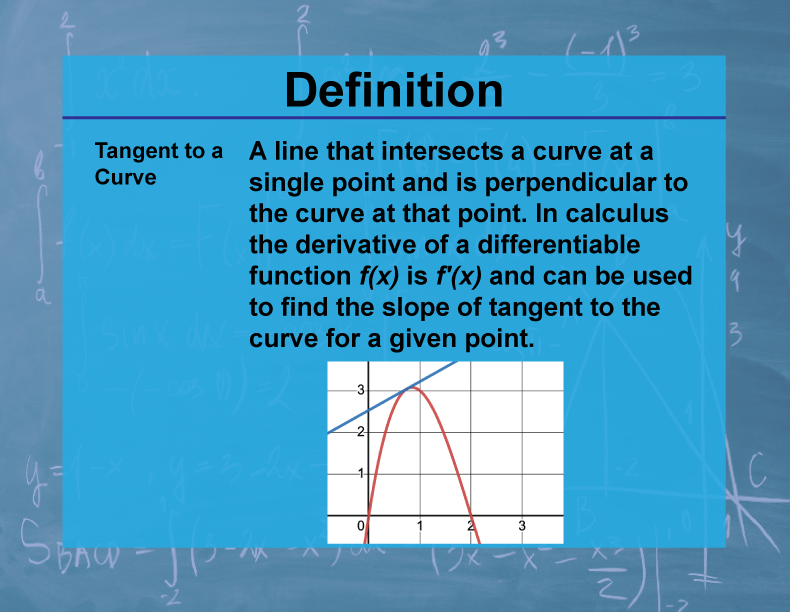
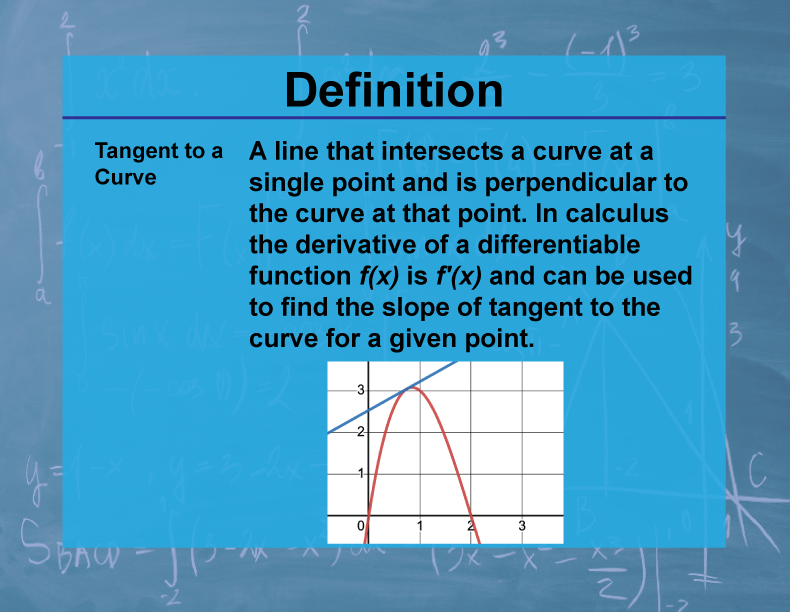
A tangent to a curve at a point is a straight line that touches the curve at that point and has the same slope as the curve at that point. In calculus terms, the slope of the tangent line at a point is equal to the derivative of the function at that point.
Description
The concept of a tangent to a curve is fundamental in calculus, providing a geometric interpretation of the derivative. It represents the instantaneous rate of change of a function at a specific point. Tangent lines are crucial in various applications, including physics (for instantaneous velocity), economics (for marginal analysis), and engineering (for optimization problems).
In mathematics education, the tangent to a curve serves as a bridge between algebra and calculus. It helps students visualize the concept of limits and derivatives, making the transition from average rate of change to instantaneous rate of change more intuitive. Understanding tangent lines is essential for grasping more advanced calculus concepts, such as linear approximation and Taylor series.
Teacher's Script: "Imagine you're on a roller coaster. At any point on the track, the direction you're moving is along the tangent line to the curve of the track. Let's consider the function f(x) = x2. How would we find the equation of the tangent line at x = 2? First, we calculate f'(2) to find the slope. Then we use the point-slope form of a line. How does this tangent line relate to the function near x = 2? How could we use this to approximate f(2.1)? This is the essence of linear approximation in calculus."
For a complete collection of terms related to Calculus click on this link: Calculus Vocabulary Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |