Definition | Circle Concepts | Circle

This is part of a collection of definitions related to circles and related topics. This includes general definitions for parts of a circle, constructions involving circles, and other topics.

—CLICK PREVIEW TO SEE THE DEFINITION—

To see the complete collection of definitions on this topic, click on this link.

The next section provides additional background information on circles and related topics. Use this material to supplement the definitions.

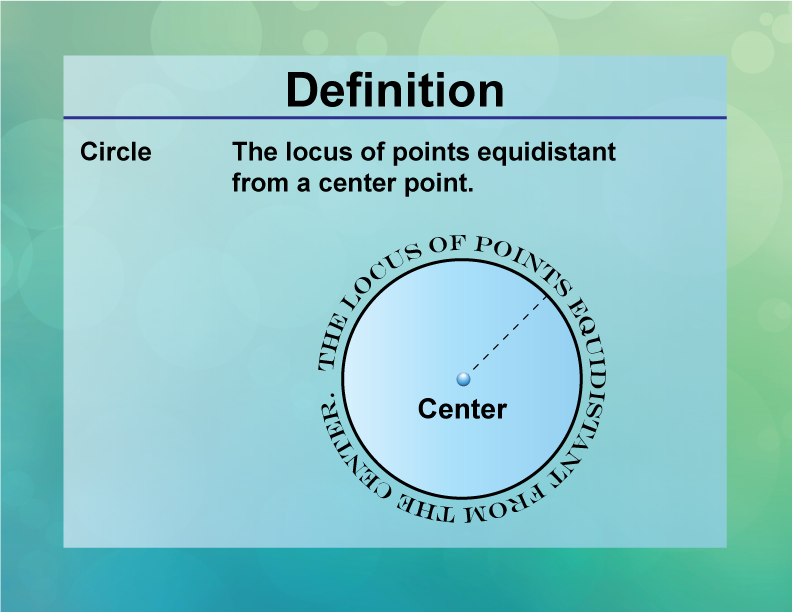
A circle is the locus of points equidistant from a given point, called the center. The "locus of points" are what you see as the circle.

The center is the one point not on the circular form. The distance from the center to any point on the circle is constant. This is part of the "locus of points" definition. The segment from the center to the circle is called the radius.

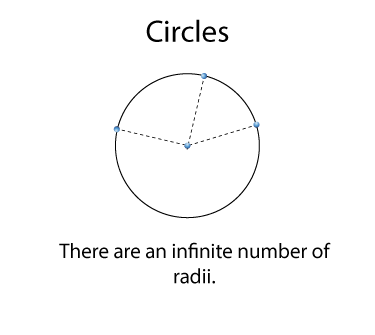
Since there are an infinite number of points that define the circle, then there are also an infinite number of radii (the plural of radius). This is shown below:

By contrast there is only one center point to the circle. A line segment that crosses the center and crosses the center is called a diameter. Think of a diameter as two collinear radii.

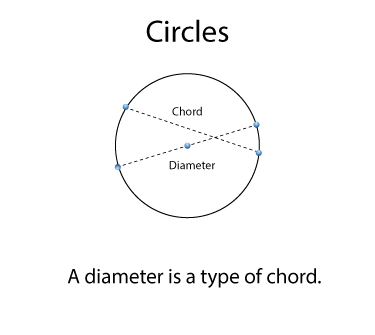
There is also the case of a line segment that intersects the circle at two points but does not cross the center. This is called a chord.

The diameter of a circle is a special type of chord. Do you see how the diameter also meets the definition of a chord?

When a line crosses a circle at two points, then it is a secant. 

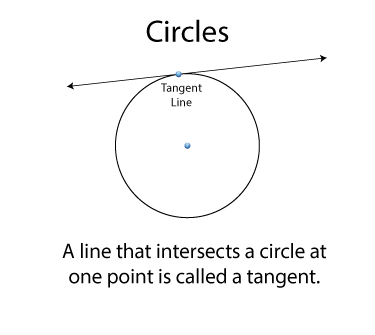
When a line intersects a circle at just one point, then it is called a tangent line. 

A central angle is formed by two radii. The vertex of the angle is at the center of the circle.

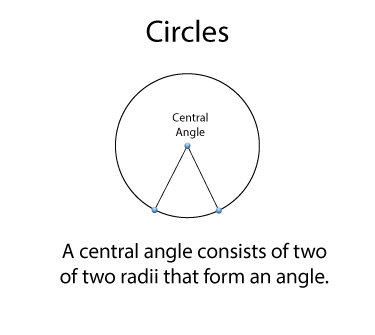
An inscribed angle is formed by two chords that intersect at the circle. Do you see where the vertex of the circle is located?

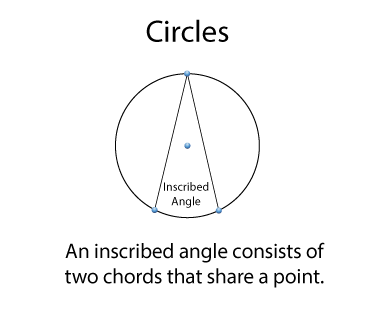
There is an important relationship between inscribed angles and central angles that share two points on the circle. In this case the inscribed angle is half the measurement of the central angle.

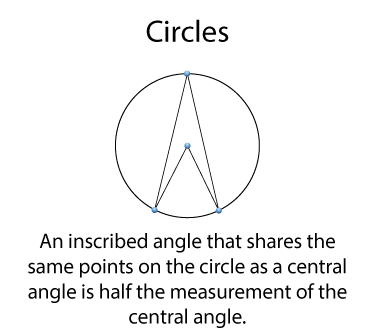
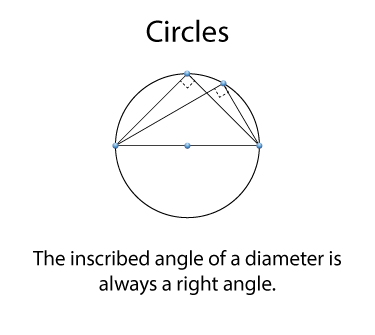
Using what we know about inscribed angles and diameters, then any inscribed angle of a diameter is a right angle. To see this, think of the diameter as a central angle of 180°.

Finally, a line tangent to a circle is perpendicular to the radius of the circle at that point.

Note: The download is a PNG file.

Related Resources

To see additional resources on this topic, click on the Related Resources tab.

Create a Slide Show

Subscribers can use Slide Show Creator to create a slide show from the complete collection of math definitions on this topic. To see the complete collection of definitions, click on this Link.

To learn more about Slide Show Creator, click on this Link.

Accessibility

This resources can also be used with a screen reader. Follow these steps.

• Click on the Accessibility icon on the upper-right part of the screen.
  

• From the menu, click on the Screen Reader button. Then close the Accessibility menu.
  

• Click on the PREVIEW button on the left and then click on the definition card. The Screen Reader will read the definition.