
Display Title
Definition--Closure Property Topics--Complex Numbers and Closure: Division
Display Title
Complex Numbers and Closure: Division
Topic
Math Properties
Definition
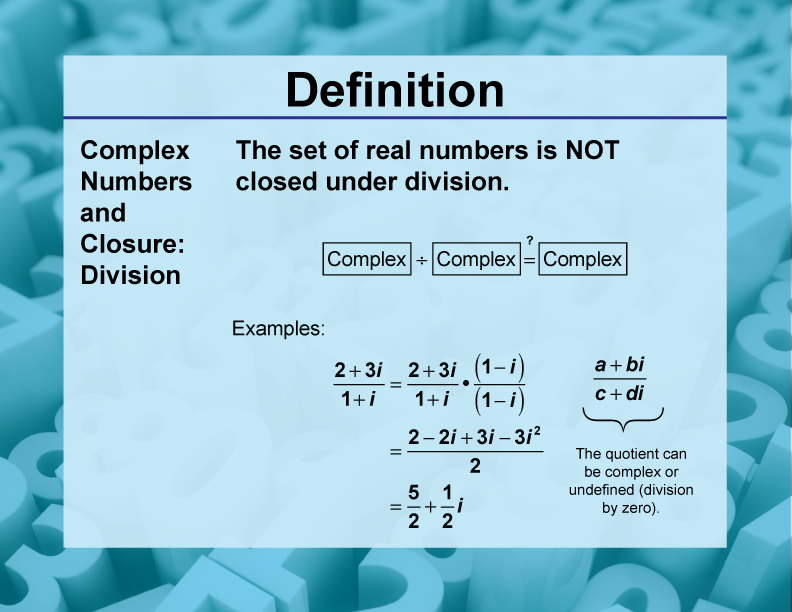
The closure property for division of complex numbers states that the quotient of any two complex numbers, where the divisor is non-zero, is always another complex number. This property ensures that the set of complex numbers is closed under division, maintaining the integrity of the complex number system.
Description
Complex numbers, expressed as a + bi where a and b are real numbers and i is the imaginary unit (√-1), form a crucial mathematical system with extensive applications. The closure property for division of complex numbers is fundamental in establishing the complex number system as a field, which is essential in advanced mathematics and various scientific and engineering disciplines.
Algebraically, we can demonstrate this property as follows: Given two complex numbers z₁ = a + bi and z₂ = c + di (where c and d are not both zero), their quotient is: z₁ / z₂ = (ac + bd) / (c² + d²) + ((bc - ad) / (c² + d²))i This result is always a complex number, proving the closure property for division.
The closure of complex numbers under division has significant real-world applications, particularly in electrical engineering and signal processing. For instance, in the analysis of AC circuits, complex division is used to calculate impedance and transfer functions, which are crucial for understanding circuit behavior and designing filters.
In mathematics education, understanding the closure property for complex number division helps students grasp the completeness of the complex number system. It serves as a bridge to more advanced concepts in complex analysis and provides insight into the structure of mathematical fields.
Teacher's Script: "Let's divide two complex numbers: (3 + 2i) / (1 - i). We multiply both numerator and denominator by the complex conjugate of the denominator: ((3 + 2i)(1 + i)) / ((1 - i)(1 + i)) = (3 + 2i + 3i - 2) / (1 + 1) = (1 + 5i) / 2 = 1/2 + 5i/2 As we can see, the result is another complex number. Can you think of any situation where dividing two complex numbers wouldn't result in a complex number?"
For a complete collection of terms related to the Closure Property click on this link: Closure Property Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSN.RN.B.3, CCSS.MATH.CONTENT.HSN.CN.A.2 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • The Language of Math • Numerical Expressions |
| Copyright Year | 2021 |
| Keywords | Closure Property |