
Display Title
Definition--Closure Property Topics--Even Numbers and Closure: Addition
Display Title
Even Numbers and Closure: Addition
Topic
Math Properties
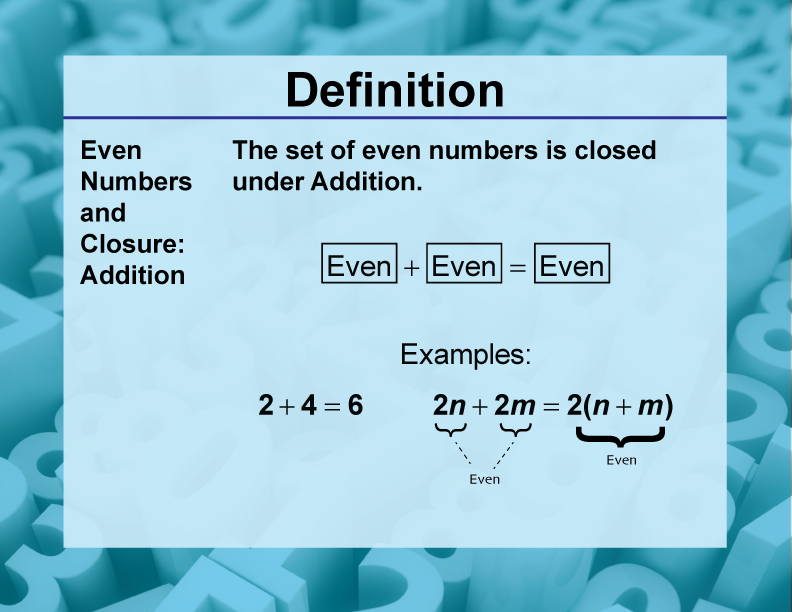
Definition
The closure property for addition of even numbers states that the sum of any two even numbers is always an even number.
Description
The closure property for addition of even numbers is a fundamental concept in number theory and algebra. It demonstrates that the set of even numbers is closed under the operation of addition, meaning that when you add any two even numbers, the result will always be an even number.
This property is crucial for understanding the behavior of even numbers and their relationships. It helps students recognize patterns and make predictions about mathematical operations involving even numbers. In real-world applications, this concept is used in computer programming, particularly in algorithms dealing with parity checks and data structures.
Understanding closure properties like this one is essential for developing a strong foundation in abstract algebraic thinking. It prepares students for more advanced mathematical concepts and helps them appreciate the structure and consistency within mathematical systems.
Teacher's Script: "Let's explore even numbers and addition. If we take two even numbers, say 6 and 8, and add them: 6 + 8 = 14. The result is also an even number! Can you think of any two even numbers where their sum isn't even? Try a few examples and see what you discover!"
For a complete collection of terms related to the Closure Property click on this link: Closure Property Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSN.RN.B.3, CCSS.MATH.CONTENT.HSN.CN.A.2 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • The Language of Math • Numerical Expressions |
| Copyright Year | 2021 |
| Keywords | Closure Property |