Display Title
Definition--Closure Property Topics--Odd Numbers and Closure: Subtraction
Display Title
Odd Numbers and Closure: Subtraction
Topic
Math Properties
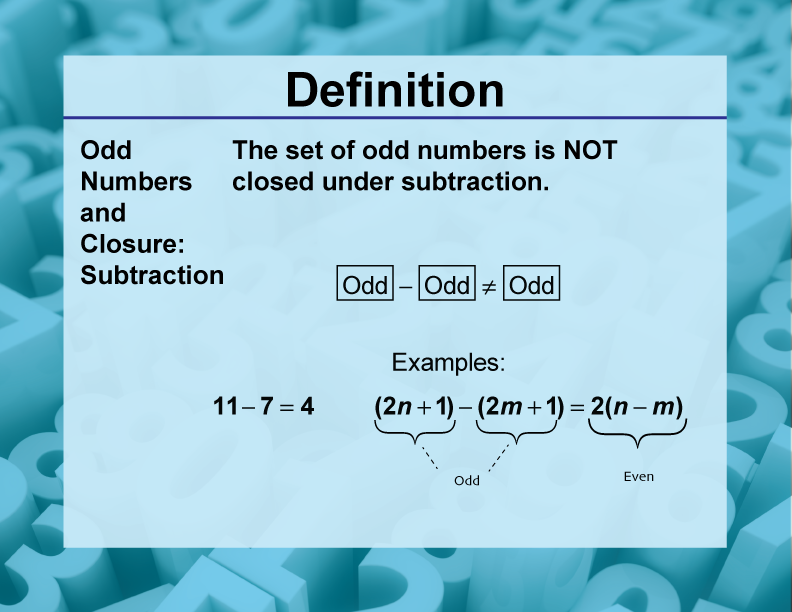
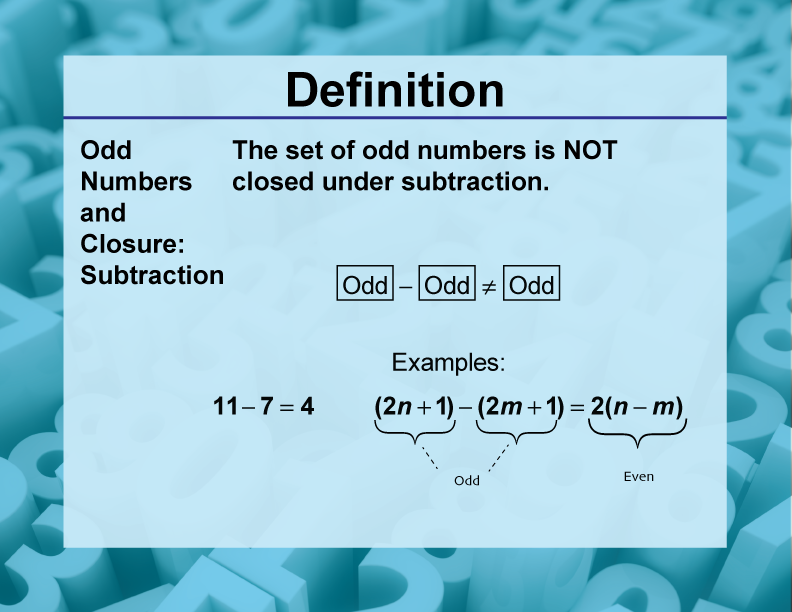
Definition
The set of odd numbers is not closed under subtraction, meaning that the difference between two odd numbers is always an even number, not an odd number.
Description
The concept of closure for odd numbers under subtraction is an important property in number theory. It demonstrates that while odd numbers have many interesting properties, they do not form a closed system under subtraction. This property helps students understand the relationships between odd and even numbers and introduces them to the concept of parity.
Understanding this property is crucial for students as they develop their skills in number theory and algebraic thinking. It provides insights into the behavior of numbers and helps in solving various mathematical problems, including those in computer science and cryptography.
Algebraically, we can express this property as: For any odd integers a and b, a - b = c, where c is always an even integer. This concept helps students transition to more advanced mathematical thinking and prepares them for concepts in abstract algebra and number theory.
Teacher's Script: "Let's subtract two odd numbers: 9 and 5. We get: 9 - 5 = 4. Notice how our result is an even number? Now, let's try 11 and 7: 11 - 7 = 4. Again, we get an even number! Can you explain why the difference between two odd numbers is always even? Try different combinations of odd numbers and see if you can find a pattern."
For a complete collection of terms related to the Closure Property click on this link: Closure Property Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSN.RN.B.3, CCSS.MATH.CONTENT.HSN.CN.A.2 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • The Language of Math • Numerical Expressions |
| Copyright Year | 2021 |
| Keywords | Closure Property |