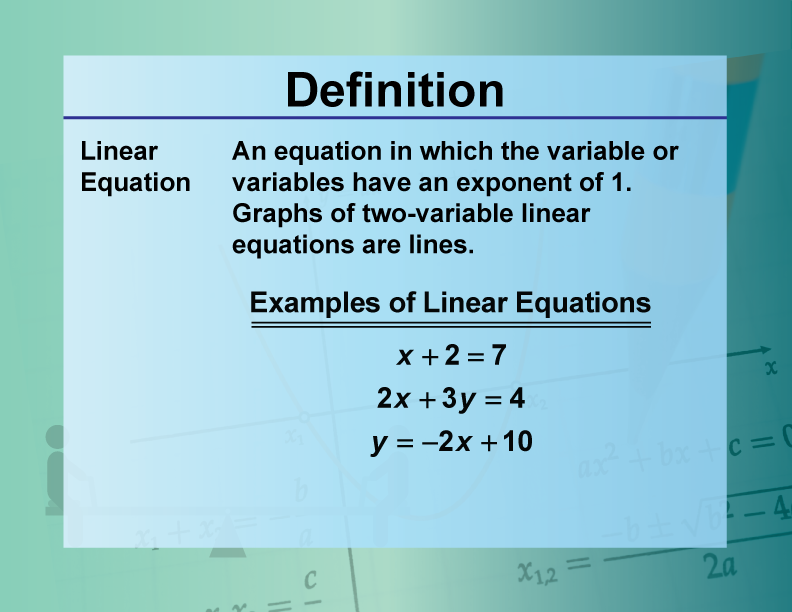
Definition--Equation Concepts--Linear Equation
Register to SaveSubscribe to DownloadPreview Add to Slideshow
(Subscribers Only)

If you are a subscriber, please log in.

Algebra >> Linear Functions and Equations >> Applications of Linear Functions

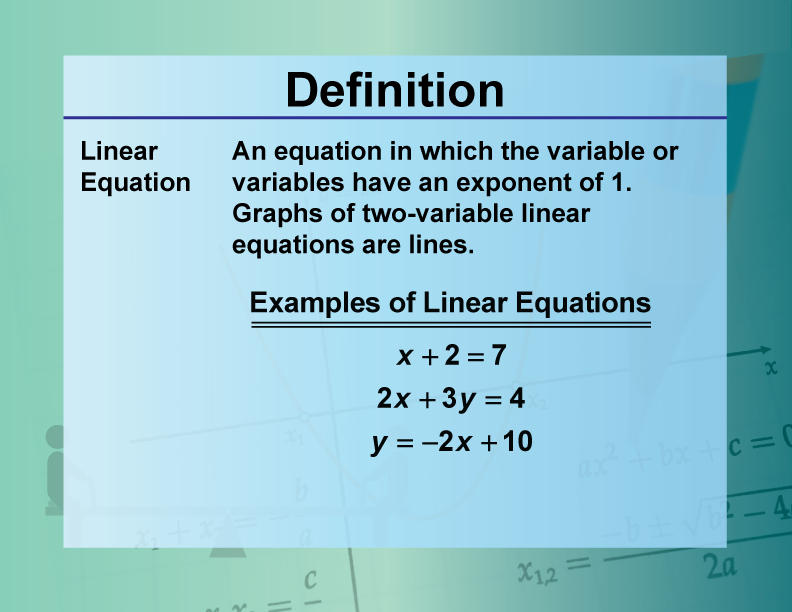
Definition--Equation Concepts--Linear Equation

Linear Equation

Linear Equation

Topic

Equations

Definition

A linear equation is an equation that does not have an variables raised to a power higher than one. A linear equation can have one or more variables.

Description

Linear equations are fundamental in algebra and describe relationships are summarized below. 

ax + b = 0Result is a single point on a number line
ax + by = cResult can be an infinite number of (x, y) coordinates that form a straight line

In real-world applications, linear equations are used to model relationships in economics, physics, and engineering. Understanding linear equations helps students analyze data, make predictions, and solve practical problems.

For a complete collection of terms related to functions and relations click on this link: Functions and Relations Collection

The following section provides additional information on solving one- and two-step equations.

Solving One-Step Equations

A one-step equation can literally be solved in one step. This is because the equation is written in a form such that an inverse operation is enough to solve it. Here are the different forms a one-step equation can take:

Equation TypeInverse OperationExample
AdditionSubtraction\begin{array}{l} x + 1 = 3\\ x + 1 - 1 = 3 - 1 \leftarrow {\rm{Inverse operation}}\\ x = 2 \end{array}
SubtractionAddition\begin{array}{l} x - 1 = 3\\ x - 1 + 1 = 3 + 1 \leftarrow {\rm{Inverse operation}}\\ x = 4 \end{array}
MultiplicationDivision\begin{array}{l} 2x = 4\\ \frac{{2x}}{2} = \frac{4}{2} \leftarrow {\rm{Inverse operation}}\\ x = 2 \end{array}
DivisionMultiplication\begin{array}{l} \frac{x}{2} = 3\\ \frac{x}{2} \bullet 2 = 3 \bullet 2\\ x = 6 \end{array}

 

With these four basic cases, there are a number of variations, depending on the numbers involved. The simplest types of these equations involve positive whole numbers. But these equations can involve integers and rational numbers.

 

The general form of each of the four types of basic one-step equations are summarized here.

Solving Two-Step Equations

You saw that with one-step equations, the “one step” involved one inverse operation. With two-step equations, there are two inverse operations involved in solving the equation. But this extra step introduces many more types of equations to solve, beyond the basic four from one-step equations.

 

There are 16 basic types of two-step equations that involve different combinations of the four basic operations. 

 AdditionSubtractionMultiplicationDivision
AdditionAAASAMAD
SubtractionSASSSMSD
MultiplicationMAMSMMMD
DivisionDADSDMDD

 

 

These 16 basic examples are summarized in the table below, where we show an example of such an equation using numbers, then followed by a general form of the equation using variables and constant terms.

 

Equation TypeInverse OperationsExampleGeneral Form
Addition and AdditionSubtraction and Subtraction2x + 3 = x + 4ax + b = (a - 1)x + c
Addition and SubtractionSubtraction and Addition3x + 4 = 2x - 5ax + b = (a - 1)x - c
Addition and MultiplicationSubtraction and Division2x + 4 = 8ax + b = c
Addition and DivisionSubtraction and Multiplication\frac{x}{2} + 4 = 8\frac{x}{a} + b = c
Subtraction and AdditionAddition and Subtraction2x - 3 = x + 4ax - b = (a - 1)x + c
Subtraction and SubtractionAddition and Addition2x - 3 = x - 4ax - b = (a - 1)x - c
Subtraction and MultiplicationAddition and Division5x - 7 = 18ax - b = c
Subtraction and DivisionAddition and Multiplication\frac{x}{2} - 4 = 8\frac{x}{a} - b = c
Multiplication and AdditionDivision and Subtraction3(x + 2) = 12a(x + b) = c
Multiplication and SubtractionDivision and Addition3(x - 2) = 15a(x - b) = c
Multiplication and MultiplicationDivision and Division14(15x) = 280a(bx) = c
Multiplication and DivisionDivision and Multiplication12(\frac{x}{5}) = 144a(\frac{x}{b}) = c
Division and AdditionMultiplication and Subtraction\frac{{x + 4}}{5} = 12\frac{{x + a}}{b} = c
Division and SubtractionMultiplication and Addition\frac{{x - 4}}{5} = 12\frac{{x - a}}{b} = c
Division and MultiplicationMultiplication and Division\frac{2}{3}x = 50\frac{a}{b}x = c
Division and DivisionMultiplication and Multiplication\frac{{\frac{x}{4}}}{5} = 30\frac{{\frac{x}{a}}}{b} = c

 

 

These 16 basic two-step equations come in different forms depending on the sign of the numbers and whether the numbers are integers or rational numbers. The simplest types of these equations involve positive whole numbers.

 

Equation TypeGeneral Form
Additionx + a = b,{\rm{ for real numbers }}a{\rm{ and }}b
Subtractionx - a = b,{\rm{ for real numbers }}a{\rm{ and }}b
Multiplicationax = b,{\rm{ for real numbers }}a{\rm{ and }}b,{\rm{ }}a \ne 0
Division\frac{x}{a} = b,{\rm{ for real numbers }}a{\rm{ and }}b,{\rm{ }}a \ne 0
Common Core Standards CCSS.MATH.CONTENT.6.EE.B.5, CCSS.MATH.CONTENT.7.EE.B.4, CCSS.MATH.CONTENT.HSA.REI.A.1
Grade Range 6 - 12
Curriculum Nodes Algebra
    • Linear Functions and Equations
        • Applications of Linear Functions
Copyright Year 2021
Keywords equations, solving equations, definitions, glossary terms