Display Title
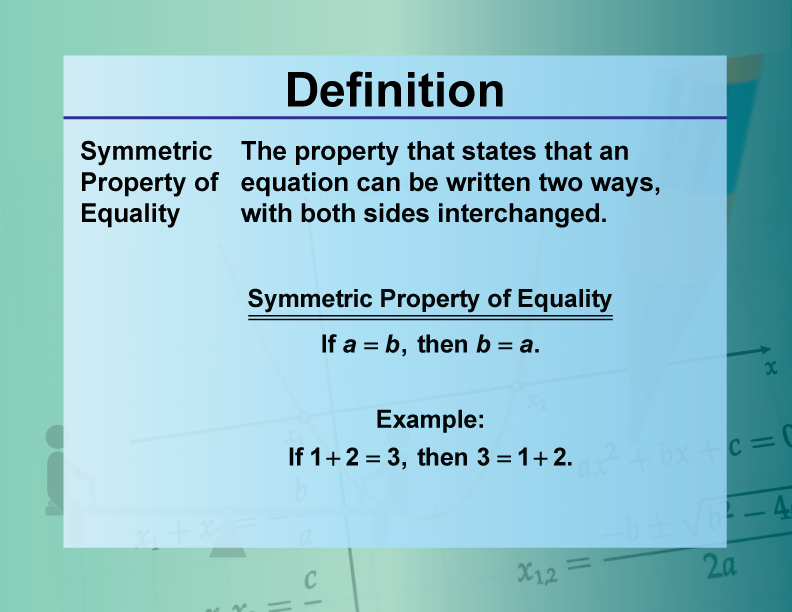
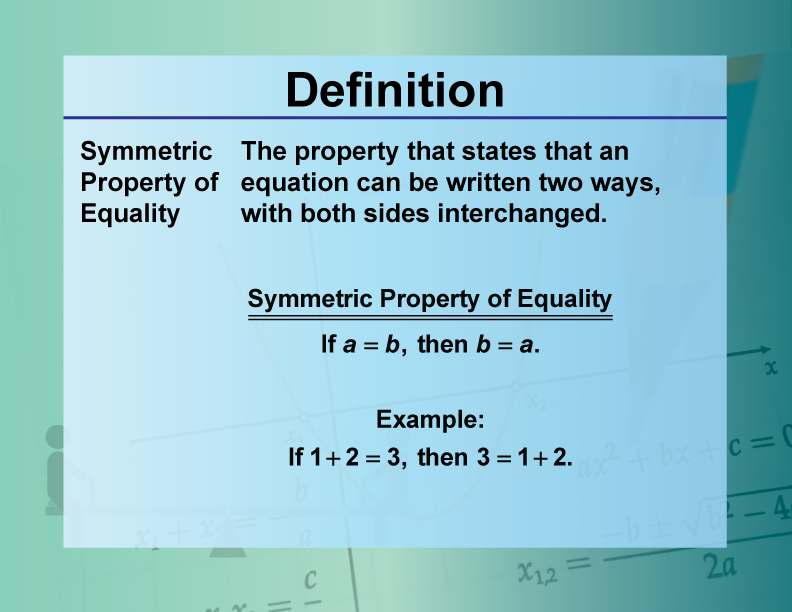
Definition--Equation Concepts--Symmetric Property of Equality
Display Title
Symmetric Property of Equality
Topic
Equations
Definition
The Symmetric Property of Equality states that if a = b, then b = a.
Description
The Symmetric Property of Equality is a basic principle in mathematics. It asserts that the equality relation is symmetric, meaning that if one quantity equals another, then the second quantity equals the first. For example, if
x = y
then
y = x
This property is used to justify steps in solving equations and proving mathematical statements.
In real-world applications, the symmetric property underlies the concept of equality and is fundamental in logical reasoning and proofs. Understanding this property helps students build a strong foundation in algebra and develop rigorous mathematical arguments.
For a complete collection of terms related to functions and relations click on this link: Functions and Relations Collection
| Common Core Standards | CCSS.MATH.CONTENT.6.EE.B.5, CCSS.MATH.CONTENT.7.EE.B.4, CCSS.MATH.CONTENT.HSA.REI.A.1 |
|---|---|
| Grade Range | 6 - 12 |
| Curriculum Nodes |
Algebra • Expressions, Equations, and Inequalities • Applications of Equations and Inequalities |
| Copyright Year | 2021 |
| Keywords | equations, solving equations, definitions, glossary terms |