Display Title
Definition--Fraction Concepts--Least Common Multiple (LCM)
Display Title
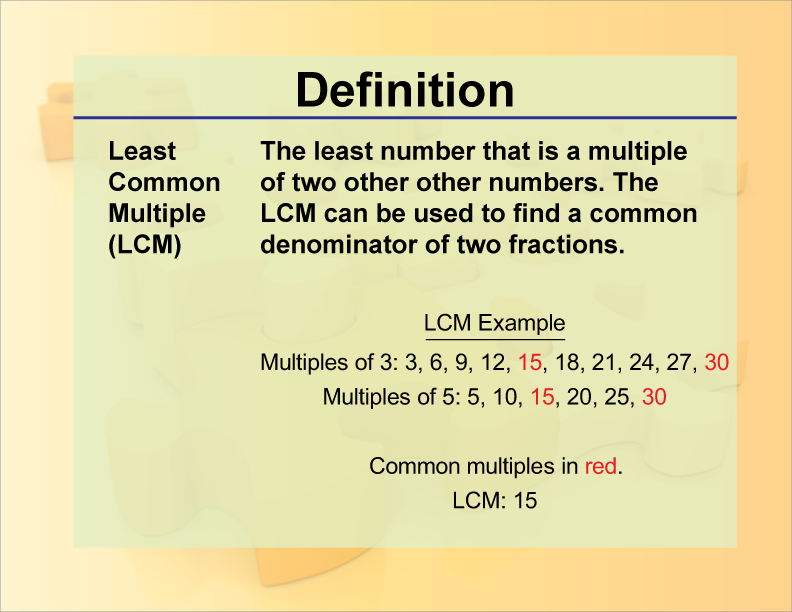
Least Common Multiple (LCM)

Topic
Fractions
Definition
The Least Common Multiple (LCM) of two or more numbers is the smallest number that is a multiple of each of the numbers.
Description
The concept of the Least Common Multiple (LCM) is fundamental in the study of fractions. It is particularly useful when adding, subtracting, or comparing fractions. The LCM helps in finding a common denominator, which is essential for performing these operations with fractions.
For instance, consider the fractions 1/4 and 1/6. To add these fractions, one must first find a common denominator. The denominators here are 4 and 6. The LCM of 4 and 6 is 12, which means 12 is the smallest number that both 4 and 6 can divide into without leaving a remainder. By converting the fractions to have this common denominator, they become 3/12 and 2/12, respectively. This makes it straightforward to add them:
3/12 + 2/12 = 5/12.
Understanding the LCM is not only crucial for arithmetic operations involving fractions but also for solving problems involving ratios, proportions, and algebraic expressions. It helps in simplifying complex fraction problems and ensures accuracy in calculations. Mastery of the LCM concept enhances a student's ability to handle more advanced mathematical concepts and operations.
For a complete collection of terms related to fractions click on this link: Fractions Collection.
| Common Core Standards | CCSS.MATH.CONTENT.6.NS.B.4 |
|---|---|
| Grade Range | 3 - 6 |
| Curriculum Nodes |
Arithmetic • Fractions • Fractions and Mixed Numbers |
| Copyright Year | 2013 |
| Keywords | fraction, LCM, least common multiple, definitions, glossary term |