Display Title
Definition--Functions and Relations Concepts--Absolute Value Function
Display Title
Absolute Value Function

Topic
Functions and Relations
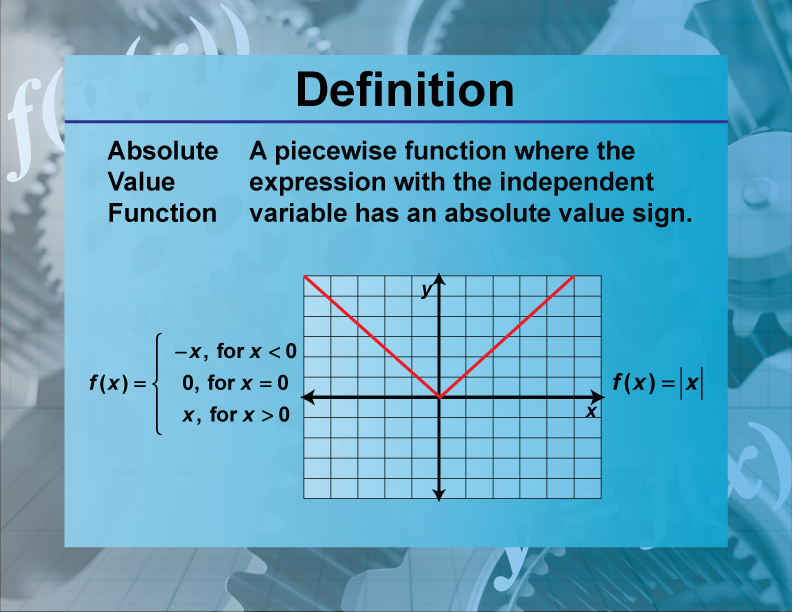
Definition
An absolute value function is a function that contains an algebraic expression within absolute value symbols. It is defined as
f(x)=|x|
where |x| denotes the absolute value ofx.
Description
The absolute value function is significant in mathematics because it measures the distance of a number from zero on the number line, regardless of direction. This function is linear in nature, as it can be broken into two linear pieces: one for
x≥0
and one for
x<0
In real-world applications, absolute value functions are used to describe situations where only the magnitude of a quantity matters, such as in distance, error measurement, and economics.
For example, if you want to calculate the deviation of a value from a target, you would use the absolute value function to ensure the deviation is always positive. Mathematically, this can be represented as
|x−target|
Understanding absolute value is crucial for students as it lays the groundwork for more advanced topics in algebra and calculus. It helps in solving equations and inequalities that involve absolute values and in understanding piecewise functions.
For a complete collection of terms related to linear functions and equations click on this link: Functions and Relations Collection
| Common Core Standards | CCSS.MATH.CONTENT.8.F.A.1, CCSS.MATH.CONTENT.8.F.B.5, CCSS.MATH.CONTENT.HSF.IF.A.1, CCSS.MATH.CONTENT.HSF.IF.A.2, CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1, CCSS.MATH.CONTENT.HSF.BF.B.3, CCSS.MATH.CONTENT.HSF.BF.B.4 |
|---|---|
| Grade Range | 6 - 9 |
| Curriculum Nodes |
Algebra • Functions and Relations • Special Functions |
| Copyright Year | 2021 |
| Keywords | definition, function, relations, glossary terms |