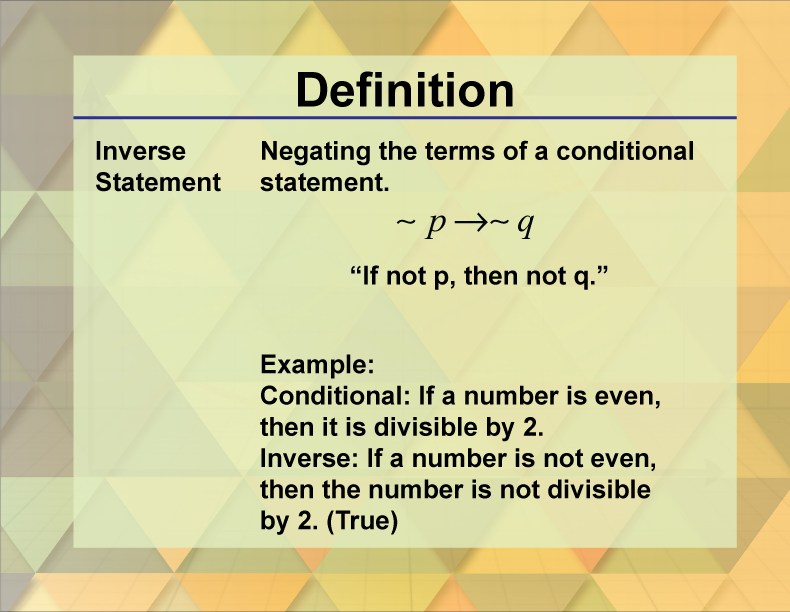
Definition--Geometry Basics--Inverse Statement
Register to SaveSubscribe to DownloadPreview Add to Slideshow
(Subscribers Only)

If you are a subscriber, please log in.

Geometry >> Points and Lines >> Definition of a Point

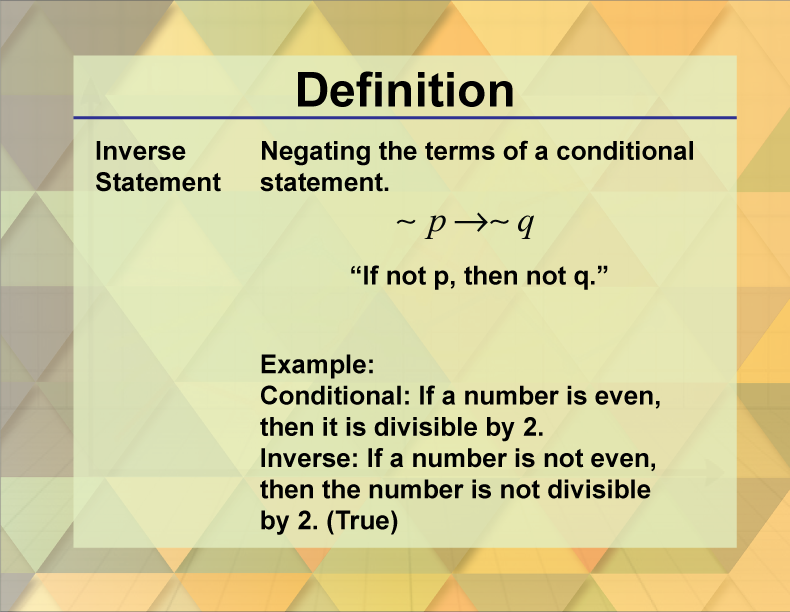
Definition--Geometry Basics--Inverse Statement

Inverse Statement

Inverse Statement

Topic

Geometry Basics

Definition

The inverse statement negates both the hypothesis and conclusion of a conditional statement.

Description

Understanding inverse statements is essential for grasping logical reasoning and transformations in mathematical proofs. For example, if we start with the statement "If A, then B," the inverse would state: "If not A, then not B." Developing this understanding aids in examining relationships between different types of logical statements and reinforces critical thinking skills necessary for advanced mathematics education by facilitating deeper comprehension of conditional logic across various scenarios.

Inverse Statement
When it rains, the streets are wet. 
Is the inverse true?

For a complete collection of terms related to Geometry Basics click on this link: Geometry Basics Collection

Common Core Standards CCSS.MATH.CONTENT.HSG.CO.A.1
Grade Range 8 - 10
Curriculum Nodes Geometry
    • Points and Lines
        • Definition of a Point
Copyright Year 2020
Keywords logic