Display Title
Definition--Linear Function Concepts--Constant Function
Display Title
Constant Function

Topic
Linear Functions
Definition
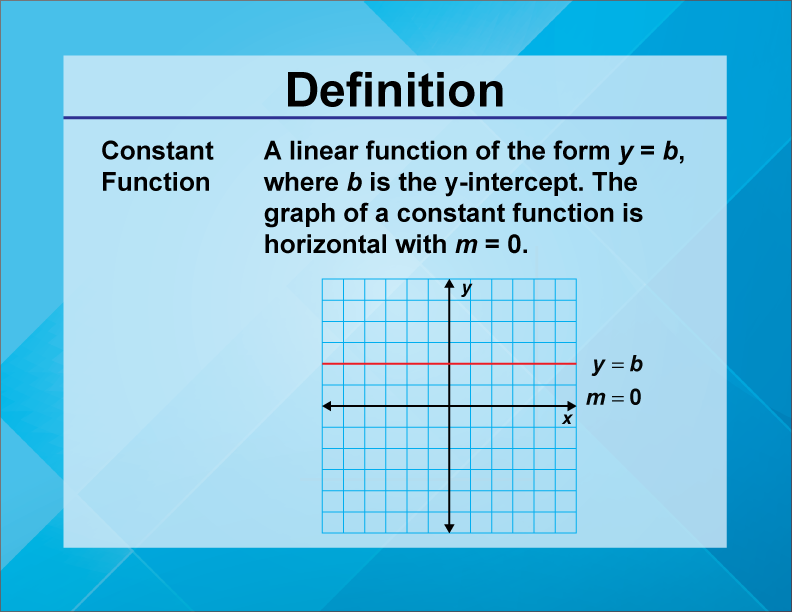
A constant function is a linear function of the form f(x) = b, where b is a constant. The graph of a constant function is a horizontal line.
Description
Constant functions are a fundamental concept in linear functions. They represent scenarios where the output value remains unchanged, regardless of the input value. This is depicted graphically as a horizontal line, indicating that the function's rate of change is zero.
In real-world applications, constant functions can model situations where a quantity remains steady over time. For example, a flat fee service charge that does not vary with usage can be represented as a constant function.
In mathematics, understanding constant functions is crucial as they form the basis for more complex linear functions. They help students grasp the concept of slope, which in this case is zero, and understand how different linear functions behave.
For a complete collection of terms related to linear functions and equations click on this link: Linear Functions and Equations Collection
| Common Core Standards | CCSS.MATH.CONTENT.8.SP.A.1, CCSS.MATH.CONTENT.HSA.SSE.A.1.A |
|---|---|
| Grade Range | 6 - 9 |
| Curriculum Nodes |
Algebra • Linear Functions and Equations • Slope-Intercept Form |
| Copyright Year | 2021 |
| Keywords | linear functions, definitions, glossary term |