Display Title
Definition--Linear Function Concepts--Identity Function
Display Title
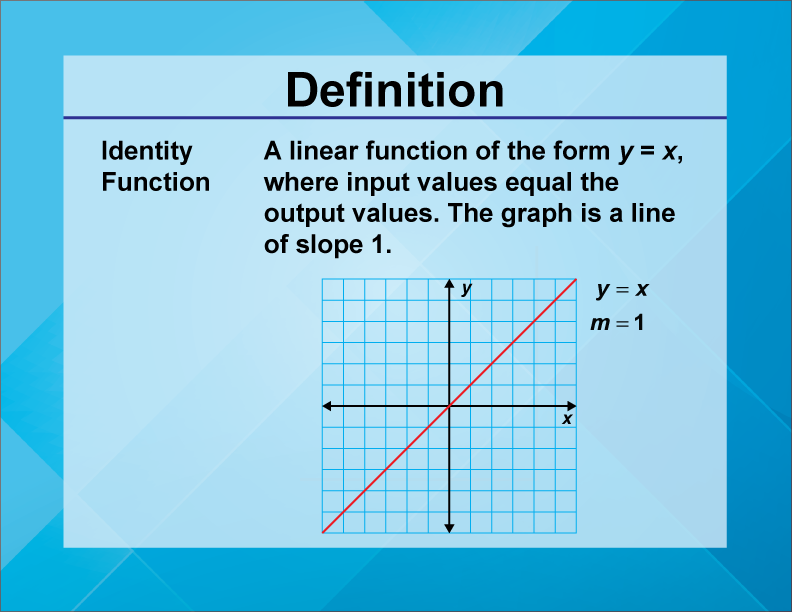
Identity Function

Topic
Linear Functions
Definition
An identity function is a linear function of the form f(x) = x, where the output is equal to the input for all values of x.
Description
The identity function is a basic yet crucial concept in linear functions. It represents a scenario where the input value is always equal to the output value, graphically depicted as a 45-degree line passing through the origin.
In real-world applications, the identity function can model situations where input and output are directly proportional and identical, such as converting units of the same measure. This also introduces the concept of identity, which is fundamental to mathematics.
In education, the identity function helps students understand the concept of linearity and proportionality. It serves as a foundation for more complex functions and is essential for grasping the behavior of linear equations. The identity function is also used as the baseline for later understanding of inverse functions.
For a complete collection of terms related to linear functions and equations click on this link: Linear Functions and Equations Collection
| Common Core Standards | CCSS.MATH.CONTENT.8.SP.A.1, CCSS.MATH.CONTENT.HSA.SSE.A.1.A |
|---|---|
| Grade Range | 6 - 9 |
| Curriculum Nodes |
Algebra • Linear Functions and Equations • Slope-Intercept Form |
| Copyright Year | 2021 |
| Keywords | linear functions, definitions, glossary term |