Display Title
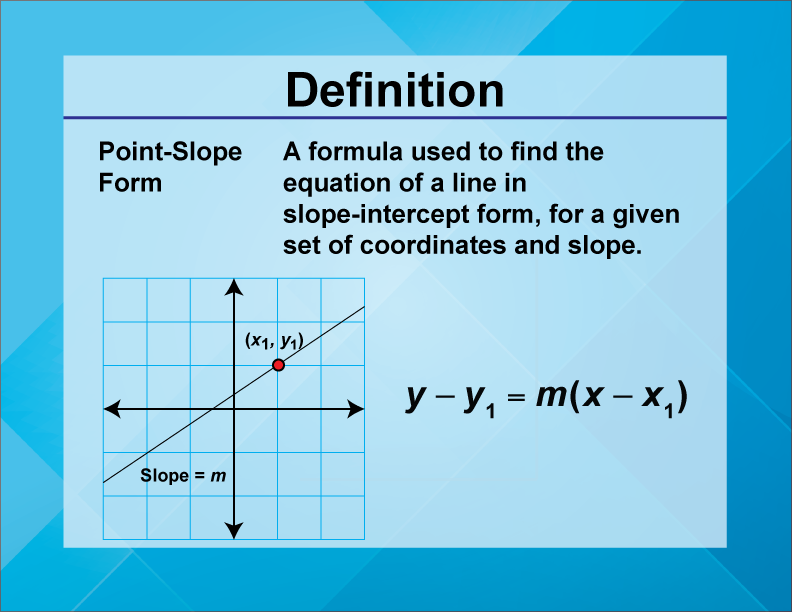
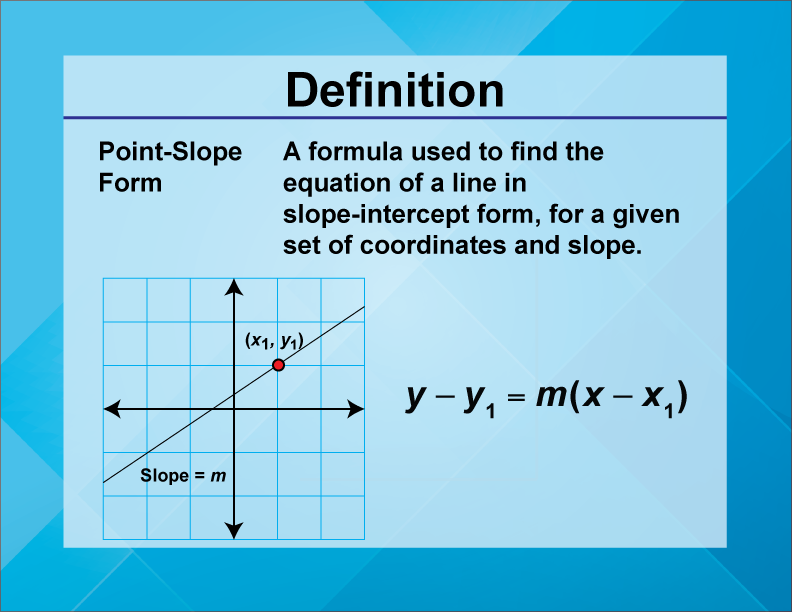
Definition--Linear Function Concepts--Point-Slope Form
Display Title
Point-Slope Form
Topic
Linear Functions
Definition
Point-slope form of a linear equation is written as y − y1 = m(x−x1 ), where m is the slope and (x1 ,y1) is a point on the line.
Description
The point-slope form is a versatile way to express linear equations, especially useful when you know a point on the line and the slope. It allows for quick construction of the equation of a line.
In real-world applications, point-slope form is used in fields like physics and engineering to model linear relationships when specific data points and rates of change are known. For instance, it can be used to determine the equation of a trajectory in motion analysis.
In education, understanding point-slope form helps students grasp how linear equations can be derived from different types of information. It reinforces the concept of slope and its importance in defining the direction and steepness of a line.
For a complete collection of terms related to linear functions and equations click on this link: Linear Functions and Equations Collection
Watch this video to learn about point-slope form. (The transcript is also available.)
Video Transcript
You’ve seen how a linear function in slope-intercept form is written: y = m*x + b, where m is the slope and b is the y intercept.
So, if you’re given the slope and y-intercept for a linear function, you can graph it.
For example, suppose a linear function has a slope of 2 and a y-intercept of 1.
Let’s graph it.
Start with the y-intercept.
Here is the point (0, 1).
Now use the slope information.
A slope of 2 means you count up 2 spaces, and then 1 to the right.
This is a new point that is also on the line.
Connect the two points with a line, and you’ve constructed the linear function with the given slope and y-intercept.
Now suppose you have a point that is on the graph of the line, and the slope.
How can you find the equation of the line through this point with this slope?
Without the y-intercept, we can’t do what you did in the previous example.
But you do have the coordinates to the point on the line.
How can you use these coordinates to find the equation?
Use the point-slope form, which is shown here.
The equation is y minus y-one equals m times the quantity in parentheses, x minus x-one.
Plug in the coordinates into this formula to find the equation in slope-intercept form.
Let’s look at an example.
Suppose a line with a slope of 3 crosses this point: (2,4).
What is the equation of the line?
Input the coordinates into the equation as shown.
Solve the equation y.
As you can see the equation of the line, in slope-intercept form is y = 3x - 2.
You can see how the y-intercept of the graph is -2.
The point-slope form is useful when you have a specific point that needs to intersect a line.
Applications of this include targeting systems that need to reach a preselected destination.
| Common Core Standards | CCSS.MATH.CONTENT.8.SP.A.1, CCSS.MATH.CONTENT.HSA.SSE.A.1.A |
|---|---|
| Grade Range | 6 - 9 |
| Curriculum Nodes |
Algebra • Linear Functions and Equations • Point-Slope Form |
| Copyright Year | 2021 |
| Keywords | linear functions, definitions, glossary term, point-slope form |