Display Title
Definition--Polygon Concepts--Incircle
Display Title
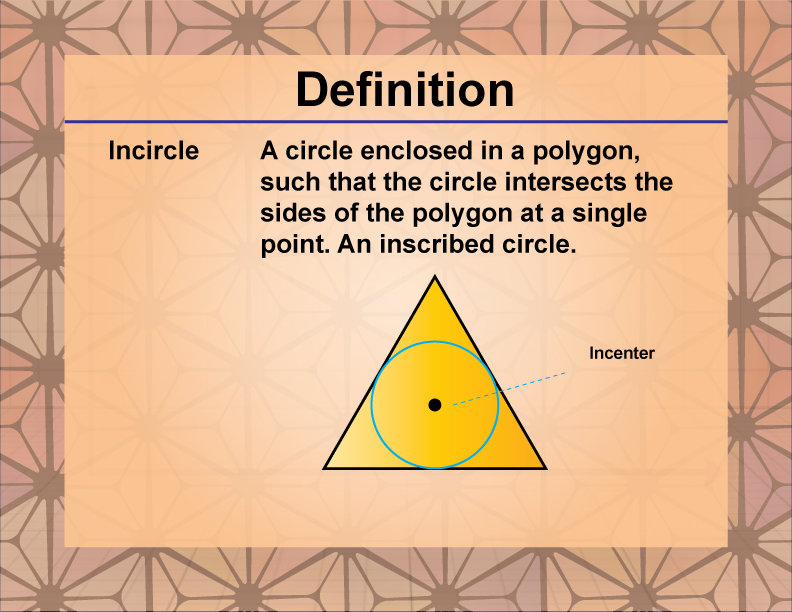
Incircle

Topic
Polygon Concepts
Definition
An incircle is the largest circle that can be inscribed within a polygon, tangent to all of its sides.
Description
The incircle is a fundamental concept in geometry, particularly in the study of polygons. It represents the largest circle that can fit perfectly inside a polygon while touching each side at exactly one point. These points of contact are called tangent points. The incircle is especially significant in regular polygons, where it's centered at the polygon's center and all tangent points are equidistant from the center. The incircle's properties are closely related to the polygon's symmetry and area. In triangles, the incircle's radius can be calculated using the semi-perimeter and area, forming the basis for many geometric proofs and problems.
For other polygons, the incircle helps in understanding concepts like symmetry, area optimization, and the relationship between a polygon's sides and its internal space. The study of incircles extends beyond basic geometry into more advanced topics like computational geometry and optimization problems. It's also relevant in practical applications such as efficient packing and design in engineering and architecture. Understanding incircles contributes to a deeper appreciation of polygon properties and their relationships to circles, enhancing overall geometric intuition.
For a complete collection of terms related to polygons click on this link: Polygons Collection.
| Common Core Standards | CCSS.MATH.CONTENT.5.G.B.3, CCSS.MATH.CONTENT.5.G.B.4, CCSS.MATH.CONTENT.3.G.A.1, CCSS.MATH.CONTENT.3.MD.D.8, CCSS.MATH.CONTENT.6.G.A.1, CCSS.MATH.CONTENT.6.G.A.3, CCSS.MATH.CONTENT.HSG.CO.A.3 |
|---|---|
| Grade Range | 3 - 8 |
| Curriculum Nodes |
Geometry • Polygons • Definition of a Polygon |
| Copyright Year | 2021 |
| Keywords | polygon, definitions, glossary term |