Display Title
Definition--Polygon Concepts--Interior Angles of a Polygon
Display Title
Sum of Interior Angles of a Polygon

Topic
Polygons
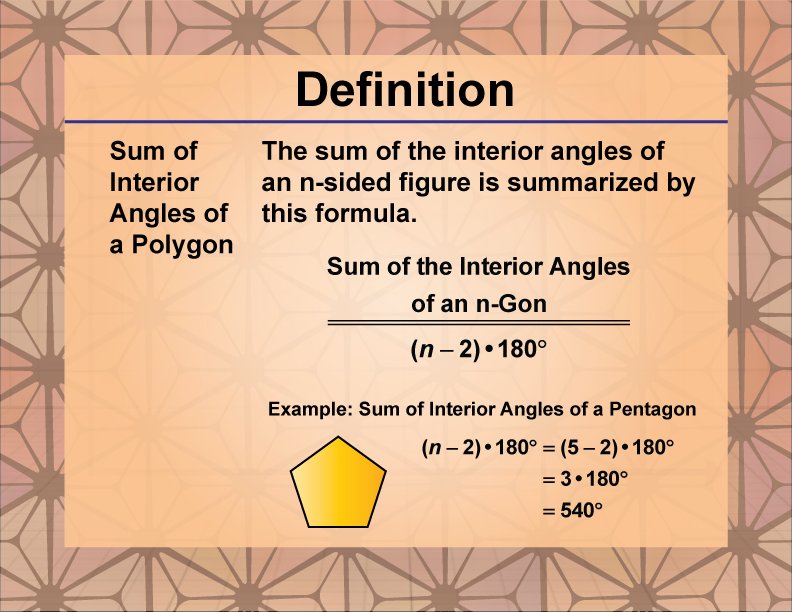
Definition
The sum of interior angles of a polygon with n sides is given by the formula: (n - 2) × 180°, where n is the number of sides in the polygon.
Description
The sum of interior angles is a fundamental property of polygons that plays a crucial role in geometry. This concept is essential for understanding the structure and properties of various polygons, from simple triangles to complex multi-sided figures. The formula (n - 2) × 180° provides a quick and efficient way to calculate the total measure of all interior angles in any polygon, regardless of its regularity.
This property is particularly useful in geometric proofs, problem-solving, and real-world applications involving polygonal shapes. For instance, in a triangle (n = 3), the sum of interior angles is always 180°, while in a quadrilateral (n = 4), it's 360°. As the number of sides increases, so does the sum of the interior angles, following a predictable pattern.
Understanding the sum of interior angles also helps in determining individual angle measures in regular polygons, where all interior angles are congruent. This knowledge is valuable in various fields, including architecture, engineering, and computer graphics, where precise calculations of polygonal structures are often required.
For a complete collection of terms related to polygons click on this link: Polygons Collection.
| Common Core Standards | CCSS.MATH.CONTENT.5.G.B.3, CCSS.MATH.CONTENT.5.G.B.4, CCSS.MATH.CONTENT.3.G.A.1, CCSS.MATH.CONTENT.3.MD.D.8, CCSS.MATH.CONTENT.6.G.A.1, CCSS.MATH.CONTENT.6.G.A.3, CCSS.MATH.CONTENT.HSG.CO.A.3 |
|---|---|
| Grade Range | 3 - 8 |
| Curriculum Nodes |
Geometry • Polygons • Definition of a Polygon |
| Copyright Year | 2021 |
| Keywords | polygon, definitions, glossary term |