Display Title
Definition--Polynomial Concepts--Degree of a Polynomial
Display Title
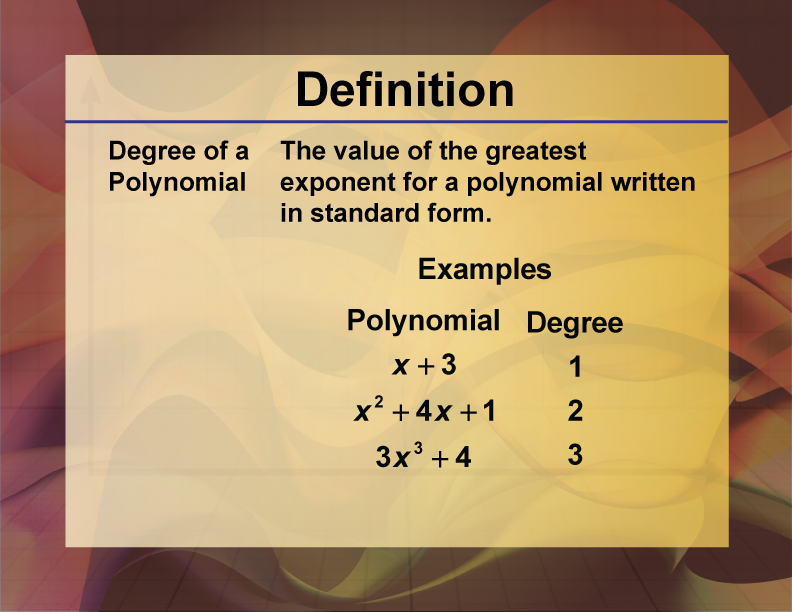
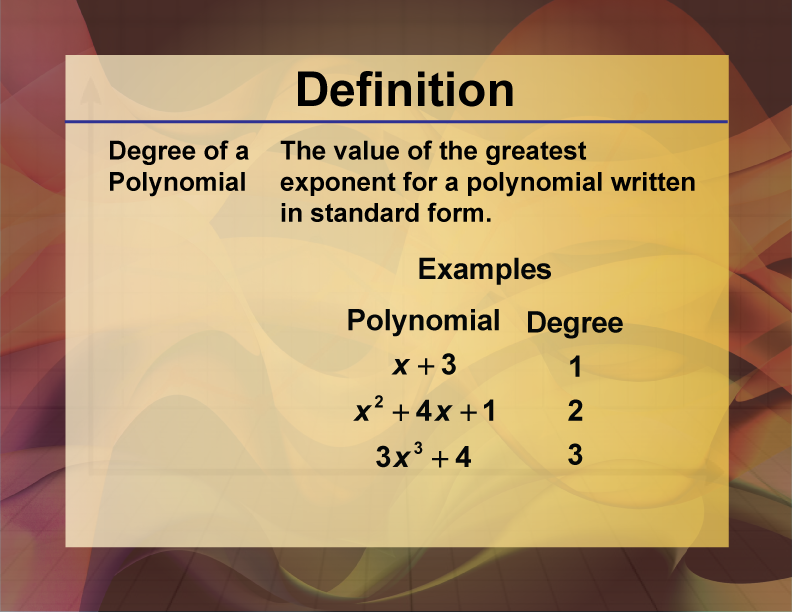
Degree of a Polynomial
Topic
Polynomials
Definition
The degree of a polynomial is the highest power of the variable in the polynomial when it is written in standard form.
Description
The degree of a polynomial is a fundamental characteristic that provides crucial information about the polynomial's behavior and properties. It plays a significant role in understanding the complexity of polynomial functions and is essential in various mathematical analyses and applications. The degree determines the maximum number of roots a polynomial can have, influences the shape of its graph, and affects its behavior as the variable approaches infinity.
In algebraic operations, the degree of a polynomial is vital for determining the result of multiplication or division of polynomials. It's also crucial in solving polynomial equations, as the degree indicates the maximum number of solutions possible. In calculus, the degree of a polynomial is important for understanding limits, derivatives, and integrals. Furthermore, in fields such as computer science and engineering, the degree of polynomials is used in algorithm analysis and signal processing.
For a complete collection of terms related to polynomials click on this link: Polynomials Collection
| Common Core Standards | CCSS.MATH.CONTENT.HSA.APR.A.1, CCSS.MATH.CONTENT.HSA.APR.B.2, CCSS.MATH.CONTENT.HSA.APR.C.5, CCSS.MATH.CONTENT.HSA.APR.C.4, CCSS.MATH.CONTENT.HSA.APR.B.3, CCSS.MATH.CONTENT.HSF.IF.C.7.C |
|---|---|
| Grade Range | 8 - 12 |
| Curriculum Nodes |
Algebra • Polynomials • Polynomial Expressions |
| Copyright Year | 2014 |
| Keywords | polynomials, monomials, definitions, glossary term |