Display Title
Definition--Polynomial Concepts--Difference of Squares
Display Title
Difference of Squares
Topic
Polynomials
Definition
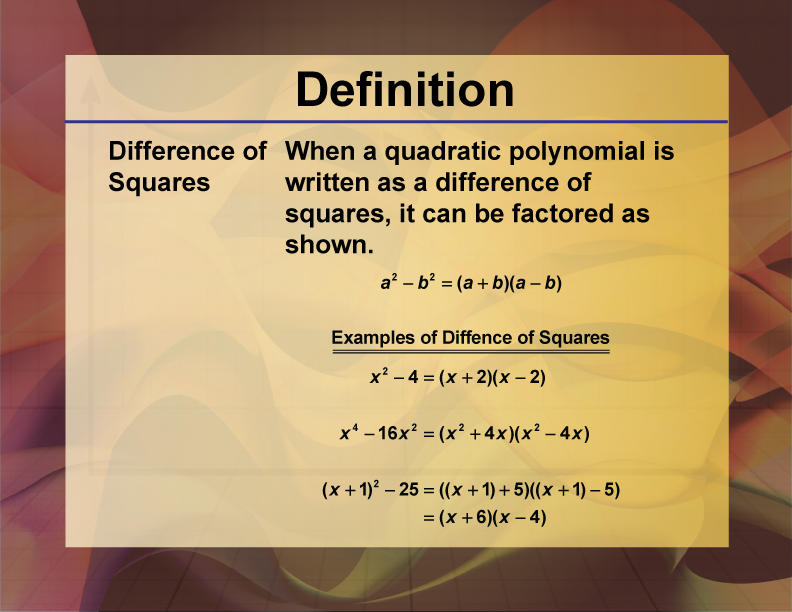
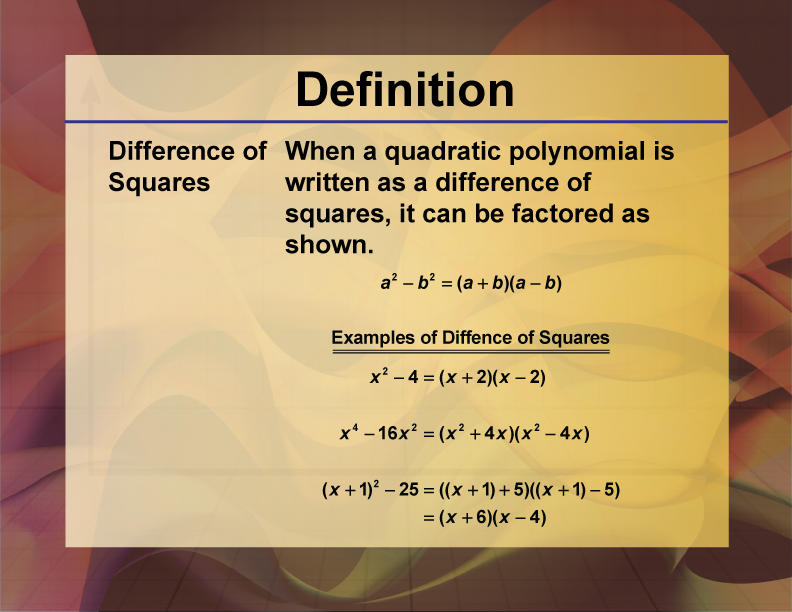
The difference of squares is a polynomial expression of the form a² - b², which can be factored as (a + b)(a - b).
Description
The difference of squares is a fundamental concept in algebra, particularly in the study of polynomials. This special factoring technique is widely used to simplify expressions and solve equations. Understanding the difference of squares pattern allows students to quickly recognize and factor certain quadratic expressions, making complex algebraic manipulations more manageable.
The importance of the difference of squares extends beyond basic algebra. It is a crucial tool in advanced mathematics, including calculus and number theory. In practical applications, this concept is used in various fields such as physics, engineering, and computer science, where it helps in simplifying calculations and modeling real-world phenomena. Mastering the difference of squares enhances problem-solving skills and provides a foundation for understanding more complex polynomial factorizations.
For a complete collection of terms related to polynomials click on this link: Polynomials Collection
| Common Core Standards | CCSS.MATH.CONTENT.HSA.APR.A.1, CCSS.MATH.CONTENT.HSA.APR.B.2, CCSS.MATH.CONTENT.HSA.APR.C.5, CCSS.MATH.CONTENT.HSA.APR.C.4, CCSS.MATH.CONTENT.HSA.APR.B.3, CCSS.MATH.CONTENT.HSF.IF.C.7.C |
|---|---|
| Grade Range | 8 - 12 |
| Curriculum Nodes |
Algebra • Polynomials • Factoring Polynomials |
| Copyright Year | 2021 |
| Keywords | polynomials, monomials, definitions, glossary term, difference of squares |