
Display Title
Definition--Polynomial Concepts--Polynomial Expansion
Display Title
Polynomial Expansion
Topic
Polynomials
Definition
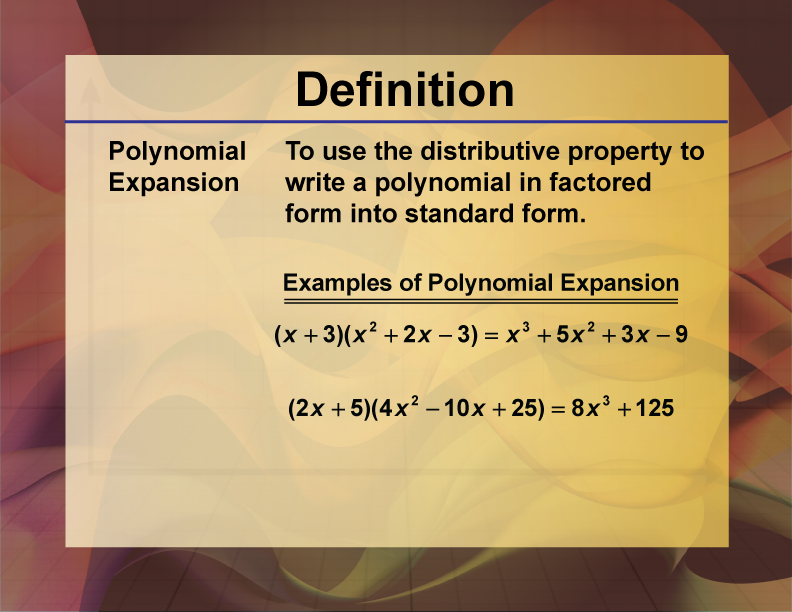
Polynomial expansion is the process of expressing a polynomial as a sum of terms by distributing and combining like terms.
Description
Polynomial expansion is a fundamental operation in algebra that involves expressing a polynomial as a sum of individual terms. This process often requires distributing and combining like terms to simplify the expression. Polynomial expansion is essential for solving equations, simplifying expressions, and performing other algebraic operations.
Understanding polynomial expansion is crucial for mastering algebraic techniques and for progressing to more advanced mathematical topics. It provides a systematic way to break down complex polynomials into simpler components, making it easier to analyze and manipulate them. Polynomial expansion is also a stepping stone to more advanced operations such as polynomial multiplication and division.
In practical applications, polynomial expansion is used in fields such as physics, engineering, and economics to model and solve problems involving polynomial relationships. It helps in understanding the dynamics of systems, predicting trends, and analyzing data. Mastery of polynomial expansion enhances problem-solving skills and provides a foundation for understanding more complex algebraic and calculus concepts.
For a complete collection of terms related to polynomials click on this link: Polynomials Collection
| Common Core Standards | CCSS.MATH.CONTENT.HSA.APR.A.1, CCSS.MATH.CONTENT.HSA.APR.B.2, CCSS.MATH.CONTENT.HSA.APR.C.5, CCSS.MATH.CONTENT.HSA.APR.C.4, CCSS.MATH.CONTENT.HSA.APR.B.3, CCSS.MATH.CONTENT.HSF.IF.C.7.C |
|---|---|
| Grade Range | 8 - 12 |
| Curriculum Nodes |
Algebra • Polynomials • Polynomial Expressions |
| Copyright Year | 2021 |
| Keywords | polynomials, monomials, definitions, glossary term |