Display Title
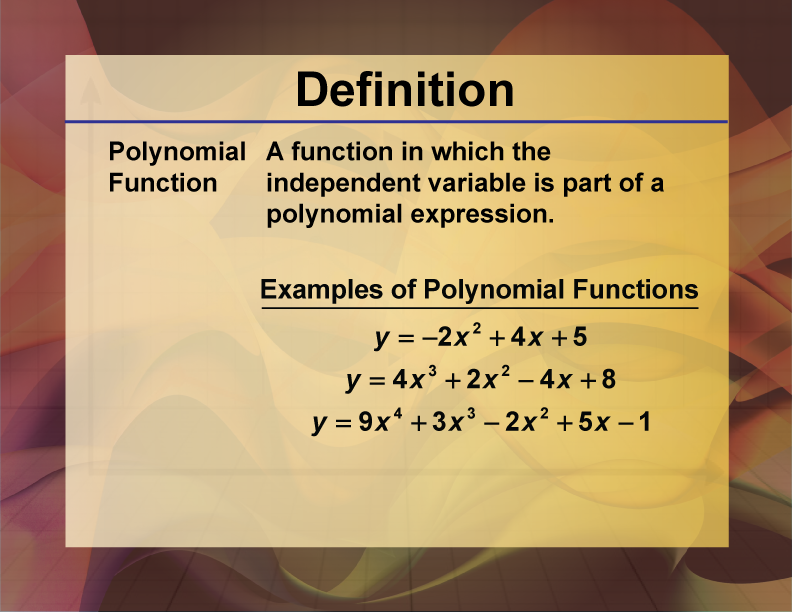
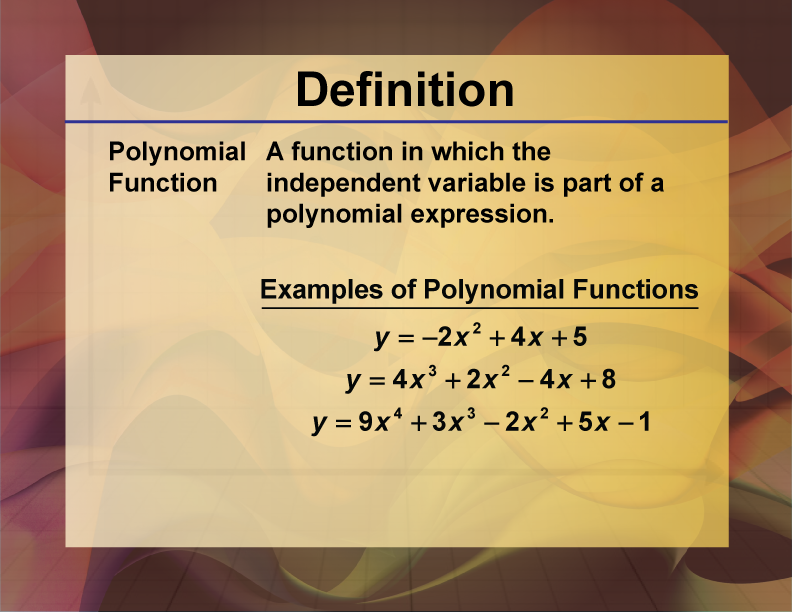
Definition--Polynomial Concepts--Polynomial Function
Display Title
Polynomial Function
Topic
Polynomials
Definition
A polynomial function is a function that is defined by a polynomial expression.
Description
Polynomial functions are fundamental in algebra and calculus, representing relationships between variables that can be expressed as polynomial equations. These functions are characterized by their smooth, continuous curves and predictable behavior. Polynomial functions are used to model a wide range of phenomena in mathematics and the real world.
Understanding polynomial functions is essential for analyzing and interpreting mathematical models. They provide insights into the behavior of systems, including the location of roots, turning points, and end behavior. Polynomial functions are also crucial in calculus, where they are used to study limits, derivatives, and integrals.
In practical applications, polynomial functions are used in fields such as physics, engineering, and economics to model and analyze real-world phenomena. They help in understanding the dynamics of systems, predicting trends, and solving complex problems involving polynomial relationships. Mastery of polynomial functions enhances problem-solving skills and provides a deeper understanding of algebraic and calculus concepts.
For a complete collection of terms related to polynomials click on this link: Polynomials Collection
What Are Polynomials?
Polynomials Are Made Up of Monomials
A monomial is a single expression that is usually the product of a number and one or more variables raised to a positive exponent power. Read the following definition.
A monomial is an example of a polynomial.
Binomials Are Made Up of Two Monomials
If you combine two monomials, you have a binomial, so long as the two monomials cannot be further added or subtracted. Read the following definition.
Notice how each binomial is made up of two monomials. Here’s an example of two monomials that, when combined, result in a monomial, not a binomial.
Trinomials Are Made Up of Three Monomials
If you combine three monomials, you have a trinomial, so long as the monomials cannot be further added or subtracted. Read the following definition.
Notice how each binomial is made up of three monomials.
The Degree of a Polynomial
The term with the highest exponent determines the degree of the polynomial.
This is a polynomial of degree 1:
This is a polynomial of degree 2:
This is a polynomial of degree 3:
| Common Core Standards | CCSS.MATH.CONTENT.HSA.APR.A.1, CCSS.MATH.CONTENT.HSA.APR.B.2, CCSS.MATH.CONTENT.HSA.APR.C.5, CCSS.MATH.CONTENT.HSA.APR.C.4, CCSS.MATH.CONTENT.HSA.APR.B.3, CCSS.MATH.CONTENT.HSF.IF.C.7.C |
|---|---|
| Grade Range | 8 - 12 |
| Curriculum Nodes |
Algebra • Polynomials • Polynomial Functions and Equations |
| Copyright Year | 2013 |
| Keywords | polynomials, monomials, definitions, glossary term |