Display Title
Definition--Polynomial Concepts--Remainder Theorem
Display Title
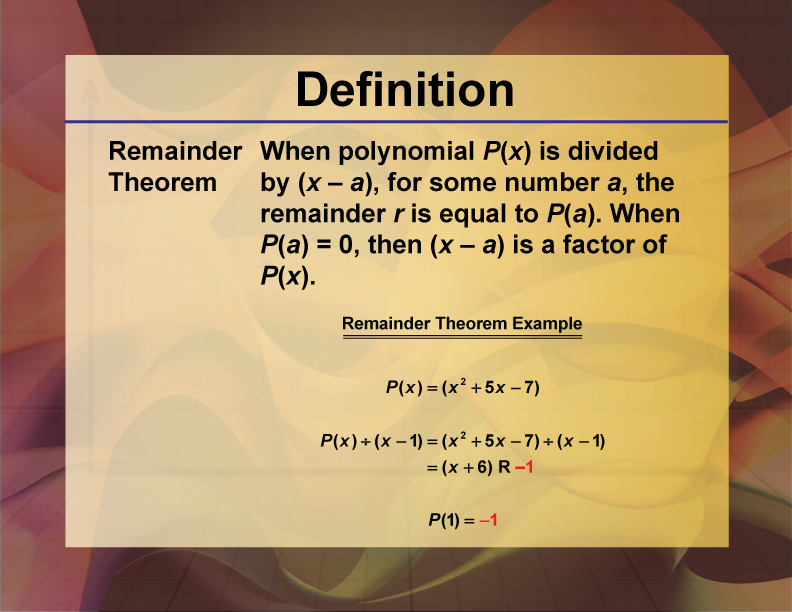
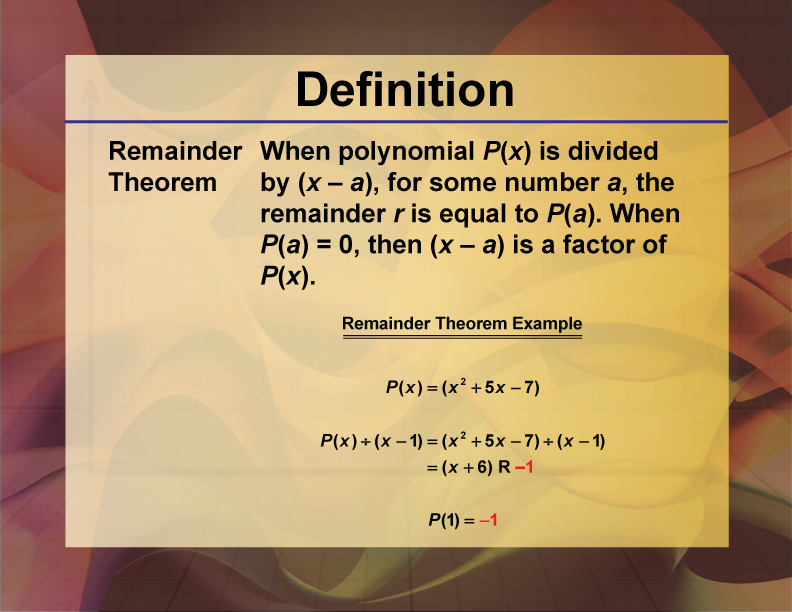
Remainder Theorem
Topic
Polynomials
Definition
The Remainder Theorem states that the remainder of the division of a polynomial by a linear divisor (x - c) is equal to the value of the polynomial evaluated at c.
Description
The Remainder Theorem is a fundamental concept in algebra that provides a method for determining the remainder of the division of a polynomial by a linear divisor (x - c). According to the theorem, the remainder is equal to the value of the polynomial evaluated at c. This theorem is particularly useful for solving polynomial equations and for analyzing polynomial functions.
Understanding and applying the Remainder Theorem is crucial for solving polynomial equations and for analyzing the behavior of polynomial functions. It provides a method for quickly finding the remainder without performing long division, making it easier to factor and simplify polynomials. The Remainder Theorem is also a stepping stone to more advanced algebraic techniques such as synthetic division and polynomial factorization.
In practical applications, the Remainder Theorem is used in fields such as physics, engineering, and economics to model and analyze real-world phenomena. It helps in understanding the dynamics of systems, predicting trends, and solving complex problems involving polynomial relationships. Mastery of the Remainder Theorem enhances problem-solving skills and provides a deeper understanding of algebraic and calculus concepts.
For a complete collection of terms related to polynomials click on this link: Polynomials Collection
| Common Core Standards | CCSS.MATH.CONTENT.HSA.APR.A.1, CCSS.MATH.CONTENT.HSA.APR.B.2, CCSS.MATH.CONTENT.HSA.APR.C.5, CCSS.MATH.CONTENT.HSA.APR.C.4, CCSS.MATH.CONTENT.HSA.APR.B.3, CCSS.MATH.CONTENT.HSF.IF.C.7.C |
|---|---|
| Grade Range | 8 - 12 |
| Curriculum Nodes |
Algebra • Polynomials • Factoring Polynomials |
| Copyright Year | 2021 |
| Keywords | polynomials, monomials, definitions, glossary term, Remainder Theorem |