Display Title
Definition--Prime and Composite Properties--Composite Number
Display Title
Composite Number
Topic
Prime and Composite Numbers
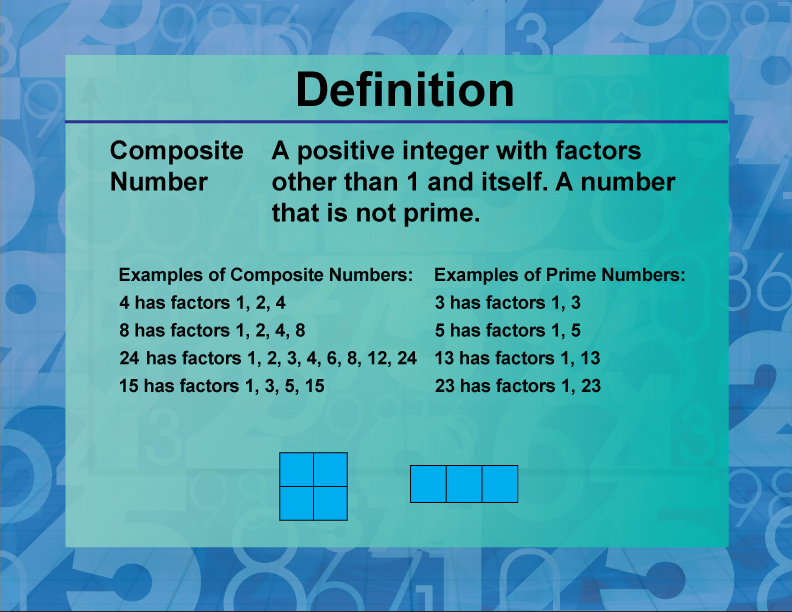
Definition
A composite number is a positive integer that has at least one positive divisor other than one or itself, meaning it can be divided evenly by numbers other than 1 and itself.
Description
Composite numbers play a crucial role in the study of prime and composite numbers, which are fundamental concepts in number theory. Unlike prime numbers, which have exactly two distinct positive divisors (1 and the number itself), composite numbers have more than two positive divisors. This distinction is essential for various mathematical applications, including factorization, cryptography, and the simplification of fractions.
Composite numbers can be broken down into their prime factors, a process known as prime factorization. For example, the number 12 can be expressed as the product of its prime factors: $$12 = 2 \times 2 \times 3$$ Understanding composite numbers and their properties helps in solving problems related to divisibility, greatest common divisors (GCD), and least common multiples (LCM). Additionally, the identification of composite numbers is significant in fields such as computer science, where algorithms often rely on the properties of numbers for encryption and data security.
For a complete collection of terms related to primes and composites click on this link: Prime and Composites Collection.
Brief Review of Composite Numbers
Watch the following video to get an overview of composite numbers.
What Is a Composite Number?
The video was uploaded on 10/11/2022.
You can view the video here.
The video lasts for 2 minutes and 56 seconds.
Video Transcript
When you multiply two numbers, each of them is a factor. Look at this example.
3*6=18
The product, 18, is the result of multiplying two factors, 3 and 6. The number 18 has other factors, which are shown here:
1, 2, 3, 6, 9, 18
The number 18 is divisible by each of these factors, with no remainder.
The number of factors determines the type of number.
A composite number has at least three factors.
Let's look at an example.
The number 9 has these factors:
1, 3, 9
It is a composite number because it has three factors.
Here's another example:
The number 24 has these factors:
1, 2, 3, 4, 6, 8, 12, 24
Although 24 has more factors than 9, both are composite numbers.
Notice that 9 has an odd number of factors, while 24 has an even number of factors.
Why is this?
The number 9 is a square number.
It can be written as 3-squared.
Here are two more square numbers and their factors:
25 is 5-squared.
Its factors are 1, 5, 25
36 is 6-squared.
Its factors are 1, 2, 3, 6, 12, 18, 36
In both cases each of the square numbers has an odd number of factors.
In general, all square numbers have an odd number of factors.
Here's why.
When you list the factors of a composite number with an even number of factors, for example, the number 12, you can create factor pairs.
Here are the factors of 12:
1, 2, 3, 4, 6, 12
Pairs of factors, have a product of 12.
1 times 12 equals 12
2 times 6 equals 12
3 times 4 equals 12
Now do the same factor pairs with the factors of a square number.
Let's look at 36:
1, 2, 3, 6, 12, 18, 36
1 times 36 is 36
2 times 18 is 36
3 times 12 is 36
6 times 6 is 36
In the last case, 6 is a factor twice, but it only appears once on the list of factors.
This is why square numbers have an odd number of factors.
Brief Review of Factors
When you multiply two numbers, each of them is a factor.
3 • 4 = 12
In this example, both 3 and 4 are factors. In fact, you can write any number as the product of its factors.
12 = 1 • 12
12 = 2 • 6
12 = 3 • 4
Notice the three factor pairs for 12. Because of the Commutative Property, we also have these factor pairs:
12 = 12 • 1
12 = 6 • 2
12 = 4 • 3
Every number has factors, which can be listed from least to greatest. Here are five numbers and their associated factors:
6: {1, 2, 3, 6}
8: {1, 2, 4, 8}
12: {1, 2, 3, 4, 6, 12}
15: {1, 3, 5, 15)
17: {1, 17}
Notice that, when written from least to greatest, every number includes 1 and the number itself.
What Are Prime Numbers?
Some numbers have only two factors, 1 and the number itself. These are called prime numbers. This table lists four prime numbers and their factors.
Number | Factor | Factor 2 |
2 |
1 |
2 |
3
|
1
|
3
|
5
|
1
|
5
|
7
|
1
|
7
|
Notice that in each case the factors are 1 and the number itself. The numbers 2, 3, 5, and 7 are prime numbers. There are many other prime numbers.
to learn more about prime numbers, watch the following video.
What Is a Prime Number?
The video was uploaded on 10/11/2022.
You can view the video here.
The video lasts for 2 minutes and 12 seconds.
Video Transcript
When you multiply two numbers, each of them is a factor. Look at this example. 3*4=12
The product, 12, is the result of multiplying two factors, 3 and 4. The number 12 has other factors, which are shown here: 1, 2, 3, 4, 6, 12
Each of these numbers divides into 12, with no remainder. Notice that 1 and the number itself are also factors. All numbers are divisible by 1, and every number is divisible by itself. There is a special class of numbers that only have two factors, 1 and the number itself.
Let's look at an example. The number 17 is divisible by 1. It's not divisible by 2. It's not divisible by 3. It's not divisible by 4. It's not divisible by 5. It's not divisible by 6. It's not divisible by 7. It's not divisible by 8.
Any whole number greater than 8 up to 17 does not divide evenly into it. Finally, 17 is divisible by 17. So the factors of 17 are 1 and 17. This is an example of a prime number. What this class of numbers has in common is that these numbers only have two factors, 1 and the number itself. Here are the first ten prime numbers:
2, 3, 5 7, 11, 13, 17 19, 23, 29
Notice that all of them, except for 2, are odd numbers. 2 is the only even prime number. Also, notice that 1 isn't prime. It does seem to follow the rule that it's only divisible by 1 and itself. The difference is that 1 only has one factor. A prime always has two factors. How can you test if any number is prime? If it is only divisible by 1 and itself, then it is prime.
What Are Composite Numbers?
Some numbers have more than two factors. These are called composite numbers. This table lists some composite numbers and their factors.
Number | Factor 1 | Factor 2 | Factor 3 | Factor 4 | Factor 5 | Factor 6 |
4
| 1 |
4
|
2
| — | — | — |
6
| 1 |
6
|
2
|
3
| — | — |
8
| 1 |
8
|
2
|
4
| — | — |
10
| 1 |
10
|
2
|
5
| — | — |
12
| 1 |
12
|
2
|
6
|
3
|
4
|
If you arrange the factors of a composite number from least to greatest, they can form factor pairs, as shown below.
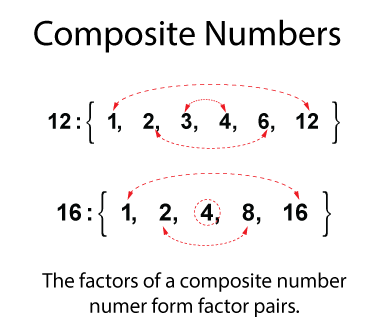
Notice the factor pairs for 12. The arrows indicate which two numbers, when multi8plied, equal 12. There are six factors, resulting in three factor pairs.
Notice the factor pairs for 16. Here are there are five factors. In this case one of the factors, 4, is multiplied by itself to get a product of 16.
As you can see, each of the numbers in the table has more than two factors, and this is what makes them composite numbers.
Factors of Square Numbers
In the previous examples, there was one example of a composite number with an odd number of factors: 4. In addition to being a composite number, 4 is also what's termed a square number. This table shows the factors of the first four square numbers.
Number
| Factor 1
| Factor 2 | Factor 3 | Factor 4 | Factor 5 |
4 |
1 |
4 |
2 |
— |
— |
9 |
1 |
9 |
3 |
— |
— |
16 |
1 |
16 |
2 |
8 |
4 |
25 |
1 |
25 |
5 |
— |
— |
Notice that each of the square numbers has an odd number of factors. Why is this? Square numbers have a factor that is multiplied by itself. For example, 16 has a factor of 4 because 16 = 42.
Prime Factors
You've seen the difference between prime and composite numbers. Prime numbers have only two factors, 1 and the number itself. Composite number have more than two factors. When a composite number has an even number of factors, then there are factor pairs whose product equals the original number. In the case of square numbers, these are composite numbers with an odd number of factors.
Nevertheless, composite numbers can be written as the product of prime numbers. Look at these examples.
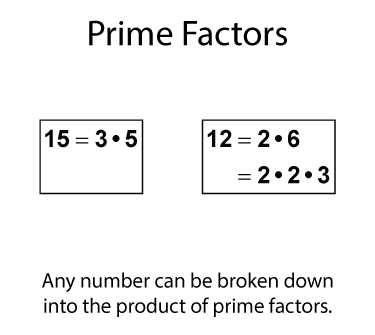
The first composite number, 16, is the product of two prime numbers, 3 and 5. The other composite number, 12, is shown as the product of multiples of 2 and 3, both of which are prime numbers.
Now that you know that any number can be written as the product of primes, we can write the product using exponents. Take a look at these examples.
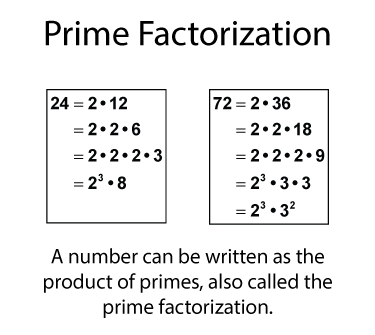
In the first example, 24 is shown as the product of multiples of 2 and 3. This string of factors can be written as 2-cubed times 8. That is known as the prime factorization.
The second example shows how 72 can be written as the product of 2-cubed and 3-squared. With a prime factorization, the number is broken down to its essential prime components.
Even and Odd Numbers
There are two formulas that can be used to generate all even and odd numbers. See the formulas below.
Using this formula, you can generate any even and odd numbers. For example, here are 10 even numbers, using values n = 1 to n = 10:
Even: 2, 4, 6, 8, 10, 12, 14, 16, 18, 20
Here are 10 odd odd numbers, using values n = 0 to n = 9:
Odd: 1, 3, 5, 7, 9, 11, 13, 15, 17, 19
Notice that the formula for an even number always has a factor of 2. While 2 is a prime number, it is the only even number that is prime. So, we can conclude the following:
All prime numbers greater than 2 are odd.
The formula for odd numbers will, in some cases, generate a prime number. However,
Not all odd numbers are prime.
Finally, we can conclude the following:
All composite numbers are even or odd.
Sum of Odd Numbers
Let's revisit the formula for an odd number. Below you'll see two odd number formulas, each representing a different odd number. Notice how the terms combine into a term with 2 as a factor. This is the same as the formula for an even number.
We can conclude the following:
The sum of two odd numbers is an even number.
We can also conclude the following:
The sum of two odd numbers is a composite number.
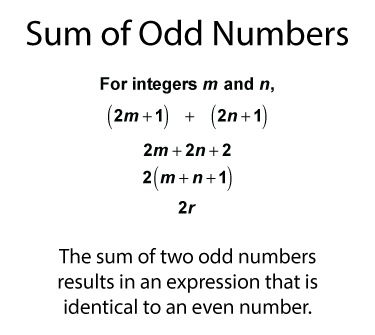
Finally, let's look at the case of the sum of consecutive odd numbers. Take a look at these sums, which are sums of consecutive odd numbers:
1 + 3 = 4
1 + 3 + 5 = 9
1 + 3 + 5 + 7 = 16
1 + 3 + 5 + 7 + 9 = 25
What do you notice about the sums? Here are the same sums rewritten:
1 + 3 = 4 = 22
1 + 3 + 5 = 9 = 32
1 + 3 + 5 + 7 = 16 = 42
1 + 3 + 5 + 7 + 9 = 25 = 52
Each of the sums is a square number. So, we can conclude the following:
The sum of consecutive odd numbers is a square number.
From what you've seen previously, square numbers are also composite numbers.
| Common Core Standards | CCSS.MATH.CONTENT.4.OA.B.4 |
|---|---|
| Grade Range | 4 - 6 |
| Curriculum Nodes |
Arithmetic • Numbers and Patterns • Prime and Composite Numbers |
| Copyright Year | 2013 |
| Keywords | composite numbers, prime numbers, factors, definitions, glossary term |
