Display Title
Definition--Prime and Composite Properties--The Fundamental Theorem of Arithmetic
Display Title
Fundamental Theorem of Arithmetic

Topic
Prime and Composite Numbers
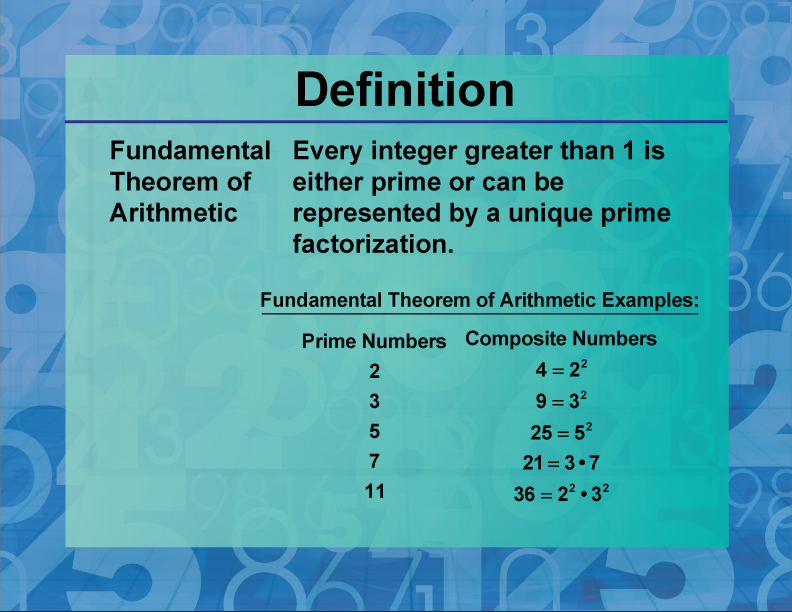
Definition
The Fundamental Theorem of Arithmetic states that every integer greater than 1 can be uniquely represented as a product of prime numbers, up to the order of the factors.
Description
The Fundamental Theorem of Arithmetic is a cornerstone in number theory, asserting that every integer greater than 1 is either a prime number itself or can be decomposed uniquely into a product of prime numbers. This theorem underscores the importance of prime numbers as the basic building blocks of all integers. For example, the number 30 can be uniquely factored into primes as 30 = 2 • 3 • 5, and no other combination of prime numbers will yield 30.
Prime numbers are defined as natural numbers greater than 1 that have no positive divisors other than 1 and themselves. Composite numbers, on the other hand, have more than two positive divisors. The Fundamental Theorem of Arithmetic guarantees that the factorization of any composite number into prime numbers is unique. This uniqueness is crucial for various applications in mathematics, including simplifying fractions, finding greatest common divisors (GCD), and solving problems related to divisibility and prime numbers.
The theorem also has profound implications in fields such as cryptography, where the security of many encryption algorithms relies on the difficulty of factoring large composite numbers into their prime components. Understanding the Fundamental Theorem of Arithmetic is essential for grasping more advanced concepts in number theory and its applications in modern technology.
For a complete collection of terms related to primes and composites click on this link: Prime and Composites Collection.
| Common Core Standards | CCSS.MATH.CONTENT.4.OA.B.4 |
|---|---|
| Grade Range | 4 - 6 |
| Curriculum Nodes |
Arithmetic • Numbers and Patterns • Prime and Composite Numbers |
| Copyright Year | 2021 |
| Keywords | composite numbers, prime numbers, factors, definitions, glossary term |