Display Title
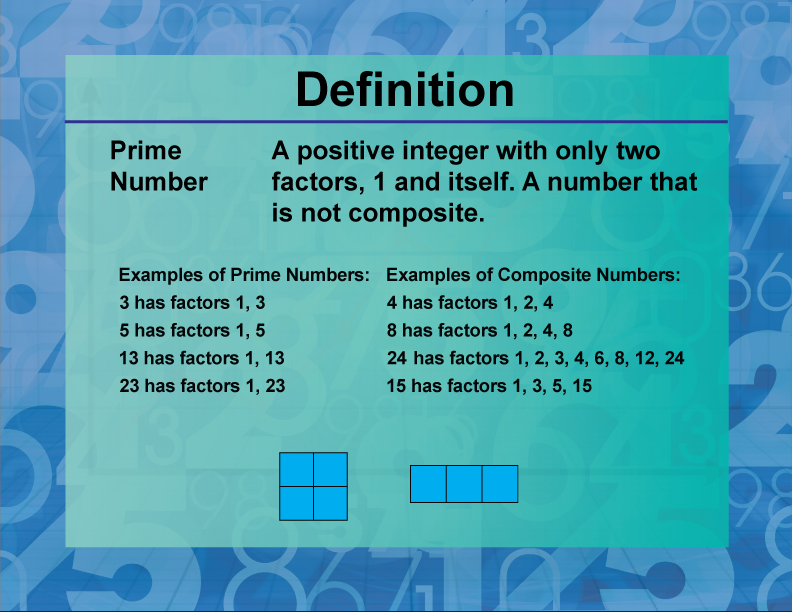
Definition--Prime and Composite Properties--Prime Number
Display Title
Prime Number

Topic
Prime and Composite Numbers
Definition
A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself.
Description
Prime numbers play a crucial role in number theory due to their fundamental properties. They serve as the building blocks of natural numbers because any natural number greater than 1 can be factored uniquely into prime numbers, a concept known as the Fundamental Theorem of Arithmetic. This unique factorization property makes prime numbers essential in various fields such as cryptography, computer science, and pure mathematics.
Prime numbers are used in algorithms for encrypting data, ensuring secure communication over the internet. For example, the RSA encryption algorithm relies on the difficulty of factoring large numbers into their prime components, making it a cornerstone of modern digital security. Additionally, prime numbers are studied extensively in pure mathematics for their intrinsic properties and relationships with other numbers. The distribution of prime numbers among the natural numbers is a subject of deep research, leading to significant mathematical discoveries and conjectures, such as the Riemann Hypothesis.
Understanding prime numbers is not only fundamental for mathematical education but also for practical applications in technology and science. Their simplicity and complexity continue to intrigue mathematicians and scientists, driving ongoing research and exploration in the field.
For a complete collection of terms related to primes and composites click on this link: Prime and Composites Collection.
Prime Numbers
Watch this video to learn abut prime numbers. (The transcript is also available.)
Video Transcript
When you multiply two numbers, each of them is a factor. Look at this example.
3*4=12
The product, 12, is the result of multiplying two factors, 3 and 4.
The number 12 has other factors, which are shown here:
1, 2, 3, 4, 6, 12
Each of these numbers divides into 12, with no remainder.
Notice that 1 and the number itself are also factors.
All numbers are divisible by 1, and every number is divisible by itself.
There is a special class of numbers that only have two factors, 1 and the number itself.
Let's look at an example.
The number 17 is divisible by 1.
t's not divisible by 2.
I
It's not divisible by 3.
It's not divisible by 4.
It's not divisible by 5.
It's not divisible by 6.
It's not divisible by 7.
It's not divisible by 8.
Any whole number greater than 8 up to 17 does not divide evenly into it.
Finally, 17 is divisible by 17.
So the factors of 17 are 1 and 17.
This is an example of a prime number.
What this class of numbers has in common is that these numbers only have two factors, 1 and the number itself.
Here are the first ten prime numbers:
2, 3, 5 7, 11, 13, 17 19, 23, 29
Notice that all of them, except for 2, are odd numbers.
2 is the only even prime number.
Also, notice that 1 isn't prime.
It does seem to follow the rule that it's only divisible by 1 and itself.
The difference is that 1 only has one factor.
A prime always has two factors.
How can you test if any number is prime?
If it is only divisible by 1 and itself, then it is prime.
Brief Review of Factors
When you multiply two numbers, each of them is a factor.
In this example, both 3 and 4 are factors. In fact, you can write any number as the product of its factors.
What Are Prime Numbers?
Some numbers have only two factors, 1 and the number itself. These are called prime numbers. This table lists numbers and their factors.
Notice that in each case the factors are 1 and the number itself. The numbers 2, 3, 5, and 7 are prime numbers. There are many other prime numbers.
What Are Composite Numbers?
Some numbers have more than two factors. These are called composite numbers. This table lists some composite numbers and their factors.
As you can see, each of the numbers in the table has more than two factors, and this is what makes them composite numbers.
Factors of Square Numbers
In the previous table, did you notice there was one example of a composite number with an odd number of factors: 4. Four happens to be a square number. This table shows the factors of the first four square numbers.
Notice that each of the square numbers has an odd number of factors. Why is this? Square numbers have a factor that is multiplied by itself. For example, 16 has a factor of 4 because 16 = 42.
| Common Core Standards | CCSS.MATH.CONTENT.4.OA.B.4 |
|---|---|
| Grade Range | 4 - 6 |
| Curriculum Nodes |
Arithmetic • Numbers and Patterns • Prime and Composite Numbers |
| Copyright Year | 2013 |
| Keywords | composite numbers, prime numbers, factors, definitions, glossary term |