Display Title
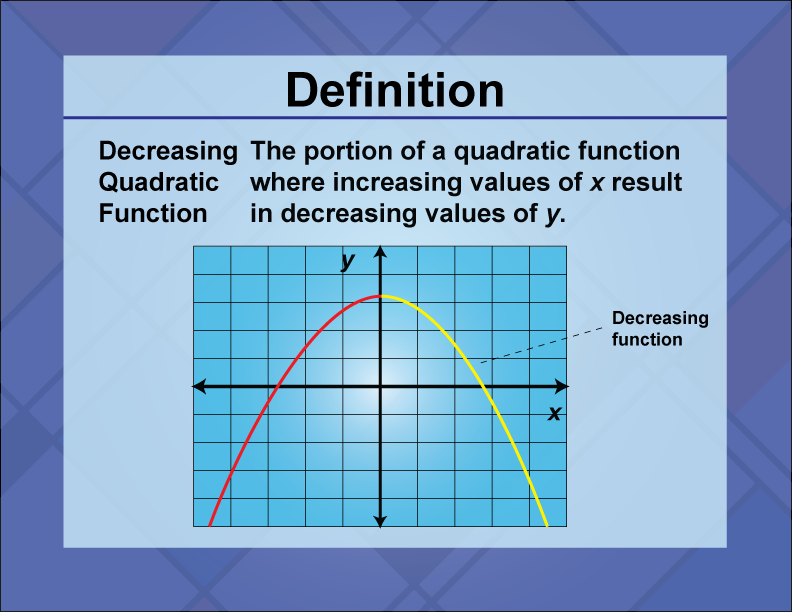
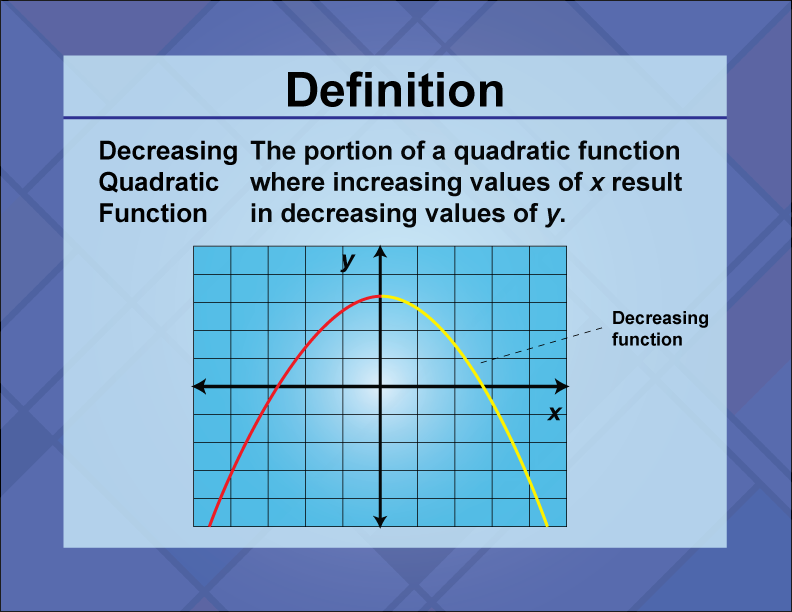
Definition--Quadratics Concepts--Decreasing Quadratic Function
Display Title
Decreasing Quadratic Function
Topic
Quadratics Concepts
Definition
A decreasing quadratic function is a parabola that opens downward, indicating that its vertex is a maximum point.
Description
A decreasing quadratic function is characterized by a leading coefficient that is negative, resulting in a parabola that opens downward. This means that as the input values increase, the output values decrease after reaching a maximum at the vertex. The standard form of such a function is
f(x) = −ax2 + bx + c
with a > 0. Decreasing quadratic functions are used in real-world applications to model scenarios where a quantity increases to a peak and then decreases, such as the trajectory of a thrown object or the profit-maximizing level of production in economics. In math education, understanding decreasing quadratic functions is important for analyzing function behavior, graphing parabolas, and solving optimization problems. Students learn to identify the vertex as the maximum point and use it to determine the range of the function.
An example of a decreasing quadratic function is
f(x) = −2x2 + 4x + 1
where the vertex is the maximum point of the parabola.
For a complete collection of terms related to Quadratic Expressions, Functions, and Equations click on this link: Quadratics Collection
| Common Core Standards | CCSS.MATH.CONTENT.HSN.CN.C.7, CCSS.MATH.CONTENT.HSA.SSE.B.3.B, CCSS.MATH.CONTENT.HSA.REI.B.4.A, CCSS.MATH.CONTENT.HSF.IF.C.8.A |
|---|---|
| Grade Range | 6 - 10 |
| Curriculum Nodes |
Algebra • Quadratic Functions and Equations • Quadratic Equations and Functions |
| Copyright Year | 2021 |
| Keywords | quadratic functions, quadratic equations, quadratic formula, definitions, glossary terms |